Solar recycling or: How to keep your star from dying

Question

I was in the shower the other day thinking about the sun going Nova, you know...like you do, and a thought occurred to me:

Could you turn the sun into a stable, mostly closed system?

Some details of the universe in question:

• The actor in this case is a type II civilization
• They have colonized 5 planets in their 12 planet system
• They have access to unobtanium for the purpose of building any machinery that needs to interact with their star or be used in the process.
• The unobtanium is not adversely impacted by the heat of the star or the cold of interplanetary space. For the sake of this question it is also perfectly sealed meaning there is no loss of whatever in your calculations.

The goal:

• Create a system that allows you to prevent a star (identical to our sun) from going nova, or burning out or at least drastically elongates the lifespan of the star (at least 20% more starry life)
• Requires the least possible amount of external stellar fusion resources from being space-trucked in to feed the system.

Answers must:

• Identify which solar products must be removed from the star
  • How would you extract it? (keep the unobtanium in mind)
  • In what quantities?
  • How much energy would it require? How does this energy compare to overall solar energy captured?
• Identify which elements/compounds must be added to the star
  • In what quantities?
  • How much energy it would take to move them from Mars orbit to the sun
  • For the sake of this question ignore resource availability
• Identify what can be recycled from the extracted stellar waste
  • Ideally most if not all of the required elements could be synthesized from solar waste.
  • The remaining waste can be dumped on a Mercury like planet nearby, the less the better.
• Propose machinery/system to execute the process

Show your work

Answer 1

A star's life ends when it can no longer undergo fusion at its core. For massive stars, this often happens when the core is made largely of iron, which can be fused (and is) in small amounts, but only endothermically. The products of nuclear fusion are, at this point, like carbon monoxide: it's not that their presence is toxic to the star, but it prevents the star from getting the fuel it needs - sort of like how CO molecules binding to hemoglobin make it difficult for a human to get the oxygen it needs. They take up space. Therefore, if you could remove those products, hydrogen could take their place at the core, and the death of the star would be offset.

I very much agree with Ender Look on one point: convection is key. It allows you to transport heavy elements from deep within the star to its surface, while mixing hydrogen back into the core. This happens in the outer regions of all stars, but in low-mass stars(say, less than $\sim0.3M_{\odot}$), this convective region reaches all the way to the core. The Sun's convective zone ends in a place called the tachocline, which occurs at about $0.7R_{\odot}$. Below this point, the star is stable against convection. In particular, something called the Schwarzschild criterion is satisfied: $$\frac{3}{64\pi\sigma G}\frac{\kappa LP}{MT^4}<1-\frac{1}{\gamma_{\text{ad}}}$$ where $\kappa$ is the opacity and $\gamma_{\text{ad}}$ is the adiabatic index. We can assume that $\gamma_{\text{ad}}=5/3$. I decided to try to model the tachocline on my own by determining where the following is satisfied: $$\frac{3}{64\pi\sigma G}\frac{\kappa LP}{MT^4}-\left(1-\frac{1}{\gamma_{\text{ad}}}\right)<0$$ I used numerical simulations by John Bahcall (in particular, the model designated (BS2005-AGS,OP)). I calculated the opacity via Kramer's opacity law, including opacity contributions from free-free and bound-free absorption, as well as electron scattering: $$\kappa_{\text{ff}}=3.68\times10^{22}g_{\text{ff}}(1-Z)(1+X)\frac{\rho}{\text{g cm}^{-3}}\left(\frac{T}{K}\right)^{-7/2}\text{ cm}^2\text{ g}^{-1}$$ $$\kappa_{\text{bf}}=4.634\times10^{25}\frac{g_{\text{bf}}}{t}Z(1+X)\frac{\rho}{\text{g cm}^{-3}}\left(\frac{T}{K}\right)^{-7/2}\text{ cm}^2\text{ g}^{-1}$$ $$\kappa_{\text{es}}=0.2(1+X)\text{ cm}^2\text{ g}^{-1}$$ Assuming that $g_{\text{ff}}\approx g_{\text{bf}}\approx1$ and $t\approx10$, I get the following plot of opacity contributions:

I then plotted the difference of the two sides of the inequality:

This places the tachocline at about $0.82R_{\odot}$ - an overestimate, but not by much.

Notice that for the two major components of the opacity, $\kappa\propto\rho T^{-7/2}$. Therefore, if you increase the density or decrease the temperature, $\kappa$ will increase and the convective region will move inwards. This is what happens during something called a dredge-up, affecting stars that are off the main sequence. Say we cut the temperature to $0.75$ times its current value at all points in the outer half of the Sun. Then we find that the convective envelope can extend to about $0.72R_{\odot}$ - progress. Now, the core extends only to $0.25R_{\odot}$, so we would need to dramatically increase the opacity much deeper in the star to further lower the tachocline.

Once we can figure this out, all that remains is to determine how to increase the efficiency $\lambda$ of this artificial dredge-up - that is, how much of the newly-produced heavy elements are cycled to the surface. Ideally, we'd have $\lambda\approx1$, and typical dredge-up efficiencies vary.

The solution I've come up with is almost counterintuitive: Add more heavy elements. Notice that $\kappa_{\text{bf}}$ - the major source of opacity - is proportional to $Z$. This means that by increasing the amount of heavy elements in the outer layers of the star, we can extend the convective zone down towards the core. Given that for the Sun, $Z\simeq0.001$, changing this shouldn't change $X$ and $Y$ by a significant amount. In fact, $Z$ is so small that there's only about one Jupiter mass of heavy elements in the Sun. I decided to adjust $Z$ by various amounts, and looked at how deep the convective zone extended.

It seems that by adding about Jupiter masses worth of heavy elements, we can extend the tachocline to at least $0.6R_{\odot}$, if not deeper. Remember, of course, that my original calculations underestimated tachocline depth, meaning that in reality, this could be enough to bring the convective zone to the edge of the core.

You might notice that around $0.5R_{\odot}$, it seems that my computations produces a convective region extending to the core even at low metallicities. I believe this zone doesn't exist in those low-metallicity models. So why does it show up? Well, notice how $X$ and $Y$ change deep in the Sun:

Inside about $0.2R_{\odot}$ - and, to an extent, inside $0.5R_{\odot}$ - there is non-negligible gradient of both $X$ and $Y$, which translates to a non-negligible gradient of the mean molecular mass, which means our stability criterion should actually be something called the Ledoux criterion, which takes concentration gradients into account. If I accounted for this, I believe this supposed convective region would disappear - except in the high-metallicity models.

My guess is that adding roughly 30 Jupiter masses (likely less, given that we don't need to increase the metallicity in the core - just the region directly outside it) worth of heavy elements would increase the opacity to the extent that the tachocline would reach the core, allowing for mixing and eventually heavy element transport to the surface. The details of how you get all of these heavy elements back out further compounds the problem, but I believe that if you could trigger a burst of fusion, that could lead to an artificial dredge-up, as I discussed before.

Rinse and repeat, periodically - perhaps every few billion years or so, just to be safe. Honestly, 30 Jupiter masses every few billion years isn't too much to ask of a Type II civilization, is it (depending on what resources are in the planetary system, of course)?

Answer 2

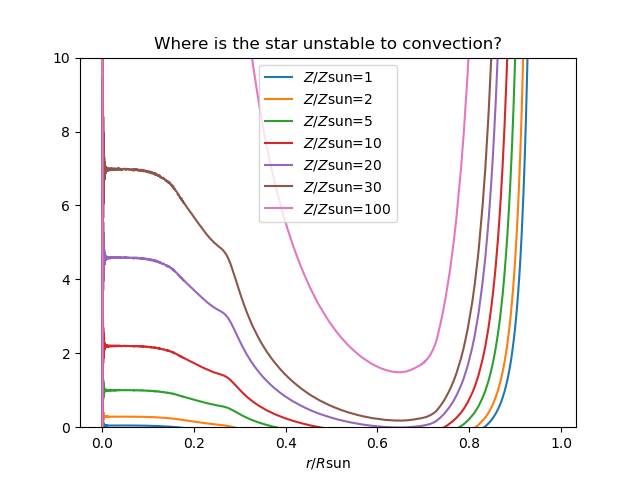
Of course, it's possible, a Kardashev type II civilization will have it quite easy.

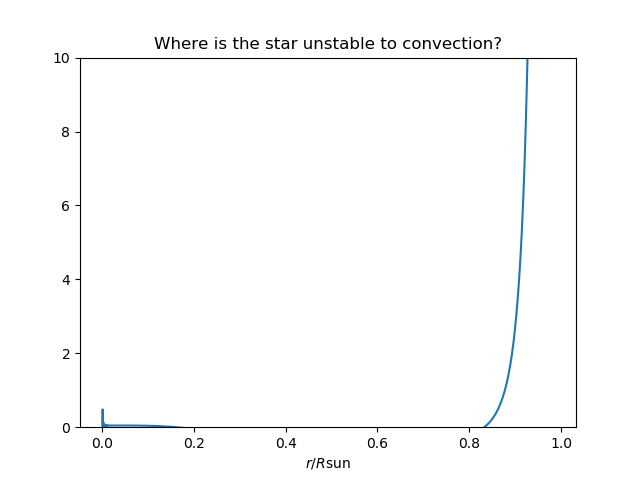
This is a healthy star, like our:

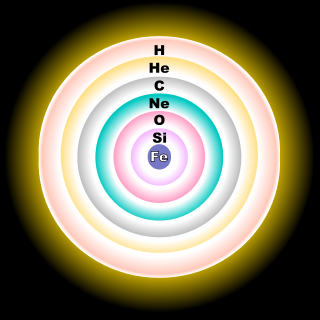
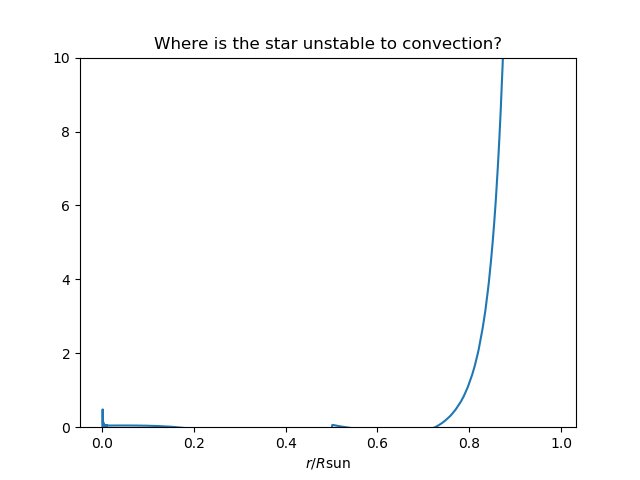
And this is a super healthy star (even when scientists says it's a failed star!):

Our objective is the second image. Now, let me explain everything.

How is a normal star

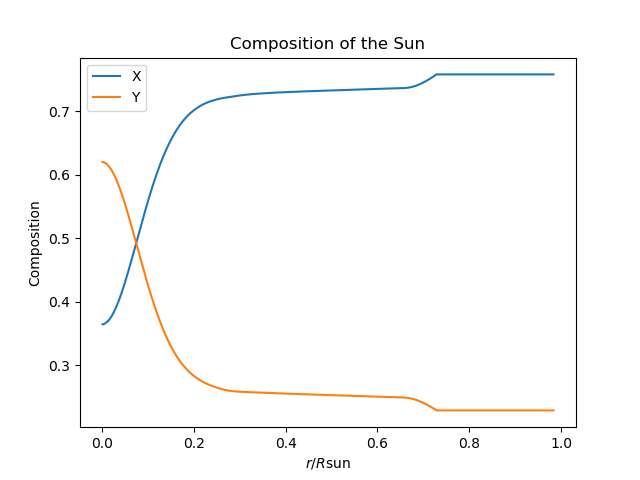
Normal stars, like our star or the star from the first image, have their elements divided into layers. Our sun is like the first image, but with fewer layers (2 main layers: 74% Hydrogen and other from 25% Helium, the 1% is scattered around the photosphere), since it's less massive and younger.

A young star only has hydrogen in its core but, after some time, helium reserves will be build from the hydrogen, and so they will stay in the core (displacing the hydrogen) due to their increased weight. This new helium will replace the main-hydrogen-fusion and create new elements that will perform the same cycle.

Our star is quite young, so the main-fusion is made from hydrogen -even when the core has 60% of helium- due the Sun doesn't have enough temperature to fuse helium yet. The problem is that when helium raise in the core, all the hydrogen (not burned) will be displaced from it to the outer layer, and because the Sun can't fuse helium, gravity will reduce its size, massively increasing the pressure of the core, causing nuclear fusion of helium that will counter-act the new pressure.

So... what is wrong or bad from that? Well, that all the non-used hydrogen is just discarded. That precious fuel won't ever be used by the Sun, instead will accumulate in the outer layer of it. So, how can fix that? That happens because the Sun transfer all its heat in form of radiation, so the solution is:

Convection - The solution

Basically, convection is this:

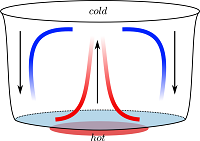
Convection is the heat transfer due to the bulk movement of molecules within fluids such as gases and liquids [...].

An example would be when you boil water in the oven, the water from the bottom becomes hotter and move to the top, forcing colder water to move to the bottom. This is characterized by a mass transfer between the hotter zone to the colder one. And that is great!

If the molecules of the sun are forced to move by convection all the "layers" of elements get broken, that means that heavier elements won't be moved to the core nor lighter elements (hydrogen and helium) will be moved outside it. It will be a homogenous mix! And we need that, so the Sun will be able to fuse all their hydrogen before start fusing heavier ones (like helium). Now the problem is: How to archive convection?

Building a convective star - Mass and Size

From Wikipedia:

Convection is the dominant mode of energy transport when the temperature gradient is steep enough so that a given parcel of gas within the star will continue to rise if it rises slightly via an adiabatic process. In this case, the rising parcel is buoyant and continues to rise if it is warmer than the surrounding gas; if the rising particle is cooler than the surrounding gas, it will fall back to its original height. In regions with a low-temperature gradient and a low enough opacity to allow energy transport via radiation, radiation is the dominant mode of energy transport.

Now, I'll summarize the following paragraph from that link into 4 items.

Basically, the mass of the star determines the type of thermal conduction due to its fusion:

• Well, the first is special. White Dwarf don't fuse, so they don't produce heat, their only transfer its remaining heat using thermal conduction. We will only use this after the star dies, in order to maintain it warm for more time.
• Medium stars (0.3 - 1.5 M_☉), like our Sun, primary fuse hydrogen-to-helium using the proton-proton chain which doesn't produce enough steep temperature gradient (only 4th power). Thus, convection isn't possible and so radiation is used in the core. The outer portion is enough cold to allow convection.
• Massive start (> 1.5 M_☉) has cores with greater temperatures, which allows the CNO cycle to produce hydrogen-to-helium fusion. This cycle has temperature rates of 15th power, enough steep gradient to make convection effective. The outer portion of the star doesn't have steep gradients due being colder, which force them to use radiation.
• Small stars (< 0.3 M_☉) have no radiation zone; the dominant energy transport mechanism throughout the star is convection.

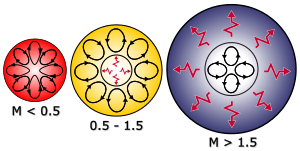
^{Black arrows are convection and red arrows radiation.}

Massive stars are difficult to make and since there are bigger they consume more fuel (even using convection), so smaller stars are the best: we need a red dwarf.

Red Dwarf - The economical star

From Wikipedia:

A red dwarf (or M dwarf) is a small and cool star on the main sequence, of M spectral type. Red dwarfs range in mass from about 0.075 to about 0.50 solar mass and have a surface temperature of less than 4,000 K. Sometimes K-type main-sequence stars, with masses between 0.50-0.8 solar mass, are also included.
[...] Stellar models indicate that red dwarfs less than 0.35 M_☉ are fully convective. Hence the helium produced by the thermonuclear fusion of hydrogen is constantly remixed throughout the star, avoiding helium buildup at the core, thereby prolonging the period of fusion. Red dwarfs, therefore,develop very slowly, maintaining a constant luminosity and spectral type for trillions of years, until their fuel is depleted. Because of the comparatively short age of the universe, no red dwarfs exist at advanced stages of evolution.

^{Emphasis mine.}

Red dwarfs are really small and so they consume a lot less of fuel and remix it constantly avoiding wasted hydrogen. Note that red dwarf produces much less heat and light, so you must (a) bring the planets close to the star (and avoid the possible tidal lock or gravitational squeezing) or (b) produce your own artificial light for those habitable planets.

But, how we make a red dwarf?

Operating a star: Making a Red Dwarf

Make a red dwarf is really simple, you just need to remove mass from it. Well, that is extremely difficult but for a type 2 civilization and with a green light for unobtanium use you may do that in just a blink of eyes.

Once you finish to remove the unnecessary mass, be sure to keep it in a safe place, free of nuclear fusion (or a new star will be made). After that, you should be constantly checking your star, feeding with more hydrogen when it needs, and removing the heavier elements that disturb the convection (a so reduce its luminosity).

After running out with all your fuel reserves, start feeding it with Jupiter, Saturn and the other gas planets from the solar system. After that, I'm sorry but we will have to accept its death... or not.

Maintaining the undead: Blue Dwarf

Blue dwarf From Wikipedia

A blue dwarf is a predicted class of star that develops from a red dwarf after it has exhausted much of its hydrogen fuel supply. Because red dwarfs fuse their hydrogen slowly and are fully convective (allowing their entire hydrogen supply to be fused, instead of merely that in the core), the Universe is currently not old enough for any blue dwarfs to have formed yet, but their future existence is predicted based on theoretical models.

Stars increase in luminosity as they age and a more luminous star needs to radiate energy more quickly to maintain equilibrium. Stars larger than red dwarfs do this by increasing their size and becoming red giants with larger surface areas. Rather than expanding, however, red dwarfs with less than 0.25 solar masses are predicted to increase their radiative rate by increasing their surface temperatures and becoming "bluer". This is because the surface layers of red dwarfs do not become significantly more opaque with increasing temperature.

Your red dwarf will become blue. Also, the increase in heat will allow you to fuse heavier elements like helium, carbon an so on. You may start feeding it with all that heavier elements (but never with iron or heavier, they consume energy instead of produce on fusion). Be careful to not overfeed it and produce a giant star or worse and go supernova.

But finally, it will perish.

The holy corpse: White Dwarf

If your civilization still wants to extend its lifespan you should consider making a new religion for it... anyway white dwarf is dead so they don't fuse.

A white dwarf, also called a degenerate dwarf, is a stellar core remnant composed mostly of electron-degenerate matter. A white dwarf is very dense: its mass is comparable to that of the Sun, while its volume is comparable to that of Earth. A white dwarf's faint luminosity comes from the emission of stored thermal energy; no fusion takes place in a white dwarf wherein mass is converted to energy. ^{Emphasis mine.}

Be careful about its size because:

The material in a white dwarf no longer undergoes fusion reactions, so the star has no source of energy. As a result, it cannot support itself by the heat generated by fusion against gravitational collapse, but is supported only by electron degeneracy pressure, causing it to be extremely dense. The physics of degeneracy yields a maximum mass for a non-rotating white dwarf, the Chandrasekhar limit —approximately 1.44 times of M_☉—beyond which it cannot be supported by electron degeneracy pressure. A carbon-oxygen white dwarf that approaches this mass limit, typically by mass transfer from a companion star, may explode as a type Ia supernova via a process known as carbon detonation [...] ^{Emphasis mine.}

But don't worry, they last a lot of time also (as red dwarf):

A white dwarf is very hot when it forms, but because it has no source of energy, it will gradually radiate its energy and cool. This means that its radiation, which initially has a high color temperature, will lessen and redden with time. Over a very long time, a white dwarf will cool and its material will begin to crystallize, starting with the core. The star's low temperature means it will no longer emit significant heat or light, and it will become a cold black dwarf. [...] the length of time it takes for a white dwarf to reach this state is calculated to be longer than the current age of the universe (approximately 13.8 billion years) [...]

^{Emphasis mine.}

Defying Death: Necromancy!

Now your sacred religion must perform a final ritual, an unholy ritual: necromancy. We can still bring alive the star, but it will not be cheap.

In this catalogue we have three rituals to perform. You may choose one of them... of all if you like, it is possible in the correct order!

First ritual: The Black God (Black Hole)

After the white dwarf becomes a black dwarf hence a truly dead star, increase massively it mass or pressure (preferably the second one) until the gravitational collapse or pressure is enough to make a black hole.

Once you have summoned your black god star feeding it with mass, the accretion disk will produce enough light to simulate a star.

Second ritual: The Army of Hundred of Demons (Tiny Black Holes)

In order to perform this ritual, you must make an extremely small black hole from a little portion of the black dwarf mass. In case you already have a black hole, you are ruined, you might split it extracting a tiny piece of it or use unobtanium to force the [Hawking radiation)(https://en.wikipedia.org/wiki/Hawking_radiation), which is sci-fi.

For being exactly, you need 962.5653 kilograms of a mass black hole to simulate the 384.6 yottawatts of energy produced per second by the Sun (calculated using this) and it will have a radius of 1.429267e-22 centimetres.

Note that this black hole will die after 7.497924e-8 seconds... so you need to feed (or replace) it quickly (if you replace be careful with the super explosion produced before banishing).

From Wikipedia

Hawking radiation reduces the mass and energy of black holes and is therefore also known as black hole evaporation. Because of this, black holes that do not gain mass through other means are expected to shrink and ultimately vanish. Micro black holes are predicted to be larger emitters of radiation than larger black holes and should shrink and dissipate faster

Basically, black holes produce virtual particles and anti-particles (not confuse with antimatter, this is completely different) in its surfaces. These particles are made from nothing but they annihilate themselves very quickly an so any physical law is broken. The interesting thing is that these particles can escape from the black hole and less massive black holes are easier to escape, so, when one of this two particles escape and the other don't, this "escaped particle" becomes "real" and in order to not break any physical law, the black hole is "obligated to pay the price (in mass and energy) of both particles), effectively reducing its mass and emitting light. If you are a physist, please don't criticize me, it's just a very basic explaining.

Last Ritual: The master of tunnels (Quantum tunnelling)

Have you heard about the fascinating Quatum tunnelling? Do you remember the Hawking radiation? Well, that was a type of quantum tunnelling.

It's difficult to explain because it is related to Heisenber uncertainty principle. 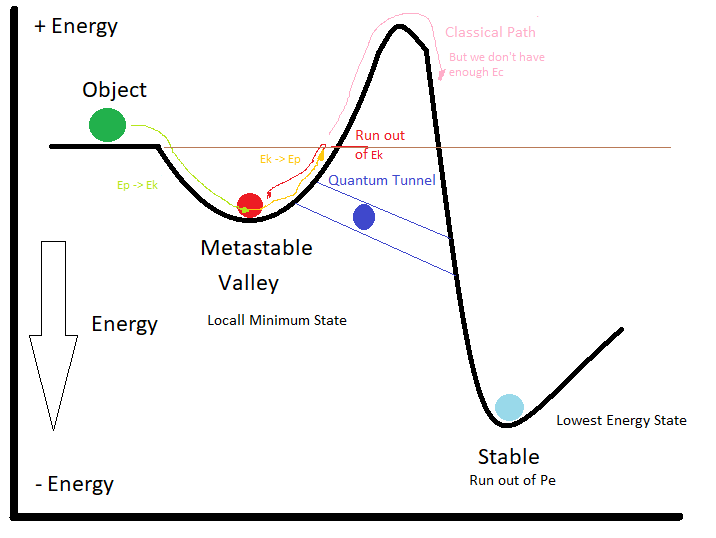Weird, right?
But imagine yourself, if you run at maximum speed to a wall, can you move through it? Well... yes, I mean, NO! Don't try it!
Theoretically speaking you can but in real life you can't. If you want an explaining, in this answer I've already explained it

Using the most refined handwavium techniques with the most exquisite unobtanium, scientists are able to force this quantum effect (for being exactly proton tunnelling), effectively splitting heavier elements into lighter ones as hydrogen. So you are able to recycle wasted elements into precious fuel for your star!

A gift for you!

These are just fancy animated YouTube videos from Kurzgesagt – In a Nutshell that explain some interesting stuff in non-scientific language! And they're last less than 10 minutes!

• Black Holes
• Red Dwarfs
• White and Black Dwarfs.

Answer 3

Basically, stars lose stability as their ratio of heavy and light atoms shifts. Hydrogen is the primary fuel of main sequence stars, but as the heavy non-fuel byproducts of that fussion becomes more concentrated. This increased the inward force of gravity to outward force of fusion ratio which causes it to burn bigger and faster as the volume where fusion is happening increases from added pressure.

So if you want to extend the life of a star, you need to fly in and collect all those heavier elements (iron, aluminum, oxygen, etc) to slow down the fusion. This is especially interesting because your civilization could use those elements to manufacture otherwise impossibly costly structures like the a dyson structure (sphere, cloud, ring, or whatever you prefer) that helped them achieve type 2 to begin with.

The one concern is that while this would extend the star's life, it would also cool it down which would limit the power that a type 2 civilization could gather. For this to be truly sustainable, there would also need to be a good way to put more hydrogen back into the star, and that would be best managed by choosing a star somewhere in a solar nursery where hydrogen can be passively added by the environment. (So, if you do a sphere, it would still need enough holes in it to let new gases in.) A Sun sized star fusses about 300 times the mass of Mount Everest every day. This means you would need a truly massive harvester fleet to keep up with it; so, whatever your unobtainium is made out of, you need to be able to produce it on a huge scale.

For power requirements, you drop your ship from the dyson structure by just decelerating it by a few thousand miles per hour so gravity takes it into the sun (not a significant power expenditure). You mine what you came for, then you use the power of the sun to get back. (solar sails or something like that) To find the power lose, Hydrogen has a mass of 1.00794u and Helium has a mass of 4.002602u, So when 4 hydrogen nuclei fuse to form one helium nucleus, the difference that’s left over is 4*1.00794u - 4.002602u = 0.029158u. This fraction represents 0.029158 / 4.002602 = 0.007285 = 0.7%...

[EDIT]

Since E=MC^2 and v=sqrt(KE/(m*1/2)), we can find that 0.007kg of mass converts to 6.2913e+14 joules which can move the remaining 0.993kg of mass ~35,596 kps. Now I could not find the exact math, but according to this https://www.wired.com/2014/12/empzeal-earthfall/ if an object were to fall from 1au into the sun, it's final journey would cover the last 7% of the distance in the final 13 hours. From this we can assume the opposite which is that if you eject from the sun at ~224kps, gravity will pull you to a stop at 1au. (perfect for landing back in the habitable zone where your dyson structure might be with your fresh building materials) so you don't need to reach the sun's full escape velocity of 618kps to get to an Earth like orbit. That means you only need 0.63% of the star's power output to eject fussed matter at the same rate of production to where you can use it to build up your civilization.

[END EDIT]

...though actual power usage is probably a bit more or less since the cargo to ship mass ratio is undefined. Also, Heavier materials like iron, oxygen, etc are also fused several times; so, if you target them I believe your star's power generated to mass ratio is theoretically better.

On a final note: This would require a round trip of 128 days. The most efficient thing to do would be to have permanent mining stations in the sun, and just spend a day or two loading and unloading your freighters. So if you assume a freight mission is 130 days, this means your total fleet would need to be able to carry ~4.7 quadrillion tons of material per haul. Or in numbers normal people can think about, that is the transport capacity of 8.6 million Triple-E Maersk heavy freight ships.

Answer 4

Assuming your unobtanium is stable even within the core of a star, you can do this:

• You build a fusion power plant made fully from unobtanium, capable to process hydrogen all the way up to iron. The bigger the power plant, the better. Ideally, it would have the same excess-heat power output as your star, you may even want it to produce some power that you don't use otherwise than dissipating it in form of heat. The power output is just the bonus.

• You drop this power plant into the core of the star, and use the generated energy to remove the produced iron from the core. How you do that, it's up to you, but I could envision a gigantic space elevator that moves the iron into a stationary orbit. You'll be building an iron planet out of the star's waste there.

• The effect is, that the excess heat from the power plant increases the star's energy production. The star, however, is a self-regulating power plant itself, so the star will ramp back its own energy production. You are thus replacing hydrogen-to-helium fusion without waste removal with hydrogen-to-iron fusion with waste removal.

• Since you are fusing all the way down to iron, your power plant needs less hydrogen to output the same amount of heat. Thus, your supply lasts longer.

• By removing the waste, you avoid the star's natural up-regulation of power output as it ages and starts fusing heavier elements. The later stages of a star burn much quicker through their fuel, in a large part because the star's power increases. You avoid this ramp up, so the fuel lasts longer.

• Because you are removing the iron, the star shrinks, and will eventually halt its own natural fusion processes. When this happens, it becomes little more than the fusion power plant's fuel reserve and excess heat radiator. Great, now you get to dictate how fast (or slow) your star burns through its fuel!

• And all the time, you are getting insane amounts of nice, easily usable electric power.

Answer 5

I would imagine the effort and power requirements to keep such a star sustainable would far exceed to energy gained from the star. I the dying stages of a star, it may be more energy effective to begin build the society to prepare for the death of the star. This may be strip mining every world of the solar system to build massive space stations to house what may be 100's of billions of citizens. The problem may be then, without a star, how do you power your civilization? They may harvest the unused nuclear fuel from the star upon its death to build more compact fusion reactor that they can extract closer to 100% from. This may sustain the civilization for a few more millennia until the obtain the ability to reach another star system. By that time, they may just move from star to star to stripe it of Hydrogen, Deuterium or such for their own use.