Would pleopod-based swimming locomotion seen in animals like giant isopods work at much larger sizes, or would drag (or something else?) be too much of a problem?
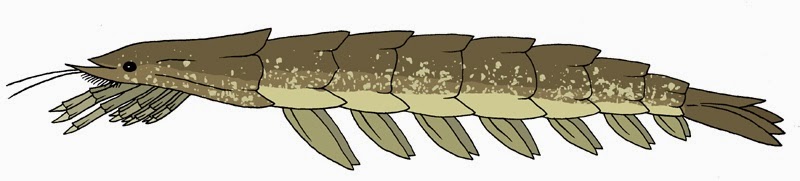
The pleopods, also known as swimmerets, are the paired olive green flaps shown lower right:
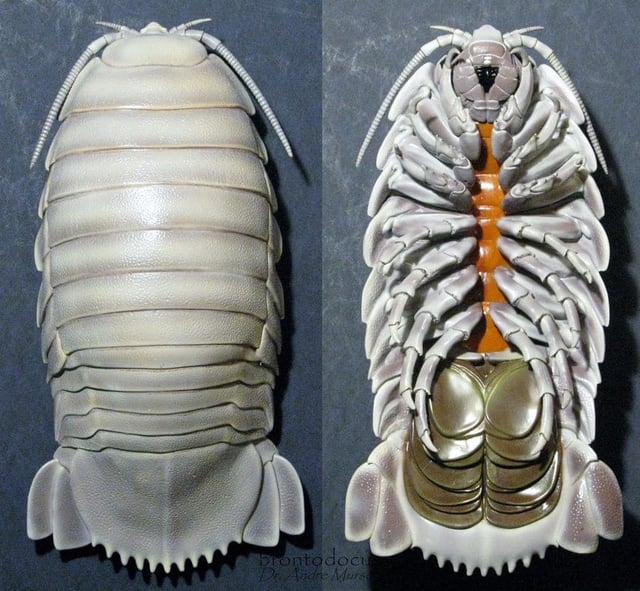
This video shows the pleopods in action from below, timestamp 0:00–0:28, with a side view later.
Imagine a creature, roughly the same proportions of the isopod, with pleopods running most of the length of its ventral side, perhaps 20–25 pairs. Anything from 5 to 30 metres long will do – that's about 12x to 80x the size of a giant isopod. Each pleopod would therefore be between half a metre to five across, and I would guess tens of centimetres thick.
This type of aquatic propulsion is called drag. Drag propulsion is so named because part of the swimming cycle, the recovery phase, has the swimming surface moving against the direction of motion to reset its position. This form of swimming is less efficient than lift-based swimming. Drag can be reduced by changing the profile of the surface in the recovery phase so it is less than the surface in the thrust phase. In the video above it's the clapping-like motion as the pleopods come together and swing forward.
My concern is that such large overlapping surfaces would, during the recovery phase, severly offset the forward motion, or be too small relative to the bulk of the organism to push it forward, or some combination thereof. Or something else I haven't accounted for.
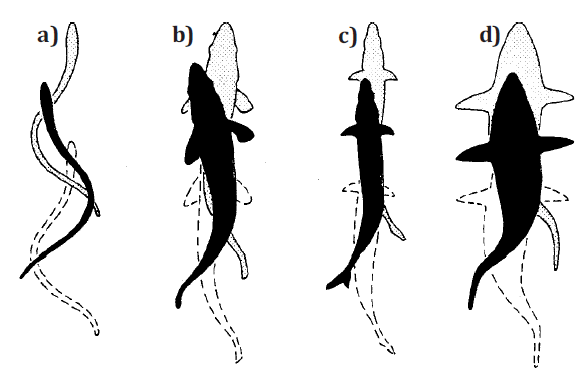
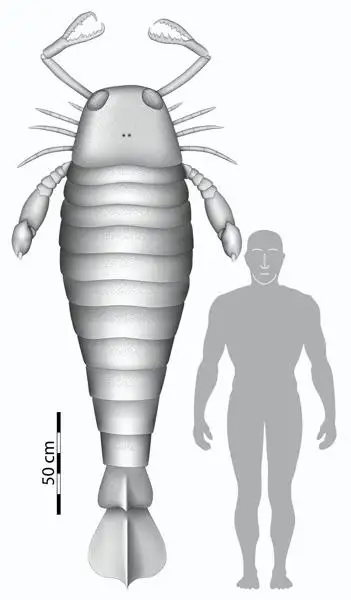
The largest organism known that may have used pleopod-based drag swimming was the Aegirocassis, but the only specimen we have is only a little over two metres long. Contrast that with the largest swimming organisms, all of which swim or swam with lift-based biomechanics. Perhaps there is an upper limit to how effective this form of locomotion can be.
Ignore all the usual square–cube law problems with scaling up arthropods: I'm only interested in the biomechanical swimming action. The organism itself doesn't need to be arthropod-like in any other way.
While I have tagged this science-based and not hard-science I am expecting some number crunching will be necessary to show whether this would scale or not, and sketch the size and/or speed limits would apply.