The planet I'm working with has two different moons, both around the same size (3/4ths that of the main planet). Is it possible for the planet to support the orbit of these moons, especially with these proportions?
-
$\begingroup$ Can you please split this into three questions? The expertise needed to answer the orbital mechanics question, for example, is very different from that needed to answer the biology question. You may want to ask them one at a time, allowing insights from the answers to one to flow into the next. $\endgroup$– userCommented Jan 15, 2016 at 20:07
-
$\begingroup$ Ahhh, thanks! I'll be sure to save the others, then. Can I leave the first one on? $\endgroup$– HawkpeltCommented Jan 15, 2016 at 20:44
-
$\begingroup$ Absolutely! I have reopened this, as it is now much more narrowly scoped and does still appear to be fully on topic for us. $\endgroup$– userCommented Jan 16, 2016 at 8:42
-
1$\begingroup$ With "3/4th of the main planet", do you mean 3/4 of the diameter, or of the volume, or of the mass? $\endgroup$– celtschkCommented Jan 16, 2016 at 9:02
-
$\begingroup$ @celtschk All three, really! Although diameter is quite important in this case... $\endgroup$– HawkpeltCommented Jan 16, 2016 at 19:35
2 Answers
Yes, it's possible
However, you may have to widen your definition of "orbit" somewhat!
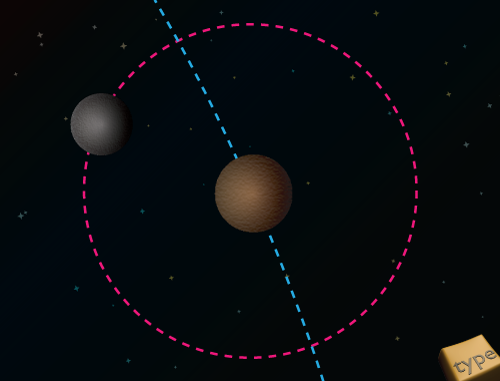
Usually it's relatively safe to say "the moon orbits around the planet", which leads most people to visualize orbits like this:
Fig 1. (As with most diagrams like this, it's not to scale; the moon would be much farther away! But then it'd be very small on the diagram.)
The light blue arc is the planet's orbit around the star. The pink circle is the moon's orbit around the planet.
With Earth's moon, the above diagram would tell the tale (more or less), because our moon is much less massive than the Earth.
However, with a moon that is about half the mass of the planet, the gravitational pull of the moon on the planet becomes significant, and this is what you would see instead:
Fig 2. While the "planet" and "moon" are (still) not to scale, the inner/outer orbit circles are approximately to scale! That means the planet would be heavily "yanked" around by the moon. The planet and moon orbit a common center point known as the "barycenter", and they both then orbit the star (light blue line, as before).
This is known as a double body system, but for our discussion, I'll stick with "planet" (or "primary") and "moons" (or "satellites").
Fig #2 is the most basic way to arrange the orbit. As with any system, the orbits could be elliptical, and/or eccentric (where the ellipses overlap each other, like my 2nd diagram in this question about binary stars, which follow the same basic rules.)
"Size" Assumption
I assumed size = volume, which means the moon has about 42% of the mass of the planet. If you instead take size = mass, so the moon has 75% of the mass, it makes the planet's orbit even wider, but the overall shape is the same.
Adding in the second moon
So far I've only addressed the question of one massive moon. Pretty much everything I've said above applies equally to the 2nd moon. But the orbits get more complicated, as the 3rd moon can start interfering with the orbits of the other two objects (or vice-versa). The orbits get even more complicated if they are eccentric (see above).
For example, the objects can now pull each other into higher orbits or even eject one object from the system, sending it out of its (satellite) orbit and (likely) into its own orbit around the star.
Collisions aren't out of the question, although they're rather unlikely.
How would they form?
One of a few possibilities:
- Leftover swirling "dust" from the creation of the solar system forms the moon.
- Large proto-planet (mass nearly as big as the planet) collides with the planet and knocks a huge mass of debris into orbit that is then pulled together by gravity into a round moon.
- The moon could be a rogue planetoid, captured by the gravity of the planet
Play Simulation Time!
If you'd like to see something like this in action and have a chance to play with the parameters, try UNL's eclipsing binary simulator. It's made for binary star systems, but the principles work just as well for planets and moons. I don't know of any online orbital simulators which can handle more than two bodies (to anyone reading: please leave a comment if you know of one!), however even this two-body simulator could be quite instructive!
Required proportions?
Your question asked about the "required proportions" to make this work. Hopefully this question has already answered that, but I realized I didn't actually address the point directly. As long as you don't have the objects so close that their atmospheres (or surfaces...) collide, the only thing you have to worry about are potentially strong tides (not only liquid ocean tides (if you have oceans), but the same tidal forces could cause seismic tremors if the planetoids are rather close together).
Roche Limit
Someone may happen by and mention the Roche limit, which is the radius within which one of your moons could actually be ripped apart by tidal forces. You would have to design quite an extreme system indeed to have to worry about the Roche limit, assuming your planetoids are all of a typical solid, rocky construction.
So, back to the question of "required proportions": as long as you keep the objects reasonably distant (say, on the order of the Earth-Moon distance of about 385,000 km), with Earth-like masses, you should be OK. I'd suggest you use the above simulator to help you visualize what your parameters might look like.
-
$\begingroup$ Ahhh, thank you so much! One question I have about tidal influence--how strong would these tides be, exactly? I've heard of tides potentially growing up to thousands of feet with two moons, and I'd like to find some way to avoid that... $\endgroup$– HawkpeltCommented Jan 16, 2016 at 20:03
-
$\begingroup$ The lack of online simulators for three bodies is because the three-body problem is unsolved and probably unsolvable in the general case. This means that you need to resort to numeric approximations, which require a lot of processing power to get a reasonable level of accuracy. $\endgroup$– MarkCommented Jan 17, 2016 at 6:21
-
$\begingroup$ @Hawkpelt I can't give you an exact answer, because there are probably infinitely many solutions to keep tides below some arbitrary number, and easily dozens of variables, including (obviously) masses, distance, but also composition, ocean depth, ocean size, atmosphere, precession, and more. I'd suggest you ask another question about tides, and include as many hard details as you can. That might be a good one for the meta sandbox so we can help you nail down some of the variables. $\endgroup$ Commented Jan 17, 2016 at 6:30
-
$\begingroup$ @Mark I know all about the n-body problem. It is harder, yet over fifteen years ago (on desktop machines far less powerful than most smartphones), I worked on algorithms for desktop simulations for n < 32. It's not as computationally expensive as you claim, to get a simulation good enough for an intuitive understanding of how such systems behave (and then some), which is my definition of "reasonable" for this question. :-) $\endgroup$ Commented Jan 17, 2016 at 6:50
-
$\begingroup$ Note that your fig 2 is pretty much what we do have with Pluto and Charon. $\endgroup$– userCommented Feb 18, 2016 at 15:45
The only examples that we have of multi moon systems is where either the multiple moons are very small relative to the planet or where a smaller moon is a much greater distance away than the larger moon. Two large moons would likely perturb each other enough to kick at least one of them out. It is very unlikely that they would chance into any of the very few (if any) stable systems.
Now if one large moon orbited close and the other orbited far enough away to treat the planet and inner moon as a single gravitational source, it might work as well.
In any case, if you have a moon that is 3/4 the size of its planet, you pretty much have a binary planet.