3
$\begingroup$

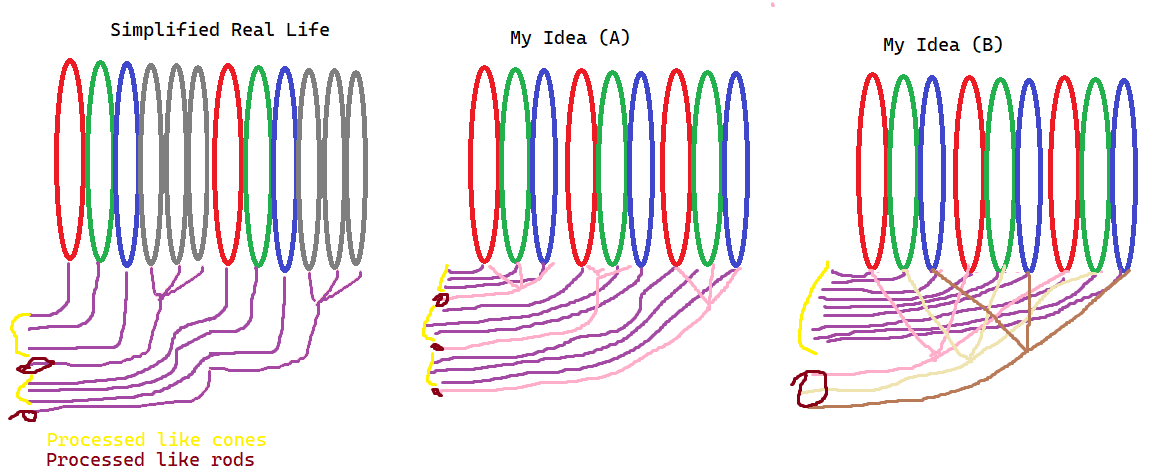
I was reading about how cones and rods work and simplifying a lot how they work I noticed that there are 2 differences:

The first difference is the granularity of connections:

  • Multiple (hundreds to thousands) rods connect per neuron, summing up all their inputs. This ensures the animal can still see even if only a few get excited with photons. The cost of it is worse visual detail.
  • Only a few (even just one in the fovea) cones per neuron. This ensures each neuron collects a smaller visual field space, producing more detail. But each neuron collects fewer photons, making it less sensible to light.

The other difference is that cones have a complement system to compare different colours.

But this arrangement of cones and rods seems to be avoidable if each cone had two neural connections rather than one. That is, one behaves like cones do (few connections per neuron + complement system) and the other behaves like rods do (many connections per neuron).

To make the case more visually understandable:

Example

(The colour of cables is just different to avoid overlaps)

The advantages of such a new layout look obvious to me: since there are no rods + cones, just cones, the entire eye has good colour vision and good dim-light vision, as each photoreceptor does the work of both.

Even more, depending on how you connect the cones (A) or (B) you can get additional benefits. On (B) dim light is still classified by colour, so maybe colour vision could be retained by applying the complement system. On (A) different coloured cones averages, compensating their light collection range, which I think would make dim-light better, at least compared to having a single type of photoreceptor which only has one colour peak.

My question is, Does any of both (A) or (B) layouts work? Does it contain any problem compared to the current wiring which I am missing? Could something like this have evolved in a different world or in Earth if life restarted (i.e.: is it plausible)?

Note: I didn't use the tag hard science because this is kind of speculative, but any citation to papers or detailed answer of why this doesn't/does work would be appreciated.

Improve this question
$\endgroup$
3
  • $\begingroup$ I'd like to note that having access to a greater color spectrum in dim light doesn't create a benifit. Quite the opposite. As luminosity dims all colors tend to smear together. That's one of the advantages to having the monocolor rods - high contrast dim light vision. $\endgroup$
    – JBH
    Commented Sep 18 at 3:10
  • $\begingroup$ @JBH I am not understanding how mono-colour improves high contrast in dim light. Could you explain more, please? $\endgroup$
    – Ender Look
    Commented Sep 19 at 17:55
  • $\begingroup$ The rods, having no need to recognize color, only recognize contrast. That means all the brain power that a cone needs to recognize both multiple colors and multiple shades is focused on just one thing: contrast. That makes it much simpler to see shapes, which is an excellent thing when you're trying to avoid a predator in dim light. Eyeballs evolved to meet needs - and you're ignoring that, judging parts to be irrelevant when they're actually incredibly relevant. I apologize for saying it, but you really should seek to understand eyesight before you seek to change it. $\endgroup$
    – JBH
    Commented Sep 20 at 3:27

2 Answers 2

Reset to default
2
$\begingroup$

The problem is the sensitivity of individual photoreceptor cells. Cone cells have a photosensitive portion that is literally cone-shaped, short and conical, while rod cells have a photosensitive portion that is literally long and cylindrical.

Rod cells are highly sensitive, and can literally be activated by a single photon, while cone cells are far less sensitive, requiring a great many photons to activate them.

When rod cells are exposed to bright daylight, after a short period of activity, their photosensitive pigment becomes 'bleached' and useless (explaining the sometimes painful process of becoming light-adapted after spending time in darkness and moving to a bright place).

Groups of rods sharing a neuron leading to the visual cortex means that in very low-light conditions, activation of any one of the rods in a group provides perception of light over a relatively large area while reducing the necessary size of the visual cortex and optic nerve. With one neuron per rod, higher night-time visual acuity could be achieved, but at the cost of a larger optic nerve and visual cortex, and for a diurnal species, that trade-off is not warranted.

However, cone cells cannot do the same job as the rods. They are evolved to respond to a great many photons and not become bleached and useless, but the adaptations that enable them to do this means that they do not become activated by low numbers of photons at all.

So, it doesn't matter how many cones get ganged together onto one neuron, because the small number of photons that impinge upon the cones at night are insufficient to activate even one of them.

Furthermore, ganging cones together would result in a reduction in the angular resolving power of the eye, as activation of one cone in a gang of cones would be percieved as activation of any one or all of them. For high visual acuity, sensory cells covering a smaller area is more desirable.

Improve this answer
$\endgroup$
3
$\begingroup$

I think you are missing some key aspects of how vision works:

  • rod and cones work differently also because they have different proteins responsible for their answer to absorbed light. It's not only a matter of wiring. If you wire a rod/cone like a cone/rod, it will still sense like a rod/cone. From Wikipedia:

The membranous photoreceptor protein opsin contains a pigment molecule called retinal. In rod cells, these together are called rhodopsin. In cone cells, there are different types of opsins that combine with retinal to form pigments called photopsins. Three different classes of photopsins in the cones react to different ranges of light frequency, a selectivity that allows the visual system to transduce color.

  • Nature, through evolution, is a fond user of Occam's razor. If the current wiring allows an eagle to have its famous eagle eye, I really don't see (pun not intended) what problem you are trying to solve that cannot be solved by the current and simpler implementation.
Improve this answer
$\endgroup$
3
  • $\begingroup$ I know that rods have a different pigment which absorbs a different light range, but is that a problem if it's replaced with 3 different pigments? I think it would be even better, as the range of wavelengths got expanded, and it would prevent some colours looking lighter at dim light but darker in bright light. One problem with the current design is that only the centre of the eye sees colours but is bad at night, while the rest see minimum colours but works better in dim light. My approach allows both things at the same time. $\endgroup$
    – Ender Look
    Commented Sep 17 at 11:57
  • $\begingroup$ @EnderLook: The scotopic vision system is not functional in daylight, when the photopic vision system is working. This is because the opsin in the rods is much more sensitive to light, and at high light level it is depleted much faster than it can be regenerated. Basically, the human eye has two interleaved photosensors: one with very high sensitivity (but monochome and with poor angular resolution) and one with full color and great angular resolution (but less high sensitivity). $\endgroup$
    – AlexP
    Commented Sep 17 at 12:13
  • $\begingroup$ @EnderLook: Numerically, the maximum luminous efficiency of the photopic vision system is 683 lumens per watt at 555 nm, whereas the scotopic vision system has a much higher luminous efficiency of 1700 lumens per watt at a shorter wavelength of 507 nm. $\endgroup$
    – AlexP
    Commented Sep 17 at 12:20

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .