It's probably at least theoretically possible to some extent, but you need a rather large instrument. Or rather, a rather large reflector.
When sound disperses freely in 3 dimensional space it decays roughly quadratically with distance. The easiest way to see why is probably by imagining a large sphere around the sound source, and realising the sound should sound equally loud around all the sphere. As the area of the sphere increases like the square of the radius r, the loudness must thus decay like 1/r^2. This is free space though, and any kind of reflecting materials could complicate this.
Probably, the simplest example is to build a large wall; if we imagine an ideal wall (as I will henceforth do for all surfaces) it's reflection will double the loudness of the sound in the non wall direction, but it would still decay with distance.
If we are inside a very wide building, with a floor and a low ceiling, we could use the same argument as above to see that the perceived loudness should be equal along a circle, and thus decays like 1/r. Though still decaying, this is a huge improvement if the distance is large.
Now, to get our instrument to sound louder when we move away, we need to do better. Enter the Ellipse!
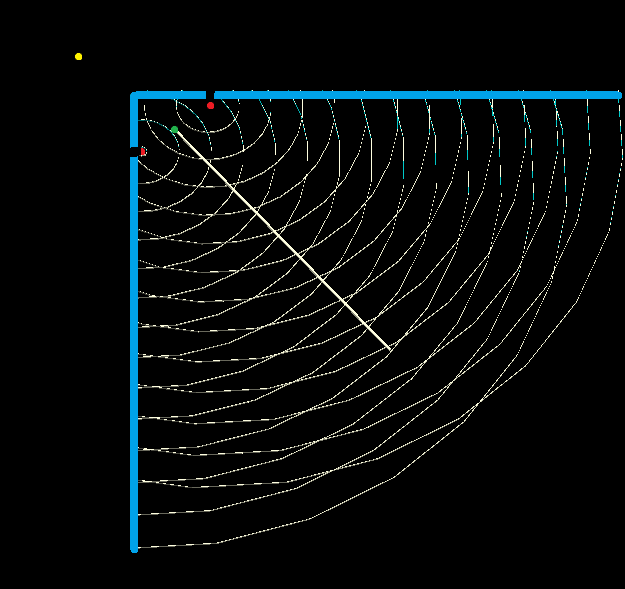
An ellipse, in the mathematical sense, is a precisely defined closed curve with many interesting properties. The one of interest to us is that it has two so-called focal points:
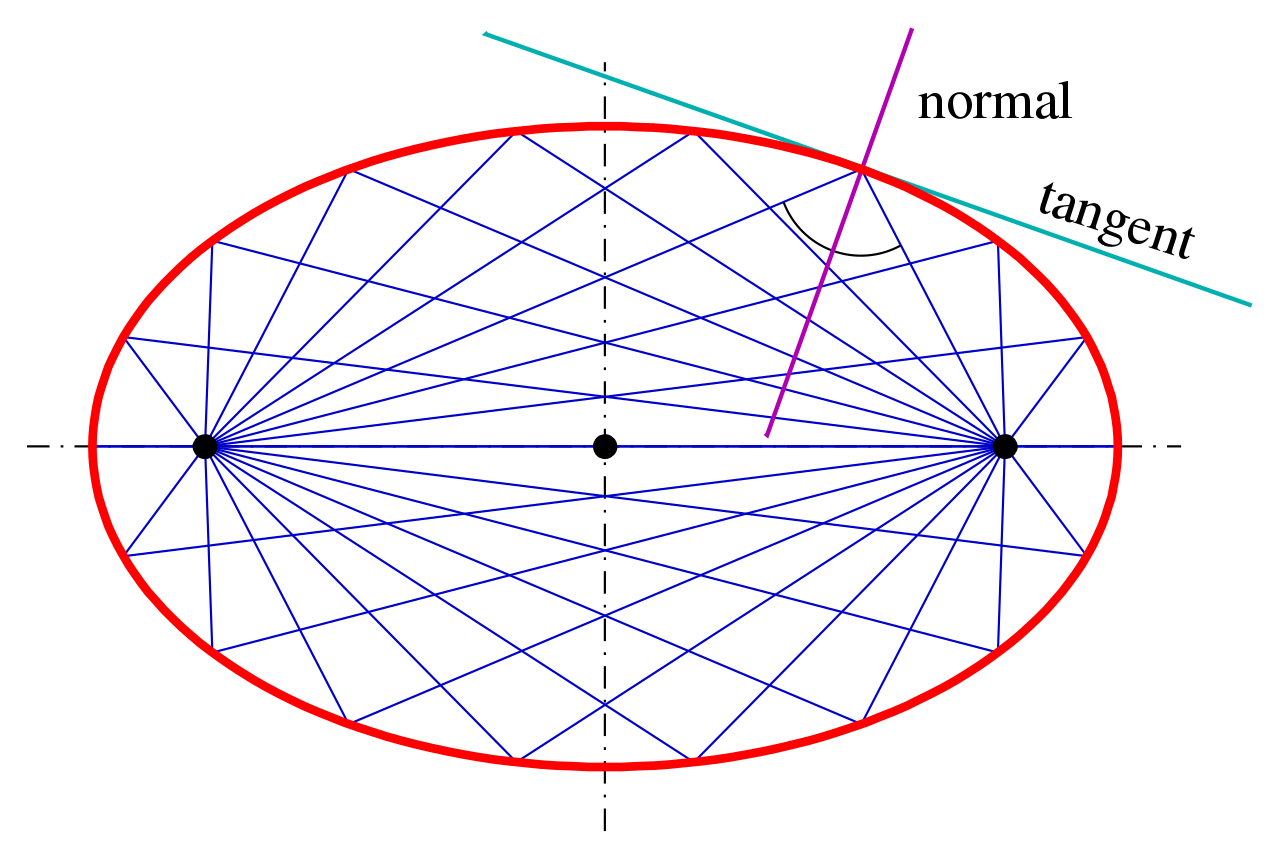 When something, like light or sound, is emitted at one of the focal points it will bounce off the walls and come together to focus at the other. This is true no matter the length of the ellipse.
When something, like light or sound, is emitted at one of the focal points it will bounce off the walls and come together to focus at the other. This is true no matter the length of the ellipse.
This gives us a First answer to your question: In an elliptical room with the instrument at one focal point, a listener could move away and first hear the loudness decay, only to increase again when she arrives at the other focal point.
Now, I presume you want this to work outside. The key observation is that you could take away some segment of the ellipse and still have the sound bouncing off what's left be focused on the other focal point! For listener midway between the points, most of the sound energy will, so to speak, go around her.
If you only care about the loudness in one direction, this is fairly straight forward.
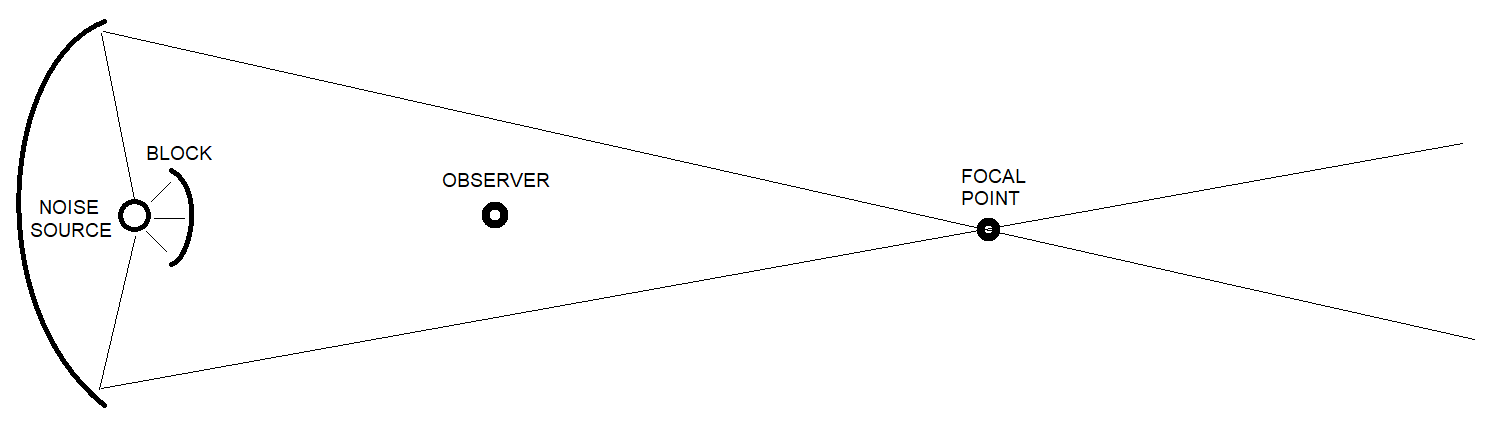
Second answer: Make a large (10m?) bowl-like reflector and place your sound source at the closest focal point.
For large distances, this will be very similar to a Parabolic reflector, but with the important difference that the reflected sound would not be "parallel" but focused. For a very close listener the sound is loud. Moving away it first gets quieter but then increases as the focal point is approached.
Now, I assume you would prefer this to work in any direction. I'm not sure how feasible this would actually be, but here goes:
EDIT: I've updated the third answer, and I'm much more confident it could work now. The old "mushroom" from my previous answer is replaced by another shape, perhaps more resembling the underside of a "flower".
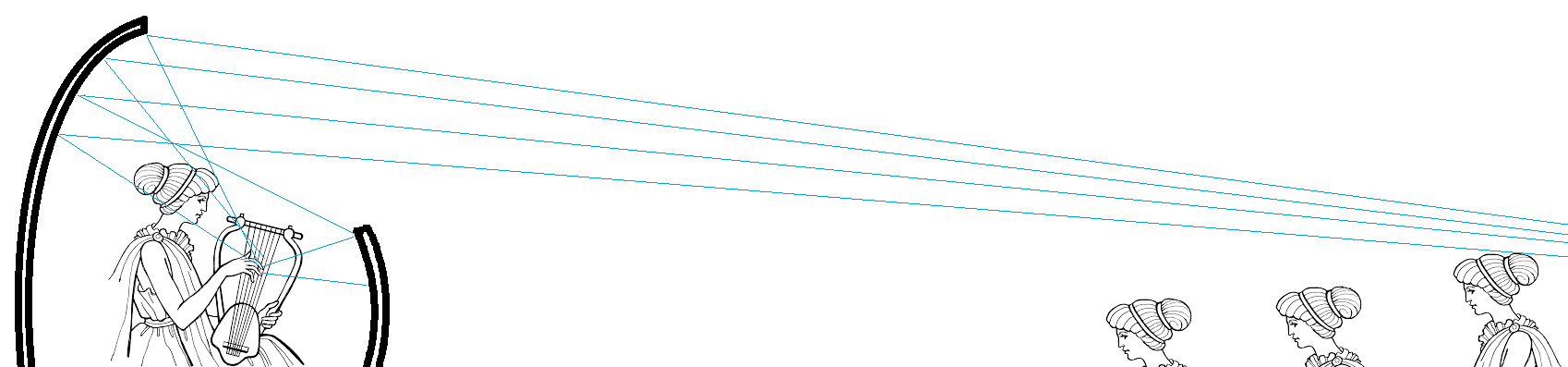
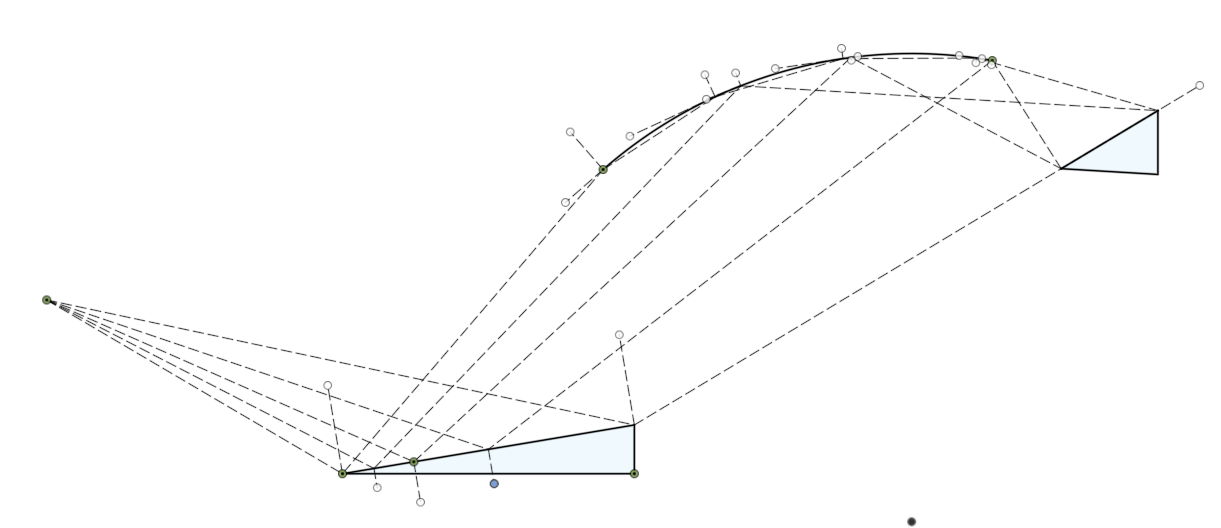
Third answer: Build a large round ceiling, shaped like this:
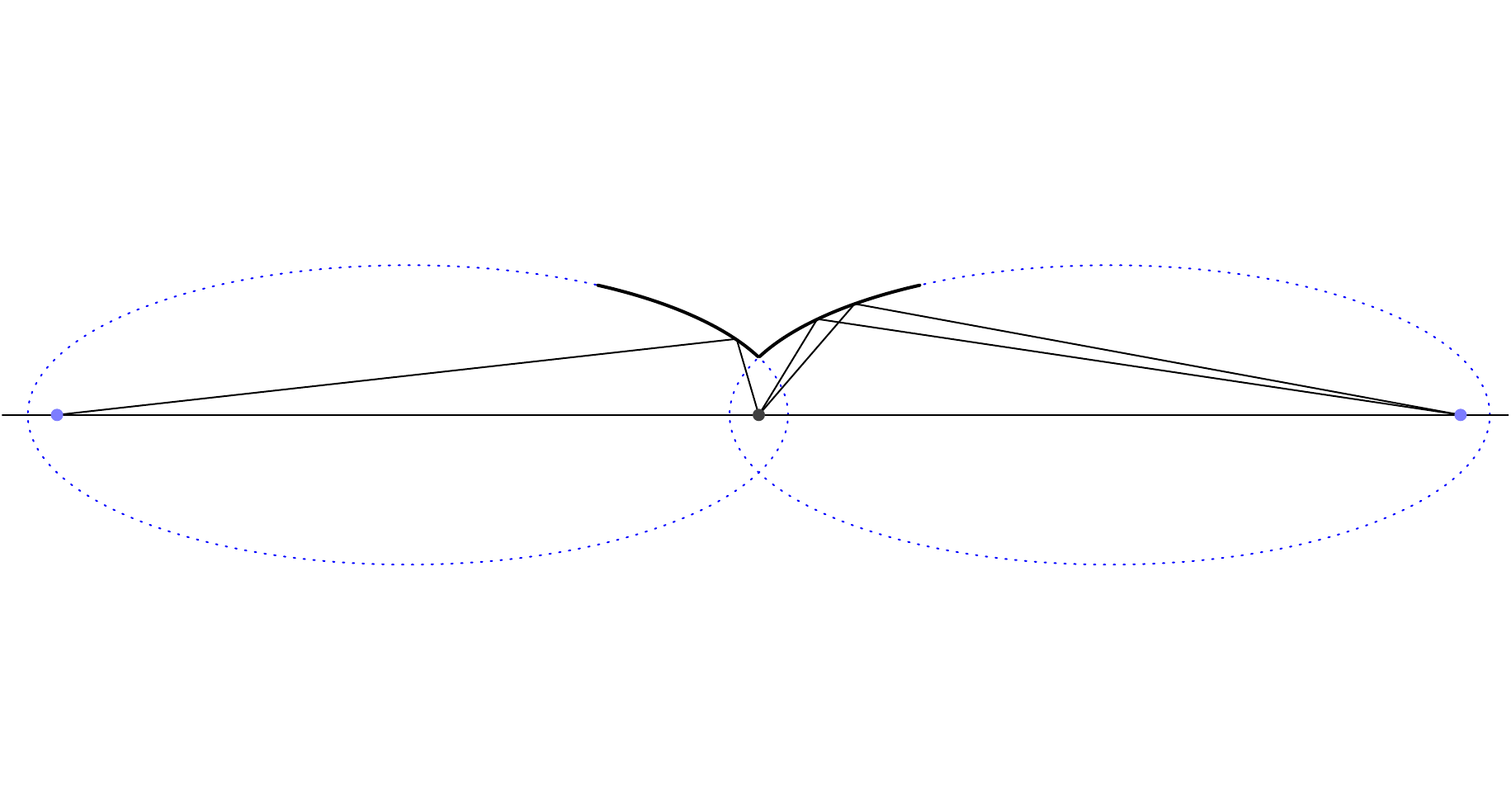 The cross-section is shaped like segments of two intersecting ellipses with a shared focal point at the same height the instrument would sound (preferably near ground level to get as much gain from reflections from below as possible) at the centre. The other focal point would form a circle around the player. Like I've drawn it, the "focal ring" would be at the ground, but you'd probably want to tilt the ellipses to get the ring at ear level.
The cross-section is shaped like segments of two intersecting ellipses with a shared focal point at the same height the instrument would sound (preferably near ground level to get as much gain from reflections from below as possible) at the centre. The other focal point would form a circle around the player. Like I've drawn it, the "focal ring" would be at the ground, but you'd probably want to tilt the ellipses to get the ring at ear level.
You MIGHT even get away with using a reflector small enough to be almost portable! The more of the elliptical arc you cover the more amplification near the ring. Most of the reflection happens near the centre, so once you reach a certain size you don't gain enormously from making it just a bit bigger (until the edge gets close to the other focal point) The proportions shown in my image should give a noticeable effect, as long as it's large enough that much of the sound goes above the heads of near by spectators.
If you only care about certain directions, and especially if you want a portable solution, my second answer is probably the most relevant. It could be adapted further depending on precisely what context you have in mind.