Not a tank that could survive being shot with a 1 GJ railgun (pretty sure nothing can survive that), but a tank that can withstand the recoil of a 1 GJ railgun that's been mounted to it.
Obviously, a way to anchor it to the ground is in order to keep it from flying away is required, but what about the frame itself actually withstanding the force? Could the vehicle survive if the barrel and all of the tank's moving parts were made from sheets of a 2d supermaterial like graphene?
SCIENCE
The value with which you must be concerned when designing against recoil is not energy but momentum.
The Kinetic Energy of a projectile is formulated with the equation:
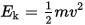
where E is the kinetic energy, m is the mass of the projectile and v is the velocity.
Momentum, on the other hand, is calculated using this similar equation:
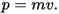
where p is momentum (from the Latin petere, or impetus), m is mass, and v is velocity.
The change in momentum of an object is known as Impulse. The Impulse-Momentum theorem and the Conservation of Momentum are largely used to calculate scenarios involving Newton's Third Law of Motion (Equal but Opposite Reactions, or Recoil if you prefer).
So Kinetic Energy and Momentum are clearly very similar values, but they are not quite the same. Specifically, if you have two guns that fire different projectiles, one is fast and light and the other is slow and heavy, the energies might be the same but the recoil will not be the same, or vice versa. This is due to the velocity squared we see in the Kinetic Energy equation. This means we need to define our projectile exactly in order to properly analyze this problem.
HISTORY
Now, with that out of the way, we can look at some history. There have, in fact, been weapons (projectile weapons, even) that have delivered kinetic payloads on the same order of magnitude as your railgun. Specifically, the Schwerer Gustav railway cannon used by Nazi Germany could deliver a payload of about 1.8 gigajoules (v = ~720 m/s, m = ~7100 kg). The recoil the weapon would experience was about 5,112,000 newton-seconds (a unit we don't really need to care about too much).
The astonishing energy delivered by the Great Gustav was largely achieved due to the massive projectile, and not so much due to the speed of the projectile. In theory, we could definitely reverse those attributes and launch a small projectile at hypersonic velocities. If we had a muzzle velocity of 1% the speed of light, for example, the projectile would only need to weight like a milligram to deliver a gigajoule of energy. Unfortunately we have an issue in this regard.
It turns out there is an upper limit to velocity while inside the atmosphere. Atmospheric Heating will literally vaporize things travelling too fast (which is one of the many reasons you can't launch satellites into space with a railgun). The fastest you can reasonably go is about 7000 m/s. Even at this speed most materials will vaporize too quickly to be useful, but super dense materials like Uranium or Iridium will survive well enough. With this speed as the upper bound, if we wanted to strike with 1 gigajoule the projectile would have to weigh about 40 kilograms. That's not super unreasonable, especially considering Great Gustav's shells weighed 7 tonnes.
With these numbers in mind, we can figure out how much impulse the railgun will produce when it fires: 280,000 newton-seconds. Compared to Gustav's figure, that number is paltry. To compare some others, the Mark 7 16"/50 guns aboard the Iowa Class Battleship produce a little over 1,000,000 newton-seconds of impulse. The primary weapon of the Abrams MBT produces about 10,000 newton-seconds of impulse.
So what does all this mean? In my opinion, a 1 gigajoule railgun would need to mounted aboard a small ship, or perhaps a very large self-propelled artillery piece (I'm fairly confident weapons like the M110 Howitzer produce similar recoil, but I could not find any definitive ballistic data). Large stationary artillery would also work, but such weapons were never really effective.
If I forgot something, or there is data I missed, please let me know!
EDIT: Turns out the M110 Howitzer produces about 50,000 newton-seconds of impulse, so I was actually mistaken about the magnitude of the recoil involved. This suggests a self-propelled artillery piece with our hypothetical 1GJ weapon will need a carriage much larger than used with the M110. I doubt it will need to be 5x bigger to manage the 5x recoil, but it will need to be significant.
EDIT 2: Ok one more edit! I found a weapon with a very similar recoil value: the German 28cm/45 SK L/45 Naval Gun. It generates an impulse of about 260,000 newton-seconds, which is close enough for our purposes. That link contains most of the relevant ballistic and dimensional data, but the long and short of it is that a weapon that size is usually mounted on a large ship (in this case it was the primary armament of some of Germany's Dreadnought-era capital ships) or as a fixed artillery piece (shore defense or railway cannon). In my humble opinion, it would be very difficult to mount this cannon on a tank, but at least some of that difficulty will be a result of the gun's weight. Our railgun will not have the same kinds of weight restrictions as a traditional cannon, though, so I think it's still feasible, especially if we're using modern materials and techniques.
You might be surprised to hear this, but there are have been weapons IRL that have fired projectiles with 1GJ+ muzzle energy.
The best way I can think of to make something the size of what you're describing not get blown away by the recoil of its own weapon is to give it a VERY long barrel for the projectile to accelerate down. This is a losing proposition eventually, the velocity (assuming the same force pushing all the way down the barrel) only increases by the square root of the length.
The second option I can give you is that you could fire a VERY light projectile. muzzle energy increases with the square of the velocity, so if you halve the weight of the projectile, you could (in a perfect world) get double the muzzle velocity, which in turn gives you 4 times the muzzle energy.
Real world problems of having an extremely light projectile: drag will slow it down very fast so you wouldn't have very much effective range, secondly is that mass is very helpful to have when you want a projectile to penetrate material.
If you are content with just disintegrating smaller objects, the small projectile scheme works well since that energy gets converted into basically an explosion on whatever gets hit, but don't think that it would punch a hole through feet of steel, it would just wreck the outside of it.
Build a shell that is a vacuum container, so the mass spins inside it. Put most of the 1GJ into projectile spin before you launch it. Then you can launch it with conventional velocities, so it hits the enemy 5 miles downrange instead of flying past the moon and hitting Jupiter's rings 3 years later.
One nice thing about a railgun is that, unlike explosive propellant, the force is not transferred to the projectile all at once, but along the entire active length of the rail. So the longer the active acceleration distance, and thus time, the less force you need for the same ending velocity. (so less force per unit time on the tank)
Having the frame survive the recoil is not such an insoluble problem, you basically have to transfer the recoil shock out to something external to the tank. Modern MBTs are usually made out of steel (covered in layers of other stuff) that approaches a foot thick. Historically, we have seen tanks and other vehicles made of significantly thicker steel than that. It is not impossible to make the frame effectively one piece, not deformable, and capable of transferring recoil away from the tank's body.
There have been tanks built that exceeded 100 tons, and mega artillery that was considerably bigger than that, so it is possible to build something that could carry such a gun, but it would obviously be very heavy and slow.
Large, self-propelled artillery (like the Russian 2s7 Pion SPG) use a blade like a bulldozer (Called a "recoil spade" -thanks, T) at the back of the vehicle to transfer recoil into the ground. The SPG stops, deploys the hydraulic blade, raises the barrel, and goes boom. Shock is transmitted directly into the ground below the vehicle. Your tank could do the same thing, but would obviously not be able to move and fire at the same time.
Alternately, you could devise some sort of rocket-like device that would fire on the back of the tank turret at the same time as the railgun. That would mitigate recoil but would have a limited fuel supply.
Odds are anything that has that much power will not be mobile...a tank that can handle that just can't exist as we currently understand the word tank.
The energy requirements and the impact/stress of such a weapon necessitate that it be stationary. A tank simply doesn't have the weight and structural integrity to handle that much force.
Additionally the power requirements mean the tank would have to carry around multiple times its weight in batteries/capacitors to fuel the cannon (I don't know the math specifically)
Something putting out 1GJ of force has to be able to offset that...a tank would go flying through the air from the recoil.
You could build artillery style and put the whole thing on some sort of modified train rails so it is held down and allowed to slide back after firing.
Graphene isn't a great idea. Strong yes, but relatively brittle. The frame materials will need toughness and strength. Steel for example has arguably the best balance of toughness and strength.
For tanks, this will be tricky, as you'd want the gun to be loaded from the inside. This is clearly not going to be possible, due to the high currents involved in the initial burst. In fact, it'd probably be advisable to raise it well above the passenger area of the tank, to prevent accidental electrocution by induced currents.
Since you must mount it outside anyway, your vehicle becomes less like a tank and more of a motorized ladder. That being the case, to improve your stability, mount your ladder on a non-conducting surface on the ground.That way, as long as your gun is pointed above the horizontal, the reverse thrust will act to drive the non conducting base into the ground, from where it will have to be dug up. In short, you can mount a 1GJ railgun on a tank, and the tank won't break apart/fly away when the gun is fired, except that it will be more of a single shot rocket launcher. Apparently the rails can't take more than one shot.
Recoil is essentially impulse, and impulse is essentially Force/Time. To reduce recoil, I can reduce the force, or I can increase the time over which that force is applied. Twice the time, half the recoil, four times the time, quarter the recoil, and so on.
Suppose I apply, say, 1GJ of energy total to push my broken down car. If I apply that all at once (One mother of a punch) I'm going to explode both my hands and my car. If I apply that over a half hour, everything is fine.
Now Imagine mounting your railgun in a very long cradle. The tanks barrel isn't so much a barrel as it is a very, VERY long recoil dampener with the gun inside. Perhaps the barrel/cradle is 40 feet long and pokes out both ends of the turret. As it fires, the actual railgun slides backwards, 'gently' decellerating and slowly transferring its energy into the chassis instead of delivering a single earthshattering blow.
