I have an idea for a planet that orbits two stars. These starsslowly orbit each other, have equivalent masses/sizes, and while one is slightly red, the other is slightly blue. When I talk about the color, the stars are primarily white, with slight tints, just enough to notice. A year is about as long as Earth's, and a sun cycle (day) is 24 Earth hours. The rotation of this planet causes eight hours of night time, say, from eight to four o'clock on equinoxes. One sun would rise at four o'clock and set twelve hours later, and the other would rise at eight o'clock and set twelve hours later. Is this system possible? I also wonder how seasons would affect the sunrises and sunsets if the planet had a tilt about the same as earth, but more important is how plausible this planetary/star system is. I'm a little bit worried about the stars, I read somewhere about red stars being smaller and blue stars being larger.
-
$\begingroup$ There would be some redshift/blueshift, though I don't know how significant it would be. $\endgroup$– iAdjunctCommented Jan 3, 2016 at 3:52
-
$\begingroup$ @iAdjunct Probably only detectable with specialized instruments. I haven't run the numbers, but I suspect the Doppler shift of the pair of stars as they orbit each other would be tiny. $\endgroup$– userCommented Jan 3, 2016 at 12:36
4 Answers
I'm afraid what you're asking for is going to be quite tricky, at least not with a universe that operates like ours. I'll try to discuss the individual issues, and then offer some suggestions on what you can do:
Same mass, different color?
... have equivalent masses/sizes, and while one is slightly red, the other is slightly blue.
Stars do come in a wide range of colors, depending on their composition and color. For the most part, however, two stars of roughly the same mass and diameter will have virtually identical spectra (color). In fact, astronomers use the spectral characteristics of stars to classify them into categories of size and mass. For more information, the stellar classification page on Wikipedia gives a decent introduction, although it is somewhat technical.
Year length and sunrise?
A year is about as long as Earth's, and a sun cycle (day) is 24 Earth hours. [...] One sun would rise at four o'clock and set twelve hours later, and the other would rise at eight o'clock and set twelve hours later.
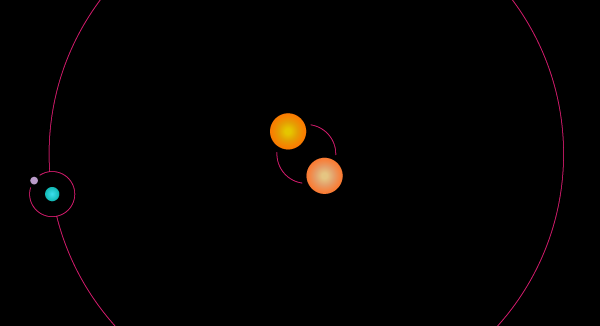
Unfortunately, this would be difficult. So-called P-type binary star systems look like this:
(Image mine, and obviously not to scale. The stars would be much closer to each other and much much farther away from the planet, relative to how I've drawn them, but then you wouldn't see anything.)
In order to have an Earth-like year and be in the Goldilocks zone around your two stellar objects, your planet would have to be quite distant from the center of the binary pair.
That means that the stars are going to rise and set at very nearly the same time. In the daytime sky, they will appear very close together, overlapping much of the time.
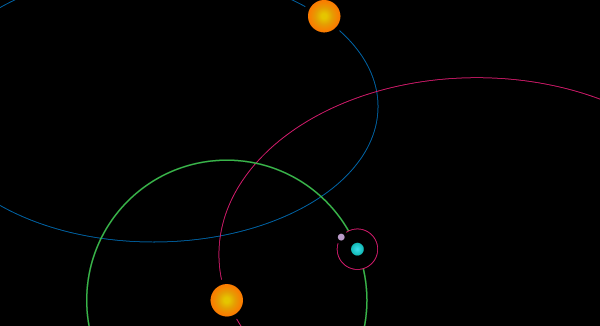
S-type systems
There's another possible configuration for the star system:
For much of the year, the planet would be "in between" the stars. Unfortunately, this means extreme temperature variations (including the planet probably being burnt to a crisp), but also in near-constant daylight. It's also probable that the "orbit" would be complex and irregular, and chances would be good of the planet actually getting flung out of the solar system altogether, becoming a rogue planet.
These s-type systems are quite common, but the sunrise pattern would only hold true for a small window of time (a few months) over a large timescale (many many years, until the stars' orbits around their barycentre and the planet's season aligned again).
Seasons
I also wonder how seasons would affect the sunrises and sunsets if the planet had a tilt about the same as earth
Seasons would be pretty much the same as they are on Earth.
In fact, for most practical questions concerning P-type binary star systems, it's helpful to think of the two stars as a single mass whose center is the center of the solar system. All other things equal, the orbits, effective gravity, and radiation (light, heat) aren't significantly different from what we experience in our own solar system.
With S-type systems, it is of course more complicated. The orbital periods for the stars can vary widely, while the Earth-like years for the planet would result in relatively normal seasons most of the time, however things can get exotic in a hurry if the planet's orbit (nearly) intersects the non-bound star's orbit. At best, you'll see an extremely hot summer. At worst, the planet's orbit will be radically changed, or the planet will be thrown out of the system altogether, sending its inhabitants into the coldest winter on record...
Of course if one or both of the stars are significantly different (i.e., something off of the main sequence), that's where you can start to get some different effects without crazy orbital mechanics, but that's only because the color, temperature, radiation, mass, etc. would be different—nothing to do with it being part of a binary system. I'll talk about this a bit more in the next section.
How to make it work
Different types of stars. Stars of different sizes, or at different points in their life cycles. They are never going to be very different, visually (to the unaided eye, stars mainly just look bright), so the most striking difference you're likely to get would be if you have stars of different sizes.
Multiple sunrises, I don't know. To make that work, the planet would have to be so close every living thing would be burnt to a crisp. However, there are many things you can do creatively with a binary star system: you have two different points of light in the sky, you can have partial solar eclipses, different "suns-worshiping" religions, twice as many solar flares wreaking havoc with electronic equipment, opportunities for divergent scientific history (it would be easier for early astronomers to figure out that the stars are orbiting a common point in the sky, so perhaps the heliocentric model could be proven much earlier than it was on Earth).
-
$\begingroup$ Really excellent answer. I just have one concern: In the image for s-type orbits, it doesn't quite look like the orbits of the two stars share the same focus, which should be the case. Am I looking at the image wrong? $\endgroup$– HDE 226868 ♦Commented Jan 3, 2016 at 14:19
-
1$\begingroup$ Thanks for your answer, it really helped. At this point I'll just kind of drop the sunrise/sunset thing and go with an elliptical orbit around a pair of binary stars. $\endgroup$ Commented Jan 3, 2016 at 14:36
-
$\begingroup$ @HDE226868 Thanks! It was a quick sketch, and I just kind of eyeballed the ellipses' foci. The barycentre would be in the center of the small ()-shaped wedge where the big pink orbit and the big blue orbit intersect (assume the stars have roughly equal masses). Possible it's off a little bit, but looks about right to me? $\endgroup$ Commented Jan 3, 2016 at 20:27
-
$\begingroup$ Wait, you made it yourself? Wow, that's awesome! $\endgroup$– HDE 226868 ♦Commented Jan 3, 2016 at 22:43
-
$\begingroup$ One dumb question - wouldn't that S-Type system be prone to spontaneous selfdestruction? Uhm... you said this may be a scratch, but what happens if booth stars meet at the () Intersection? Shouldn't they pull each other out of orbit? $\endgroup$ Commented Jan 5, 2016 at 13:13
Sort of
You can have 2 stars, and you can have one rise 4 hours after the other. Just stick your planet in the L4 or L5 Lagrangian point of your binary star system. This is stable over large time-scales and puts the stars 60 apart, which gives you a 4 hour gap between sunrises (assuming a 24 hour day).
Sounds good. What's missing?
Those Lagrange points are only stable if your central star is at least 25 times more massive than the other one. It's going to be a lot brighter.
-
1$\begingroup$ So this system rules out the condition that the suns have "equivalent masses". But in return for giving up this condition, the "different colors" condition becomes easier to support. It seems like a reasonable trade-off to me. $\endgroup$– David KCommented Jan 3, 2016 at 15:25
-
$\begingroup$ Although it's a cool idea, I doubt such a system would be hospitable to life. The brighter star must be ~100 times brighter for the system to be stable in the long term. All stars mass at least ~0.08M☉ (the bare minimum for a red dwarf); this puts a lower bound on the larger mass of about 8 M☉. Stars this large are currently thought to be less habitable, and also stay in main sequence for only ~80M years, a lifespan which rapidly decreases as the mass increases further. $\endgroup$ Commented Jan 4, 2016 at 2:20
-
$\begingroup$ @ThomasKwa if you read the stability condition from the third paragraph of your link, you'll find that for a relatively low mass planet, the brighter star must be at least 25 times more massive (than the fainter one), for a lower bound of 2 solar masses. $\endgroup$ Commented Jan 4, 2016 at 2:41
-
$\begingroup$ Although if it's a main sequence star, that gives approximately 10 solar luminosities... $\endgroup$ Commented Jan 4, 2016 at 2:44
-
$\begingroup$ @frodoskywalker - unfortunately there are mass ratios necessary for the objects in Lagrangian orbits. The most massive object has to be many times the mass of the second which has to be many times the mass of the third. First object stellar mass, second planetary mass, third asteroid mass. For a habitable planet that humans can settle, or have interesting lifeforms, or intelligent natives, the stars in the system have to have a certain narrow range of masses. They cannot have masses differing enough to be the first and second largest masses in a Lagrangian orbit. $\endgroup$ Commented Feb 19, 2018 at 23:30
I think it could work (roughly) as long as we can cheat on the mass. First, use a binary system where your planet orbits a midsize, red dwarf star, which would supply some of your light (at well under 1 AU) while a hot star with maybe 1.5 solar masses orbits as a few AU (the two stars orbit around each other, while your planet orbits the red star). Assuming you aren't tidally locked to the red star, as you orbit each star would spend (up to) 12 hours from sunrise to sunset (though the exact times would vary by time of year). If you balance the orbits right, each star would appear at close to the same size.
For instance, consider the scenario (it's close, but there are better combinations):
You orbit a red dwarf star of mass 0.5 solar masses at a distance of 0.35 AU. You might or might not be tidally-locked at that distance, so we can just apply handwavium and say you aren't.
There is a hot F-type star with 1.5 solar masses at a distance of about 3.2 AU. That would be a yellow-white star.
You would get about almost exactly half of your heat/energy from each star (each would supply 1/2 solar radiance). This is because the hotter star is about 80 times as luminous as the red dwarf in absolute (bolometric) luminosity.
As far as stability, this may be a bit dicey (to improve it, move the planet closer to the red dwarf a bit, and the distant star further away). HOWEVER, a fast calculation shows that the radius of the Hill Sphere around the red dwarf (where the gravitational pull of both stars is balanced) is 1.2 AU and a popular rule of thumb is that orbits are stable inside of 1/3 of the Hill Sphere radius.
In addition, the paper by Holman and Wiegert on orbital stability of planets in binary star systems gives a formula for the radius of a stable orbit in binary star systems, and the formula for S-type systems gives a stable orbital radius of 0.57 AU or less*, as long as the eccentricity of the binary orbit is low.
*-(For very low binary eccentricity, the formula for the maximal stable orbit around a star in a binary star system is approximately $d = (0.464 - 0.380\mu)\alpha$ where $\mu$ is the relative mass of the secondary star, or, in this case, $\mu = 0.75$, and $\alpha$ is the distance between the two stars)
-
1$\begingroup$ A planet orbiting a red dwarf star, while there is another far more massive star orbiting the red dwarf star fairly close by? Even ignoring the issue of frame of reference, you may want to look up the term perturbation. This is actually somewhat similar to the question of why we don't see natural satellites around moons. $\endgroup$– userCommented Jan 3, 2016 at 12:38
-
$\begingroup$ The red dwarf would orbit the F-type star, not vice versa (well, they would orbit around their center of mass, but that would be closer to the F-type star). Also, as @MichaelKjörling said, the planet won't last in that orbit for long. Stability will be a huge problem. $\endgroup$– HDE 226868 ♦Commented Jan 3, 2016 at 14:31
-
$\begingroup$ Moons don't have moons usually due to either (1) very tiny Hill Sphere radius or (in the case of our Moon) (2) orbital decay due to tidal forces. Neither would hold in the case I described. $\endgroup$ Commented Jan 3, 2016 at 22:05
If we can cheat a bit we can have two stars of the same mass but different colors. Their size will differ, though!
To start with, lets take a star system consisting of a 2 solar mass star and 1 solar mass star, assorted planets.
Now, we also need a system containing a 1 solar mass star and the planet of interest. Other planets are irrelevant.
Apply lots of time to this system, wait for the 2 solar mass star to enter it's red giant phase. Now, they run into each other. The two 1 solar mass stars impact and merge into a single 2 solar mass star which will still be a main sequence star (this is one of the hypotheses for blue stragglers) Through some incredible luck the planet ends up in a suitable orbit around both stars.