How many kids?
This is the toughest factor to find. While Santa isn't specific to a single religion, traditions differ in various parts of the world, and belief rates in Santa Claus are not tracked by the WHO, looking at Christians seemed to be a reasonable, if imperfect, start. According to 2010 estimates, there are 2.2 billion Christians in the world. I have no idea how many of them are kids, but we're all kids at heart, aren't we? So I'll just go with 2.2 billion kids in my calculations. That might sound like a huge overestimate, but, as you'll see, even if that number is off by a factor of ten, it won't make much difference.
No matter how you look at it, though, that's a lot of chimneys.
How many cookies?
Assuming Santa heeds his doctor's advice and doesn't eat the entire plate of cookies (he just eats one—he has to keep up appearances, after all!):
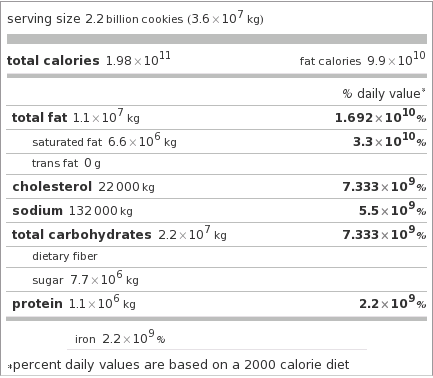
Excluding the obligatory sip of milk, by the time the night's over, Jolly Ol' Saint Nick would have ingested food energy (it's mostly empty carbs anyway!) equivalent to the yield of a 200 kT thermonuclear bomb!
Naughty or Nice list: 225 TB
Accounting for names (100 characters), identifying information (200 characters), address/coordinates (200 characters), photograph (100KB) (he has to be able to recognize the little tykes on sight if one of them happens to wake up in the middle of the night), Santa would need storage for around 100KB on each kid.
$$100 \text{KiB} \cdot 2.2\times10^9 = 225 \text{TiB}$$
Very roughly speaking, that means Santa's carrying around about a hundred 2TB external HDDs (2015 budget: US$7,500, chump change for Kris Kringle!). That's a fair amount of storage for one man to carry around, for sure, but compared to Santa's other challenges, a hundred hard drives would be the least of his worries.
Cargo
This one's easy: 2.2 billion Red Ryder Lever Action BB Guns at 1kg each is 2.2 million metric tons.
Volume-wise, let's say you can get about 10 presents per cubic meter (don't want to smash the pretty bow ribbon!). For a sense of scale, check this query:
- 44% of the volume of Sydney Harbor
- 69% of the total annual volume of oil transported by oil tankers worldwide
If I were Santa and refused to delegate the actual home visits, I would at least mobilize a huge global shipping network months in advance, to ship the presents to local warehouses and perhaps even neighborhood depots from there, for staging purposes. That way on Christmas Eve itself, he has much less work to do, and much, much less cargo to drag around the globe.
Time estimate
This is where it starts to get rough.
According to the map on the Wikipedia page linked above, Christians are spread out East-West more or less across the entire globe. Very roughly speaking, but precise enough for our purposes here, let's say Santa has 24 hours to deliver all of the presents. Can one man do it?
$$24h / 2.2\times10^9 kids = 1.091\times 10^{-8} h/kid = 39.27 \mu s/kid$$
I hope Santa's a fast eater!
Forces on Santa
It's hard to get a precise estimate of the distance Santa will be traveling, how much time will be spent taking off, flying, and landing, versus just going door-to-door in apartments. While acceleration during takeoff would surely be very bad, why don't we just consider the best possible case for Santa's delivery: every kid in the world is beamed with a transporter, semi-comatose, into Russia, in a single line, shoulder to shoulder, with their arms outstretched. Santa would then just need to take a step, stop, hand out a present, and repeat. Let's say taking a step and stopping take 2/3 of the time (1/3 accelerating, 1/3 decelerating), and handing out the present takes the other 1/3.
Santa therefore has $39.27/3 = 13.09 \mu s$ to accelerate through roughly half a metre. Thanks to Newton's 2nd law, we know:
$$s = v_0t + \frac{1}2 at^2$$
With a little arranging, and $s = 0.5m, t = 13.09 \mu s, v_0 = 0$ (he accelerates from a standstill), we get:
$$a = \frac{2s}{t^2} = 5.84 \times 10^9 m/s^2 = 5.95\times10^8 g$$
That's 595,000,000g. You can check this page for the gory details of human tolerance, but, really, don't bother. Most humans wouldn't survive 40g-50g or so. At 595 million g, Santa is now, at best, a buttery sweet-smelling liquid.
But there's another, worse problem than that!
(Worse than Liquid Santa? This can't be good...)
No matter what kind of fuel you use, moving Santa in one direction means an equal force in the opposite direction is required. As it turns out, that will amount to a lot of energy. How much? $6.4\times10^{20} \text{J}$, which is about 11% of the world's total energy (oil) reserves.
But what happens when you burn all that heat in a short amount of time?
Liquid Santa is now setting off a continuous chain of fireballs, with a total yield equivalent to $1.5\times 10^{11}$ tons of TNT, which is more than enough to vaporize every child on Earth, and plunge the rest of us into a nuclear winter severe enough to have a deadly White Christmas, all year round!
Anyway, I think that takes care of your cargo problem!

