The tilt of the axis of rotation of the entire double planet system has no effect on the visibility of one planet from the other; what it does have an effect on is the course of the sun across the sky. What does affect the visibility of one planet from the other is the fact that their axes of rotation are not perpendicular to the orbital plane of their revolution around the common barycenter.
Here is a drawing, made with the assumption that the axes of rotation of the two planets are parallel (which they don't have to be), both are tilted 25° with respect to the ecliptic, and that the plane of rotation of the two planets is inclined 8° with respect to the ecliptic. (Click to embiggen.)
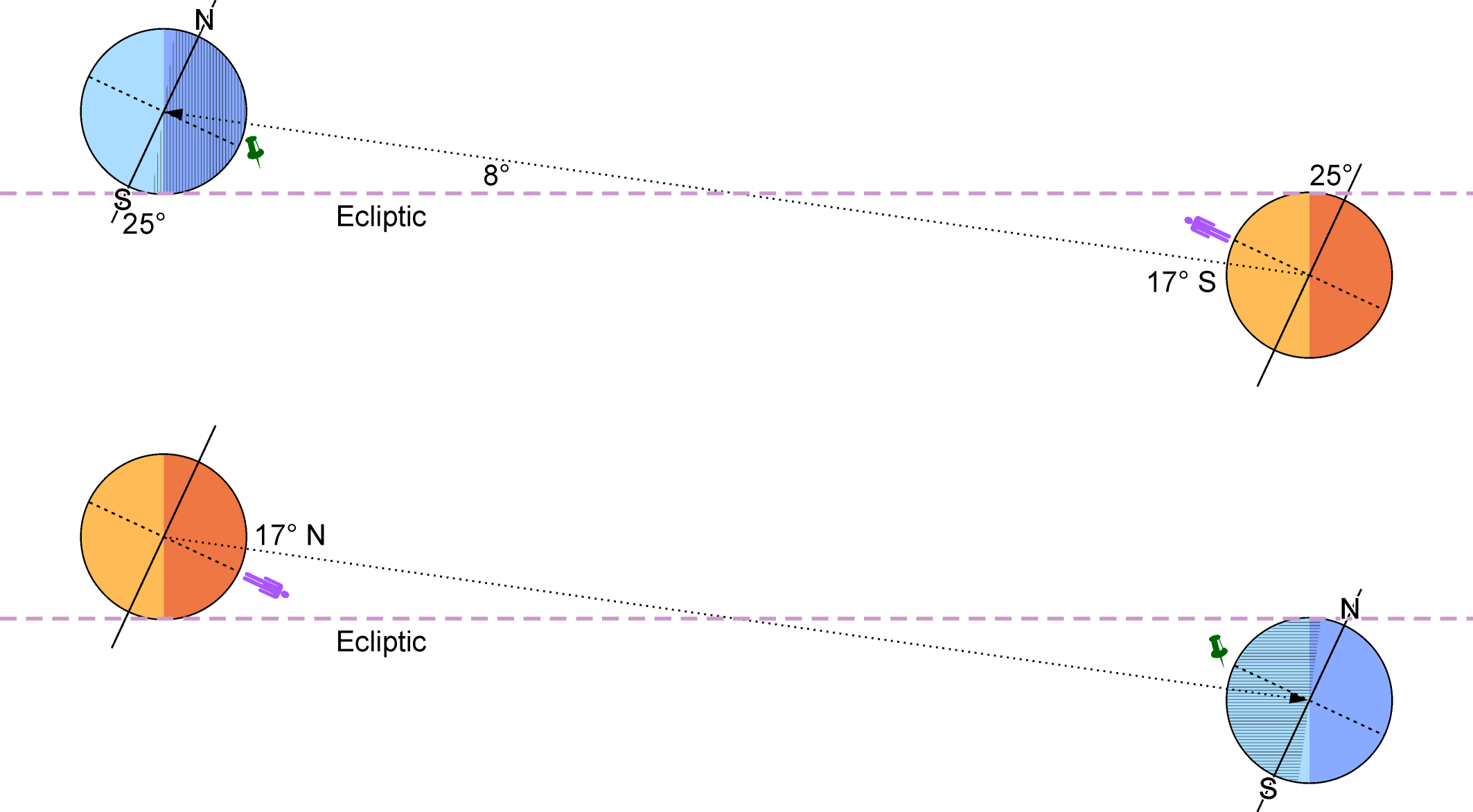
On the diagram I have marked the axes of rotation and the equators of the two planets. I have placed a purple observer on the orange planet, and a green fixed target on the blue planet. The diagram shows the planets at two opposite points in their revolution around the common barycenter.
Note that in such a double planet configuration there is natural prime meridian on both planets, right on the line between their centers.
The hatched part of the blue planet is the part which is visible to the purple observer on the orange planet; note that the observable part varies during the cycle: in the top configuration, the purple observer can observe the north pole of the blue planet, while in the bottom configuration the purple observer can observe the south pole of the blue planet.
Alternatively, you can think of the hatched part of the blue planet as being the part from where the orange planet is visible.
Note that between the two opposite positions the blue planet moves from 17° south from the point of view of the purple observer to 17° north, a total 34 degrees.
The two planets move from the top configuration to the bottom configuration and back during each revolution around the common barycenter, that is once every solar day.
The difference between the 25° axial tilt and the 8° inclination of the orbital plane is 17°. This means that the purple observer, who is placed on the equator of the orange planet directly in line with the blue planet can move wherever they want between 73° latitude south and 73° latitude north and the blue planet will remain visible at all times. But above 73° latitude the blue planet will dip below the horizon for part of the day.
As the observer moves east or west, the blue planet will be lower and lower in the sky, and its motion instead of being up and down will be more and more horizontal; when the observer is 90° offset from the direct line between the planets, the other planet will appear exactly on the horizon, moving in a horizontal arc of 34°. Beyond that, the blue planet is always below the horizon.
Ah, but real life orbits are almost never perfectly circular. They are almost always eliptical, so that in adition to the libration in latitude and the north-south movement of the other planet in the sky there will also be a libration in longitude and an east-west movement...
For further delight:
How does the Earth move in the sky as seen from the Moon? on Astronomy SE. This is a good reference to understand how the blue planet moves in the sky from the point of view of the purple observer on the orange planet.
The Wikipedia article on libration. This is a general introduction to the geometry of why tidally locked does not mean that the view is unchanging.
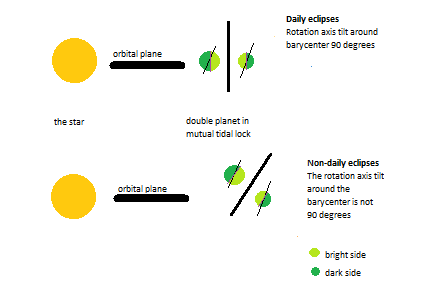In the previous question I was told that in order for the double planet system not to have eclipses every day, their rotation axis tilt around the barycenter of mass must not be 90 degrees.
