2
$\begingroup$

I’m at a bit of a wall here. I have numbers for mass, radius, and gravity for my planet (Neogaea), but the density is escaping me.

Mass: 1.31 earth masses

Radius: 1.027 earth radius

Gravity: 1.25 earth gravities

This gives a density of 0.289 (with earth being 1).

I don’t know much about this, which is why I’m here, but this seems... low. Neogaea is a mostly water world, so this could work. The main landmass spans from the North Pole to past the South Pole, there’s are various islands around this landmass, the rest is ocean.

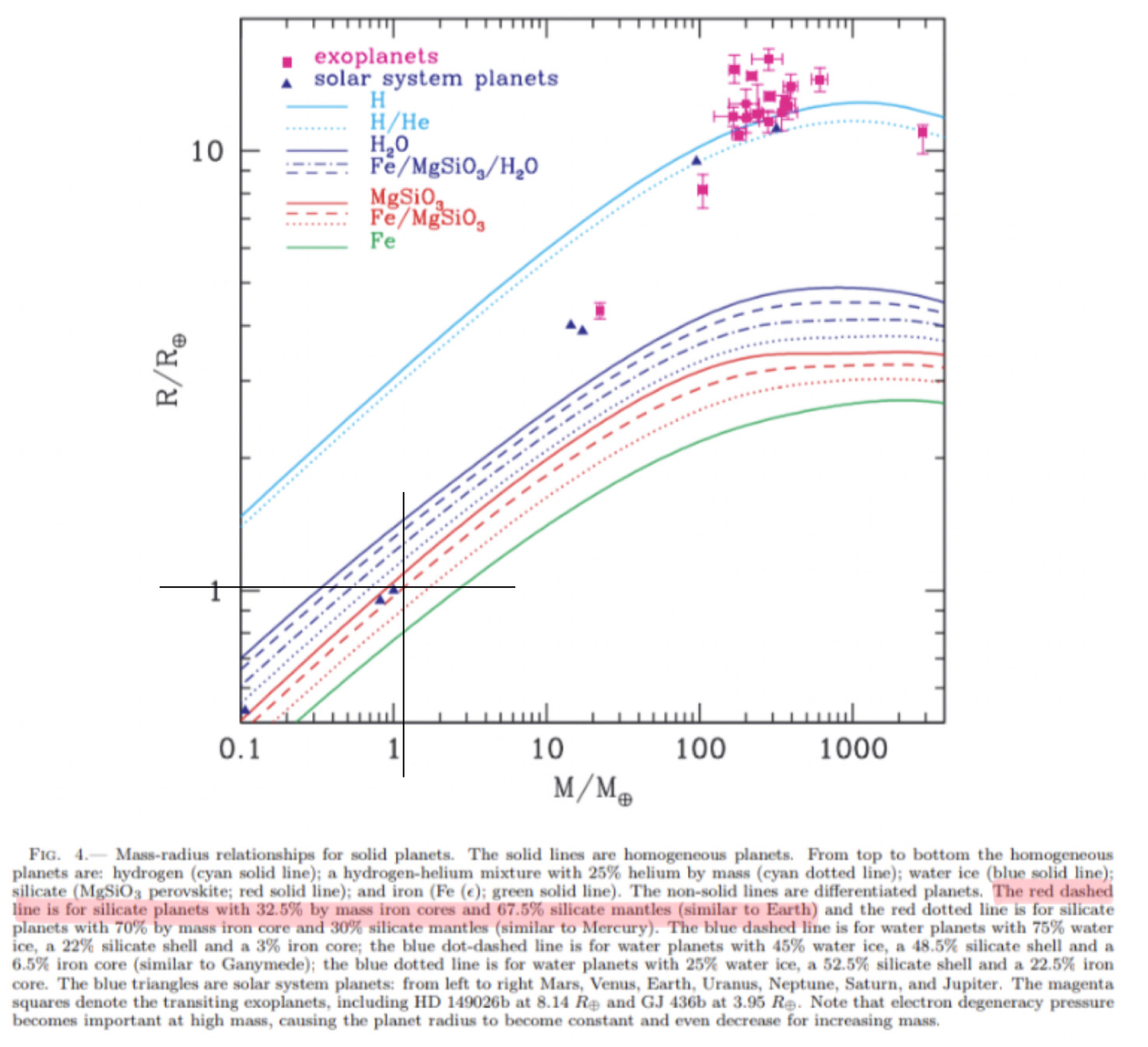
The planet is meant to be habitable, and similar to earth (in order to make it habitable) enter image description here

My question is whether or not a density of 0.289 makes sense for this planet. If this isn’t clear enough, tell me and I will try to clarify further.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ How did you calculate the density? $\endgroup$
    – L.Dutch ♦
    Commented Jan 12, 2021 at 20:26
  • $\begingroup$ (Volume) 4/3п1.027^3= 4.54 (Density) 1.31/4.54= 0.289 $\endgroup$
    – Ren
    Commented Jan 12, 2021 at 22:09
  • $\begingroup$ What is the volume of Earth assuming that its radius is 1? $\endgroup$
    – L.Dutch ♦
    Commented Jan 13, 2021 at 4:47
  • $\begingroup$ Just fix you math, your density there is 1.20937etc, which sound just about right. Maybe a bit high, but well within reason. $\endgroup$
    – PcMan
    Commented Jan 13, 2021 at 11:02

1 Answer 1

Reset to default
6
$\begingroup$

You have blundered somewhere in calculating the density

$density = mass / volume$

$density = 1.31 M_E / (1.027 R_E)^3 = 1.31/(1.027)^3 D_E = 1.209 D_E$

which seems much more reasonable.

To explain why I didn't count the constants:

$V_E =4/3 \pi R^3_E$

$V_P = 4/3 \pi R^3_P$

$V_P/V_E = $$ 4/3 \pi R^3_P \over 4/3 \pi R^3_E $$= R^3_P/R^3_E = (1.027R_E)^3/R^3_E$

Improve this answer
$\endgroup$
4
  • $\begingroup$ I forgot to ask whether or not I had messed up the density, but this is what I was thinking had happened. Thank you for the help! $\endgroup$
    – Ren
    Commented Jan 12, 2021 at 21:15
  • $\begingroup$ Your math is wrong, I believe. The volume of a sphere is 4/3пr^3 according to google’s calculator. Maybe I’m just dense, but I don’t see why you used the radius^3 to calculate the volume. This gives me 4.54 for the volume every time and way I calculate it. Then, 1.31/4.54= 0.289 $\endgroup$
    – Ren
    Commented Jan 12, 2021 at 22:23
  • 1
    $\begingroup$ @Ren, you can neglect constants in ratios. $\endgroup$
    – L.Dutch ♦
    Commented Jan 13, 2021 at 4:26
  • 1
    $\begingroup$ @Ren: I have a spreadsheet that calculates all of this & my numbers match those in the answer. However, I do have a slight difference with your value of gravity. I get 1.244 compared to your 1.25. $\endgroup$
    – user81881
    Commented Jan 13, 2021 at 6:48

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .