Can a ship with a rotating habitat be stable as it travels space that fast for years?
The speed it travels at is irrelevant. The stability is important, but quite unrelated... the interesting gyroscopic effects of rotating bits of spacecraft applies just as much to starships as "stationary" habitats. It is in the nature of starships that you will need enormous engines (or other devices to provide thrust, like a photon sail) and enormous shields and huge reaction mass tanks (for rockets) and supplies and all the rest... this works to your advantage, because the rotating part of the ship can be relatively light and small compared to the rest which will help with stability. Combined with reaction wheels and reaction control thrusters, your ship should be stable enough.
If we accelerate a ship at at those tremendous speeds similar to the Venture Stars capabilities
Pedantry alert: you don't "accelerate at a tremendous speed". Acceleration is the rate of change of speed. If relativity is to be believed (and evidence suggests that we probably should believe in it) one inertial reference frame is pretty much like another. So from here on, I'll ignore speed (almost) entirely, and concentrate on acceleration because that's all that matters.
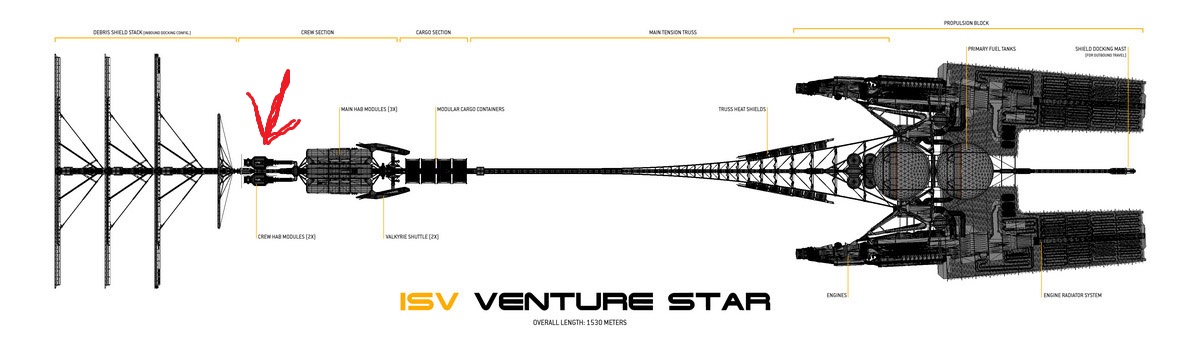
I believe that the Venture Star was designed to be accelerated at 1.5g, which is an impressive figure. At that rate, you don't need your spun gravity... you'd just turn it off. Given that modern day engineering is capable of making structures that can withstand 1G of acceleration for extended periods of time, having your spun sections cope with 1.5G should be no problem, especially if you parked them ahead of time. Which is of course exactly what the Venture Star did.
Can ships with large rotating torus habitats be safely accelerated at tremendous speeds?
You've got three quite separate issues.
Are the support structures capable of standing up to the main engine thrust, even when the rotating sections are parked? I'm going to assume "yes", because having a ship that falls to bits when you press the go button is a bit embarassing.
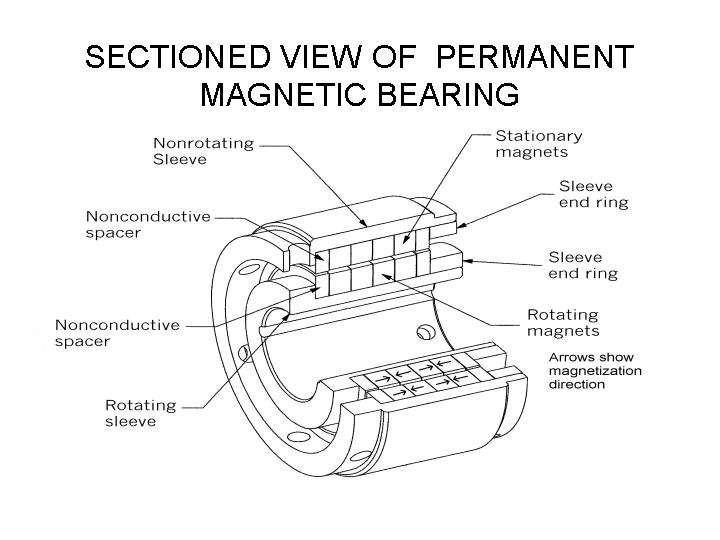
Are the bearings up to snuff?
That's harder to say. With the engines switched off, the forces on your bearings are largely radial. Turn them on though, and now the hub is being pushed forwards and the outside will naturally want to lag a bit. You now have to use thrust bearings instead of simple bearings, which will increase the complexities of the engineering somewhat.
What happens to the direction of artificial gravity when the engines turn on?
It'll start pointing backwards, is what will happen to it, because the contents of the spun sections will be experiencing acceleration due to both centrifugal and engine thrust forces, and those vectors will add up and point somewhere other than straight towards the stern or straight away from the axis.
Here's a couple of helpful diagrams shamelessly stolen from Project Rho's informative page on spun artificial gravity, which you seem to have visited before but might be worth you revisiting:
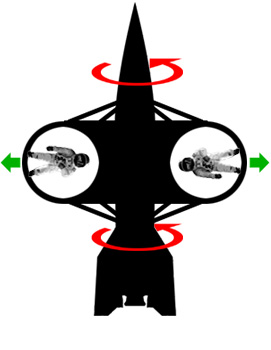
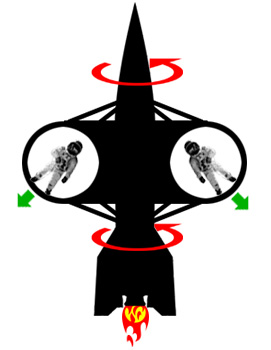
If you're prepared to park your rotating sections before lighting up your engines (which for a toroidal gravity deck simply means reducing its rotating to zero rather than folding it up too) you can avoid these problems, but if you want to combine both forces (eg. because you have low thrust and you run your engines for a long time) you'll want to have intermediate, angled positions so that your artificial gravity vector always seems to point down.
Here's a nice example of acceleration due to gravity (indistinguishable from thrust due to your engines) combined with centrifugal forces:

(also demonstrating that we are capable of making suitable bearings capable of withstanding forces as strong as 1G of thrust, though making it work for years in a vacuum is left as an exercise for the reader). Note the outward movement, with hinge points at the top of each tether. Youtube link for a slightly more exciting ride.
Take home message: a torus is great if you're not accelerating, that's why they appear on lots of habitat designs. A starship will necessarily have long periods of acceleration, which makes its use inconvenient during those times. The ratio of thrusting to coasting will inform your design. A torus might be best for very long coasting flights.
Or is there actually a limit to how fast these ships should travel to safely navigate in a stable manner in interstellar space?
Your speed limits in space have little to do with your artificial gravity.
Firstly, you're very sharply limited by your drive technology. For a rocket, even a super high tech beam core antimatter rocket like the Venture Star, you are limited by your exhaust velocity. For a beam core antimatter rocket, that exhaust velocity is about 30% of the speed of light (for reasons I'm not entirely certain about; the pions coming out of an annihilation reaction are travelling at .94c, but the quoted pratical exhaust velocity figures for beam core rocket designs are lower than that, eg have a look at the Robert Frisbee's excellent paper How to build and antimatter rocket for interstallar missions which goes into some detail on the issues and performance of antimatter-driven rockets).
Your ship's delta-V, $\Delta V$, is the maximum change in velocity it can perform. If you carry all your fuel and reaction mass with you and travel at modest speeds, this is constrained by $\Delta V = V_e \log_e(R)$ where $V_e$ is your exhaust velocity, $\log_e$ is the natural logarithm function and $R$ is the ratio of the fully-fuelled mass of your ship to the unfuelled mass.
Important Note
By "modest" I mean "not too close to lighspeed". As your Lorentz factor creeps up (and by .6c it is a non-ignorable 1.25) the less you can make use of simple equations (as Hypnosifl helpfully pointed out). Similarly, when you're using a rocket where a substantial amount of the stuff going out the back simply turns into photons and departs unhelpfully, you can't just use the regular equation. The highly complex and ugly relativistic antimatter rocket equation is available in Frisbee's paper, if you're feeling brave. For simplicity, and to give you a rough idea of what you're up against, I'm ignoring it. Just remember that the numbers I'm giving below are hugely over-optimistic, and real figures will be much, much worse.
Now, that said: If you want your $\Delta V$ to equal your $V_e$, you need a mass ratio of $e$ (or about 2.72). To add anothe $V_e$ metres per second to your $\Delta V$, you need to multiply your mass ratio by $e$. If you want to get to 60% of lightspeed and your $V_e$ is 30% of lightspeed, you need a mass ratio of about $e^2$ or 7.4. To get back down from .6c to 0 again, you'll need a total $\Delta V$ of 1.2c and hence a mass ratio of $e^4$ or 55. For a 10000 tonne starship, that means you need more than a quarter of a million tonnes of antimatter on board, and good luck with that.
Take home message: Rockets are terrible for interstellar travel. There's a good reason the Venture Star used a laser sail for boosting (though I prefer sailbeam designs). You should probably use a magnetic braking sail too.
The second issue is shielding. You'll note that the Venture Star diagram you've shared has a massive stack of plates marked "debris shielding". I won't go into the details of shielding a ship trucking along at a decent percentage of the speed of light, but it is hard and the damage it will take will be punishing. Lose that shielding and you're dead. Your speed is therefore limited by how much shielding you can get your engines to push. Centrifuges won't come into it.
Oh, and a third potential issue:
safely navigate
Navigating starships is tricky, because working out which way you have to go isn't entirely trivial. Once you've solved that issue, you light up your big rocket (or other boost mechanism) and away you go... you don't need to do much steering on the way.
In the case of spun sections, that's very important. Off-axis accelerations, such as those caused by rotating the ship, will have all sorts of very unpleasant effects and will definitely put large and uneven loads on your bearings. Turn off the rotation before turning!
(also, partial turns at high speeds are a terrible idea, because you'll get high-velocity crud slipping past your shield and wrecking your ship. don't turn at high speeds. interstellar flying should be in straight lines.)
If so, how can it affect the Coriolis effect on-board if it were to travel that fast and the physical problems it would cause for the ship.
Important note: the artificial gravity is provided by the centrifugal force, not by the coriolis force. The former affects all objects in a rotating frame, the latter only affects objects which have a velocity vector relative to that frame (eg. a person walking around in your hab section, or a dropped object, etc).
So long as you're thrusting along the rotation axis of your gravity decks, and so long as your bearings are strong enough to withstand the force of the engine thrust and the stresses of your rotating sections, you'll be just fine.