A la this image I made.
The "how" (nuclear pulse drive "adjusting" Eros's orbit at its aphelion) and "why" (uber-sized Rods from God) isn't particularly relevant — all that's relevant is that 433 Eros's perihelion has been changed from 1.1334 AU to 1 AU, and its orbit just happens to intersect with the Moon at that perihelion. Oh, and none of this is to scale, but the angles in it (especially the first image) are intended to be accurate.
Anyway, in order to calculate the presumably-unfortunate effects of this on the Earth, I've done some math (see the bottom of this post). However, as I can't find hard data which would let me make fairly accurate guesses, I'm currently making the following assumptions:
- 10% of the energy released by the impact is converted into ejecta velocity; the remaining 90% is absorbed by the Moon.
- 10% of the ejecta energy is in ejecta that escapes the Moon at 2,380 m/s or more; the remaining 90% falls back into the Moon. The Moon's escape velocity of 2,380 m/s provides a benchmark for determining the mass escaping the Moon; 10% of the energy dumped into flinging rocks out of the Moon will fling them fast enough to escape the Moon's gravity. All the rocks flung by that 1% of the impact energy will be going ≥ 2,380 m/s, letting me determine the maximum mass escaping the Moon.
- 25% of the ejecta escaping the Moon falls into the Earth over time, and 25% of it will fall into the Moon over time, with the remainder coming back under the gravitational influence of the Moon. This relies on the next assumption...
- ...that Eros hitting the Moon tail-on at a right angle relative to its surface means none of its fairly impressive velocity will carry over into propelling ejecta, meaning none of the ejecta the impact produces will be going quickly enough to escape the Moon/Earth system entirely. Sure, there's Newton's Third Law, but much of the "equal and opposite" reaction to Eros's impact/disintegration will go into crushing/compressing lunar rock, not throwing it away from the Moon. Also, crushed rock can be crushed further or pushed out of the way to crush more rock, whereas rock that's been thrown away can't have any more energy dumped into it.
- Of the 50% of the ejecta that escapes the Moon, ends up under its gravitational influence again, but does not directly fall into it, the Moon will act as a shepard moon towards it, slingshot half of it (the ejecta orbiting slightly inside the Moon's orbital path) out of the Earth/Moon system, and slingshot the other half of it (the ejecta orbiting slightly outside the Moon's orbital path) into the Earth.
- 10% of the ejecta pulled into the Earth actually makes it through the atmosphere to impact; the rest is too small to make it to the surface and disintegrates in the atmosphere.
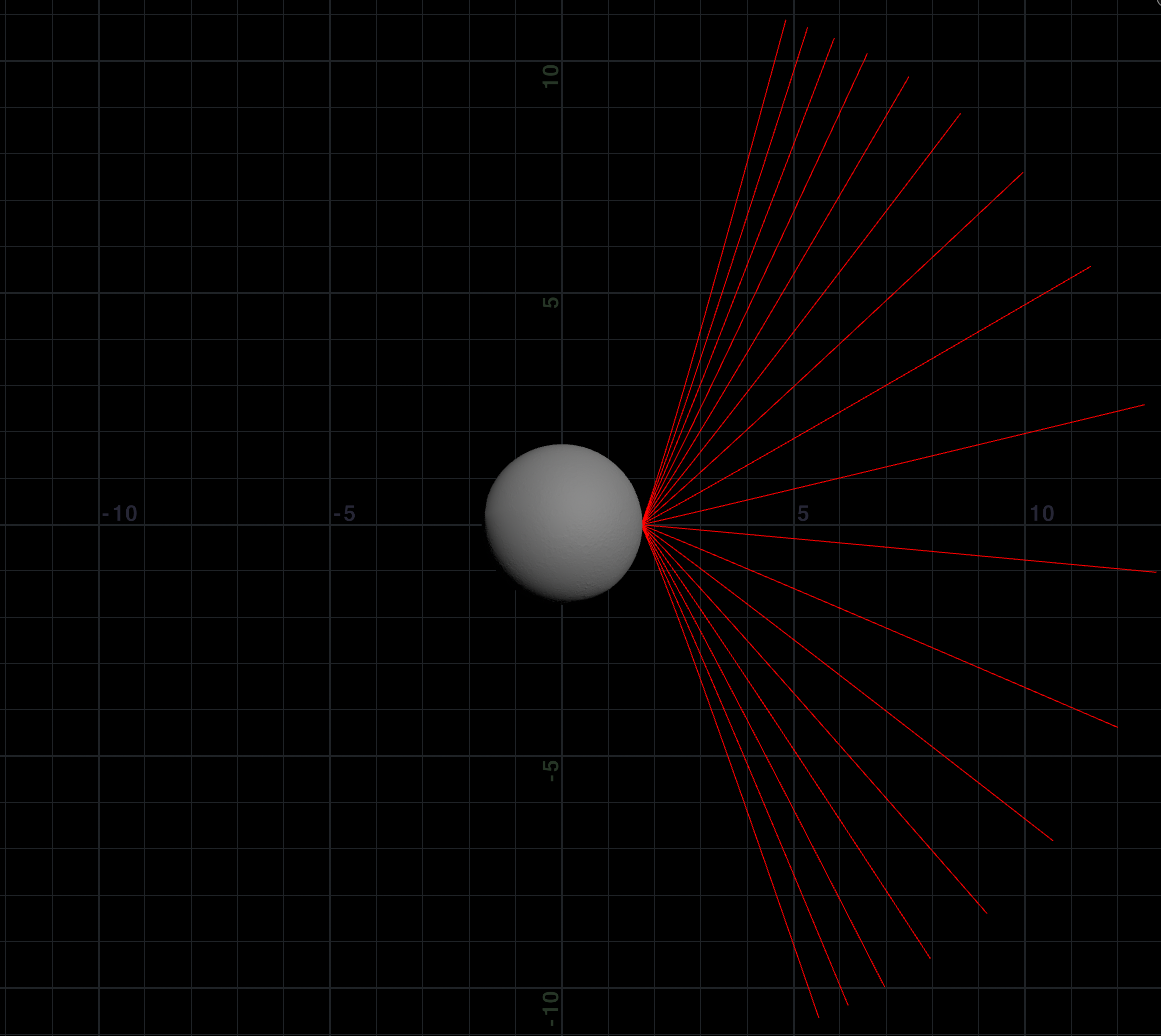
Basically, if we look at the image below (which I got from the 9th page of this), assume the asteroid represents the Moon, and assume the arrow represents the trajectory of a piece of ejecta...

90% of the impact's energy is in that little explosion and doesn't go anywhere.
9% (90% of 10%) of the impact's energy propels Class I ejecta.
0.25% (25% of 10% of 10%) of the impact's energy propels Class II ejecta.
0.25% (25% of 10% of 10%) of the impact's energy propels Class IV ejecta that gets slingshotted out of the Earth/Moon system.
0.25% (25% of 10% of 10%) of the impact's energy propels Class IV ejecta that gets slingshotted into the Earth.
0.25% (25% of 10% of 10%) of the impact's energy propels Class V ejecta that reaches the Earth directly.
In regards to the Moon, Class III doesn't exist; any ejecta that ends up in Class III will, over time, get pulled out of orbit and crashed into the Moon by lunar mascons, essentially putting it in Class II.
Or, for another visual, this image:
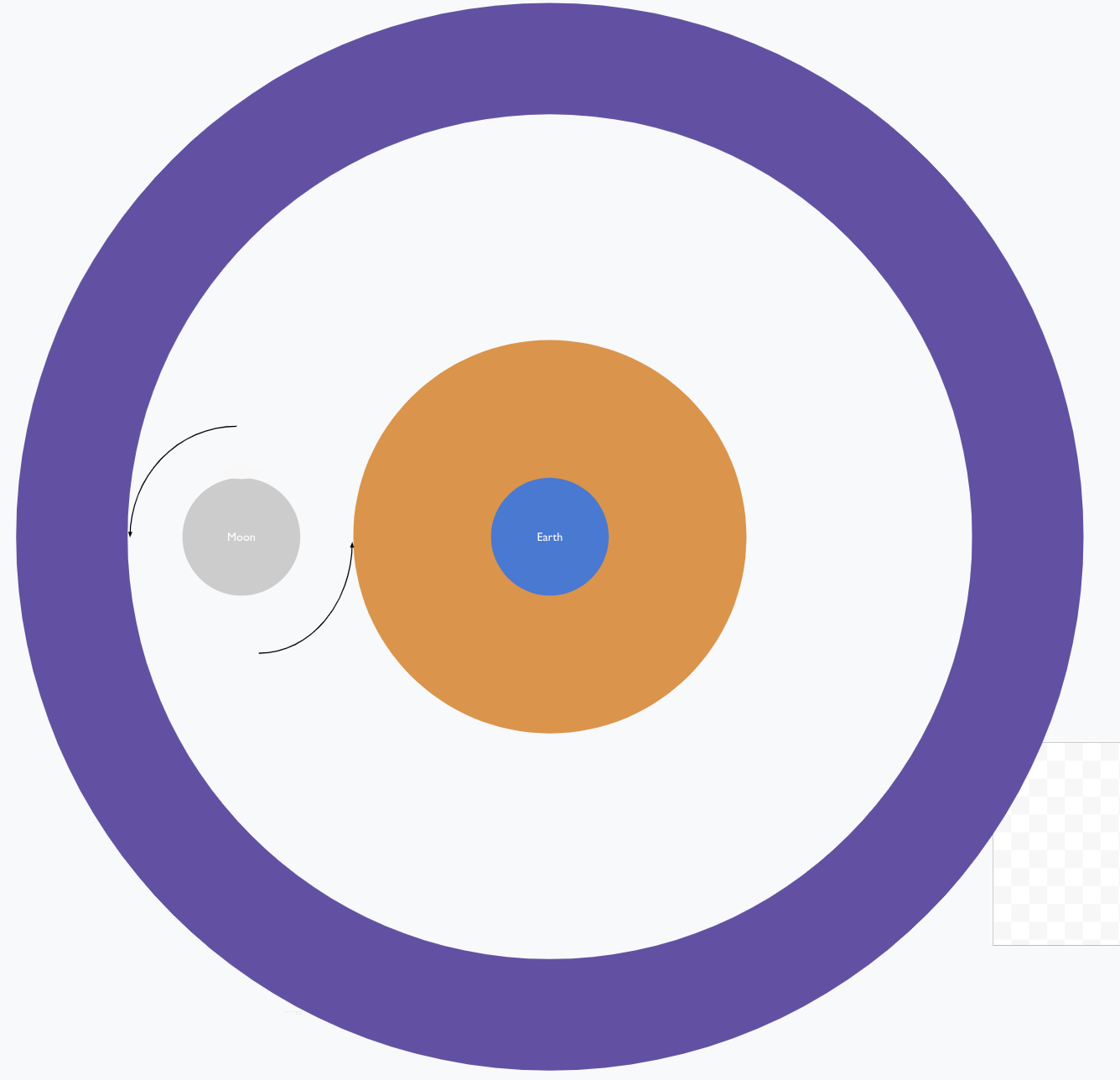
This is a "top-down"/"birds-eye" view of the Earth/Moon system; the Moon's orbit takes it towards the bottom of the page, and the entire thing is moving to the left as it orbits the Sun.
If ejecta is in the blue section, it's orbiting faster and at a lower altitude than the Moon, so it'll either fall into the Earth or "outrun" the Moon over time, eventually catch up to it again, and then slingshotted around the "bottom" of the Moon and out of the Earth/Moon system.
If ejecta is in the red section, it's orbiting slower and at a greater altitude than the Moon, so the Moon will "outrun it" over time, meaning the ejecta will either fall into the Earth or have the Moon eventually catch up to it again, slingshotting it around the "top" of the Moon and into the Earth.
If ejecta is in the green section, it's not going fast enough to escape the Moon's gravity, so it'll fall back into the Moon.
Yet another image, from the same perspective:
The Moon acts as a shepard moon; any particle orbiting to the left of or behind the Moon in the same direction gets thrown into the purple outer ring, whereas any particle orbiting to the right of or in front of the Moon in the same direction gets thrown into the orange inner ring, and likely within the Earth's Roche limit for particles of that size, meaning tidal forces will eventually tug the particle into the Earth.
Why am I asking this question?
Simple: I need to make better assumptions. Remember all those percentages? I need to know whether, for instance, assuming the Moon will absorb 90% of the impact energy is accurate or not, or whether or not the Earth will actually be hit with half of what escapes from the Moon.
To reiterate — I'm currently assuming that:
10% of the energy released by the impact is converted into ejecta velocity
10% of the ejecta energy is in ejecta that escapes the Moon
50% of the ejecta escaping the Moon hits the Earth
90% of the ejecta hitting the Earth burns up and becomes airborne particulates; the remaining 10% physically hits the Earth's surface
Are these accurate assumptions to make in regards to modeling an impact between the Moon and 433 Eros?
Anyway, here's that math I mentioned:
6.687 quadrillion kg 433 Eros impact w/Moon @ 1 AU from Sun, prograde Moon velocity
- 34,148 m/s velocity relative to Sun - 1,022 m/s Moon orbital velocity = 33,126 m/s collision.
- (((33,126 m/s)^2) x 6.687 x (10^15) kg)/2 = 3.66892913 x (10^24) J impact energy, 3.66892913 x (10^23) J net ejecta energy, and 3.66892913 x (10^22) J of energy in the ejecta escaping the Moon.
- ((((3.66892913 x (10^22) J) x 2) / ((2380 m/s)^2)) = 1.29543434 x (10^16) kg total ejecta escaping the Moon, of which...
- ...3.2385859 x (10^15) kg falls back into the Moon, 3.2385859 x (10^15) kg is flung out of the Earth/Moon system by the Moon, 3.2385859 x (10^15) kg is flung into the Earth by the Moon, and 3.2385859 x (10^15) kg falls directly to the Earth by itself.
- Of the 6.4771718 x (10^15) kg of ejecta hitting the Earth, 5.8294546 x (10^15) kg burns up into atmospheric dust and 6.4771718 x (10^14) kg physically impacts the Earth's surface.
It would be a lot worse if Eros hit the Moon head-on as the Earth was coming around the Sun at a right angle to its trajectory. Conversely, it'd be a lot less dangerous if it came in in the same direction Earth was orbiting in. As a compromise, I had Eros broadside the Earth/Moon system halfway between those two extremes.
Also, note that these calculations don't include the velocity incurred by Eros accelerating as the Moon's gravity pulls on it, nor the velocity incurred by the nuclear pulse drive attached to it firing off its last H-bombs to add some extra ΔV right before impact for maximum energy release. They're also fairly inaccurate in that they try to divide the types of ejecta trajectories into a few neat little categories rather than the mess that n≥3 orbital physics usually are, as well as because they portray 2,380 m/s as the velocity of all the ejecta escaping the Moon (some would go that speed, much would go faster, meaning that 1.29543434 x (10^16) kg is probably too much). However, these are the bare minimum; I'm basically going for a Fermi estimate here.