I asked a similar question (in a SpaceEx manner) on Space Exploration, but here I've made it a bit more ... worldbuilding.
We have a nice Space Elevator, and I'm in charge of the luxury guest accommodation for the six-day round trip.
Setting aside the design considerations, I am interested in what the experience would be physically.
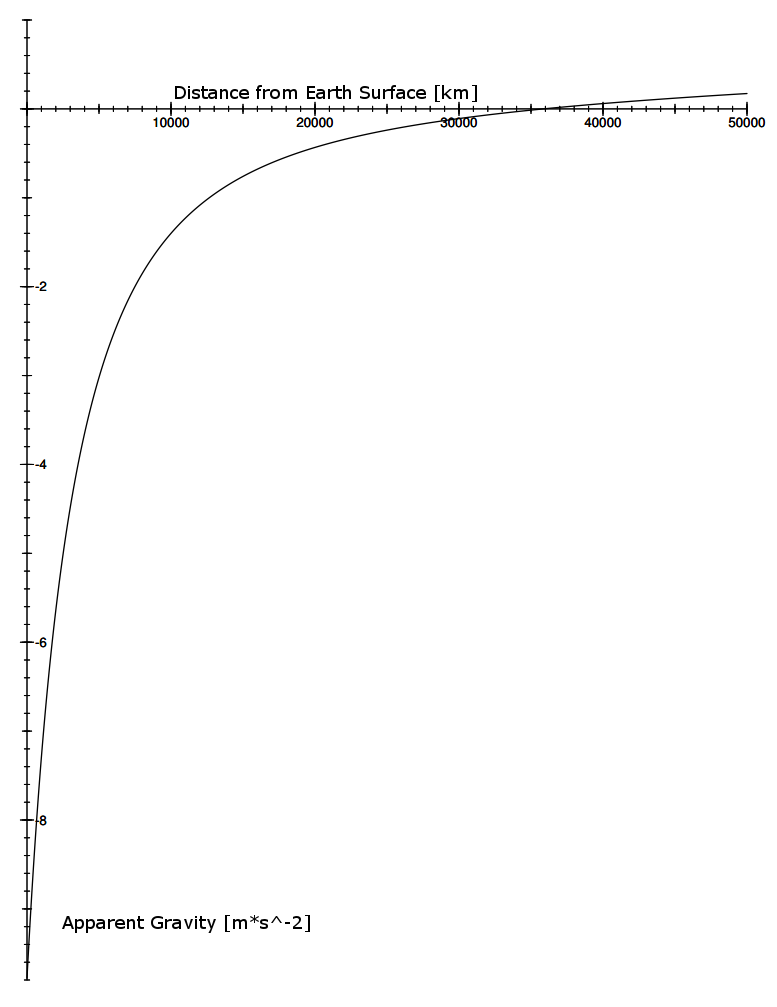
Standard conceptual 50,000km space elevator attached to a counterweight; accelerating at low altitudes, then a constant velocity, and then a deceleration.
What effects will my guests feel (1) accelerating upwards at low altitudes, (2) constant velocity at very high altitudes, (3) deceleration, but 'looking up' at the Earth, and (4) stationary on the counterweight.
No numbers needed, just "then they fall to the ceiling..." You see, I have to design the suite right, with seatbelts, beds, showers, etc. for the most discerning guest!