13
$\begingroup$

I'd like to travel really really fast, and I've got some scientists proposing a novel new way of doing so.

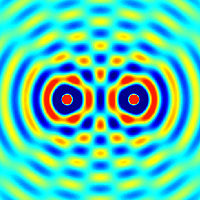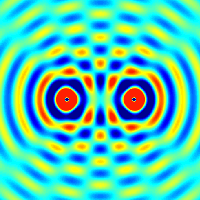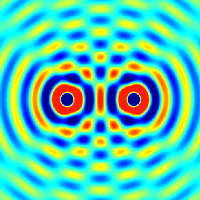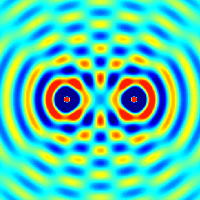
They've developed the technology to generate extremely powerful controlled gravitational waves. Based on my knowledge of these things, I understand that they propagate at the speed of light as a ripple in the time-space continuum. My scientists tell me that I can ride in a patch of distorted space in which I don't actually need to exceed $c$ locally in order to effectively travel faster than the speed of light relative to a destination. Of course, a single gravitational wave travels the speed of light, so I know I can't go any faster by riding one of those, but my scientists are proposing that I ride the moving interference pattern between two sets of waves, since a local maxima caused by wave interference can effectively move significantly faster than $c$, based on the angle of the intersecting wave patterns.

enter image description here

If we image the blue sections of this image to be peaks in which the fabric of space is streched, we can travel in one of the blue bubbles, which should "move" faster than c, since they don't represent an actual moving wave, but rather the intersection point between two waves.

The effective propagation speed of one of the intersection points is based on the angle at which the two waves intersect. Specifically, propagation speed s can be given by the equation $s=u/\sin{\theta}$, where $u$ is the speed of the wave front and $\theta$ is the half-angle between two otherwise symmetric waves.

My scientists tell me that, if we line a potential space lane with gravitational wave emitters, we can create a route that can be traveled at what are effectively superluminal velocities. However, they're asking me for a very large sum of money to do this. Should I fund their project or rescind their grant money and feed them to my pet sharks?

Improve this question
$\endgroup$
7
  • 5
    $\begingroup$ It sounds a lot like an Alcubierre drive. $\endgroup$
    – HDE 226868 ♦
    Commented Feb 17, 2016 at 0:26
  • 1
    $\begingroup$ This stuff about gravitational waves bothers me. I mean, we're essentiallY saying we detected some kind of energy wave from two celestial bodies crashing into each other, so going off of Einstein's theory we detected a gravitational wave. And if we detected a gravitational wave, then Einstein's theory is correct. So it was definitely a warp in space and time, even though we cannot see time. $\endgroup$
    – Xandar The Zenon
    Commented Feb 17, 2016 at 4:03
  • 1
    $\begingroup$ Struggling to visualise this. My first attempt had a ship travelling subluminally in compressed space that followed a wave at light speed; but that's not faster than light. Second attempt; travelling subluminally through compressed space in a standing wave between two gravitational waves; carefully timed to cross from compressed space to compressed space at the moments the waveform inverts, where your speed would be almost arbitrary but set, fundamentally, by the wavelength of the gravitational waves. Is that second thing what you mean? $\endgroup$
    – sh1
    Commented Feb 17, 2016 at 5:26
  • $\begingroup$ @sh1 Second attempt is sopt on. Added the words "standing wave" to my question since that's exactly what I meant. $\endgroup$
    – ckersch
    Commented Feb 17, 2016 at 14:37
  • $\begingroup$ @HDE226868 This idea was inspired exactly bu Alcubierre drives. I was reading about them and wondered if it would be possible to make something like one using a standing wave pattern between two sources of gravity waves. $\endgroup$
    – ckersch
    Commented Feb 17, 2016 at 14:52

6 Answers 6

Reset to default
16
$\begingroup$

No can do.

I was able to find the answer here, written by LIGO scientist Dr. Amber Stuver:

How valid is the wave-like-in-water analogy? Can we “surf” these waves? Are there gravity “peaks” like there are “wells”?

Stuver: Because gravitational waves can travel through matter unchanged, there isn’t a way to surf them or use them for another kind of propulsion. So no gravitational-wave surfing.

The “peaks” and “wells” is an excellent point. Gravity is always attractive because there is no negative mass. We don’t know why but it has never been observed in a lab or any evidence found elsewhere in the universe. So gravity is usually represented on spacetime graphics as being a downward curvature, or your “well.” A mass traveling by the “well” will tend to bend inward toward it; this is gravitational attraction. If you had negative mass, you would have repulsion, which would be represented by a “peak.” A mass moving by a “peak” would tend to bend away from it. So there are“wells” but no “peaks.”

The water analogy is very good at talking about how the strength of the wave decreases as it travels away from its source. A water wave will get smaller and smaller just like a gravitational wave will get weaker and weaker.

Slightly simplified, this means that you can't use gravitational waves for propulsion because they don't transfer energy quite in the same way that water waves do. The analogy breaks down further because gravitational waves are plane waves, not sinusoidal waves - so you shouldn't try to visualize them as being anything like water waves.

Improve this answer
$\endgroup$
9
  • 6
    $\begingroup$ I always love it when scientists are willing to say "We don't know why ____ has never been observed in a lab, or any evidence found in nature, so we typically do {insert simplification here}." Its nice when they admit they're a little bothered why something is so convenient. $\endgroup$
    – Cort Ammon
    Commented Feb 17, 2016 at 1:51
  • $\begingroup$ How does the presence of a gravity well affect the effective distance between two points, relative to the distance between two points in a vacuum? Will it always be greater than the effective vacuum distance between two points, given gravity waves created by a positive mass? $\endgroup$
    – ckersch
    Commented Feb 17, 2016 at 14:51
  • $\begingroup$ @ckersch It alternates between stretching them together and stretching them apart. $\endgroup$
    – HDE 226868 ♦
    Commented Feb 17, 2016 at 16:05
  • $\begingroup$ If there exist 'peaks' where the distance between points are stretched apart, wouldn't it be possible to travel in the peaks to move faster than light speed in undisturbed space? $\endgroup$
    – ckersch
    Commented Feb 18, 2016 at 15:26
  • $\begingroup$ @ckersch I don't think so, because the spacecraft wouldn't be nudged in any specific direction, AFAIK. $\endgroup$
    – HDE 226868 ♦
    Commented Feb 18, 2016 at 15:58
4
$\begingroup$

No, you can't "surf" it because you're not attracted or repelled by the boundary.

Feed them to the sharks. This is pseudoscience and building such emitters would require more energy than the sun uses, and transfer very little of it to any other objects.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ "Because you'd not attracted or repelled by the boundary" - This would still be the case for photons, but radiation pressure is a good propulsion mechanism nonetheless, even for neutral objects. $\endgroup$
    – HDE 226868 ♦
    Commented Feb 17, 2016 at 0:11
  • $\begingroup$ My thought was not to "surf" in manner of using the wave for propulsion, but rather to travel via other means at a high superluminal velocity in a patch of distorted space in a standing wave where distances are effectively shorter. And yes, energy consumption will be in solar masses/hr. $\endgroup$
    – ckersch
    Commented Feb 17, 2016 at 14:42
  • $\begingroup$ You might want to clarify the question, then. Without a way to reflect, standing waves would not be possible. $\endgroup$
    – JDługosz
    Commented Feb 17, 2016 at 15:04
2
$\begingroup$

It might be possible to distort spacetime along your path with massive gravity spikes, not just a few waves. However, even if you did, you would have to wait a period of time proportional to the time it takes light to travel for the gravity wave/spikes to decrease the path length between you and your destination. Of course, the effect of trying to do so would likely disrupt the entire fabric of the universe as the equivalent of a gravity EMP propagates outward from their emitters.

I think the idea may be similar to that of The Speed of Darkness, and similar limits apply, other than the unique ability to distort spacetime. Of course, you are talking less about a nice calm ripple of gravity and more of a "Hulk is angry that the can of spam doesn't open" kind of gravity wave. Sure, the can is going to get opened, but it is less clear if any of the soft squishy meat on the inside will survive the encounter.

Improve this answer
$\endgroup$
0
$\begingroup$

The answer is to produce the same but opposite direction wave to produce thrust. The thrust will be produced due to compression of the fabric of space-time. Gravity waves can come in different forms. One from binary, and one from a rapid expansion or contraction of matter.

The only waves I conceive will provide thrust are the explosive type, because of it's contraction in 2d shell contraction expansion configuration.

Imagine a bomb goes off, then imagine the compression wave just passes a second bomb when the second one goes off. The second bomb is forced toward the first explosion. Now change them from bombs, to gravity pulse devices. The second one isn't forced away only warped until it activates, and that opposing force generated propels the two attached pulse devices, or array of...

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Your proposed way of generating waves doesn’t seem to overcome the issue I noted in an earlier answer, that they cannot be “surfed”. «Gravity waves [sic] can come in different forms. One from binary, and one from a rapid expansion or contraction of matter.» that doesn’t make sense. $\endgroup$
    – JDługosz
    Commented Oct 31, 2016 at 6:04
-1
$\begingroup$

I suggest you keep your money and wait for the publication of NASA research that has already been funded, performed, passed peer review and is due to be published at the end of this year.

For if you're not familiar with the terms, what you're describing sounds a hell of lot like the Alcubierre drive. The research to be published is about verifying a suspected warp bubble generated by the EmDrive as explained here.

Improve this answer
$\endgroup$
4
  • $\begingroup$ See here regarding the EmDrive. Warp bubble is just so much nonsense: check the edit history for cranks passing through, and note the “citation needed”. $\endgroup$
    – JDługosz
    Commented Oct 31, 2016 at 3:23
  • $\begingroup$ That's why I'm advising against investing and instead saying to wait for the soon-to-be-published already-performed research that will probably/hopefully provide substantial information regarding the feasibility. After all, why fund some snake-oil scientists when there's real science soon available for zero cost? $\endgroup$
    – Vaesper
    Commented Oct 31, 2016 at 3:35
  • 1
    $\begingroup$ This does not provide an answer to the OP’s question. A pointer to Alcubierre Warp was already noted as the very first comment to the Question. The emDrive, if it were relevent, should also be noted as a comment to the original post, not an Answer. $\endgroup$
    – JDługosz
    Commented Oct 31, 2016 at 6:01
  • $\begingroup$ @JDługosz why should it be a comment? My answer is a "No, for this specific reason", to the OP's question "Should I fund it or not?". $\endgroup$
    – Vaesper
    Commented Oct 31, 2016 at 8:24
-1
$\begingroup$

You don't need to be riding it...you just need to be in the force field of the wave/distorted SPACE. This force field will do the work opposite to the motion itself.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .