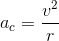12
$\begingroup$

My habitat ring is constructed in orbit around a planet with disconnected sections: (not to scale, obviously)

enter image description here

The sections are connected by cables and winched together.

As the diameter gets smaller, the ring should spin faster.

enter image description here

Does this give my ring artificial gravity?

Improve this question
$\endgroup$
10
  • 1
    $\begingroup$ Assuming you're starting with a low-g habitat: Well, as your sections are winched together they are ALSO getting closer to the planet, so their faster spin would be counteracted by a stronger pull from the planet, netting out to zero or near zero change in gravity. (IANAPhysicist, this is just my best guess.) $\endgroup$
    – Isaac Kotlicky
    Commented Jul 10, 2017 at 16:07
  • 1
    $\begingroup$ artificial-gravity.com/sw/SpinCalc might be helpful. Especially if that middle thing is not a planet. $\endgroup$
    – Alonzo Muncy
    Commented Jul 10, 2017 at 16:09
  • 3
    $\begingroup$ The Ringworld is unstable! $\endgroup$
    – Mark
    Commented Jul 10, 2017 at 21:14
  • 1
    $\begingroup$ What's the point though; having gravity for about thirty seconds? Also, wouldn't it just by spinning, create some? $\endgroup$
    – Mazura
    Commented Jul 11, 2017 at 0:16
  • 3
    $\begingroup$ Yes, it would. But mechanical stress on cables would be enormous - about weight of half of the ring multiplied on acceleration. It would work if structure is more complex - internal ring spins fast and supports slower external ring that has gravity. $\endgroup$
    – Vashu
    Commented Jul 11, 2017 at 2:24

4 Answers 4

Reset to default
15
$\begingroup$

Let's start off looking at this from a conservation of angular momentum point of view. We can say that angular momentum is definitely conserved here because there is no external torque being applied to the system.

I'm not sure that orbital energy is conserved here since it really seems like whatever motors are pulling the cables tighter will need to do work to do that which allows energy to be transferred from batteries/solar into orbital energy (so total energy is obviously conserved it's just that we'd also have to account for other energy sources besides orbital energy).

Let's say the station starts off orbiting at a radius of $R_0$ in a "neutral" circular orbit (i.e. station occupants feel weightless). This gives us an orbital velocity of $v_0 = \sqrt{\frac{\mu}{R_0}}$ where $\mu$ is the gravitational parameter of the central body.

Our specific angular momentum is then just:

$R_0 v_0 = \sqrt{R_0 \mu}$

Now, let's say you reel in on those cables and bring the whole radius down to $R_f$. Since angular momentum is conserved we have $R_0 v_0 = \sqrt{R_0 \mu} = R_f v_f$. We can solve this for $v_f$:

$v_f = \frac{\sqrt{R_0\mu}}{R_f} = \sqrt{\frac{R_0\mu}{R_f^2}}$

To answer your original question we need to determine if this is slower or faster than the circular orbit velocity at $R_f$:

$v_{f,circular} = \sqrt{\frac{\mu}{R_f}}$

If we rearrange our expression for $v_f$ we get:

$v_f = \sqrt{\frac{R_0}{R_f}} \sqrt{\frac{\mu}{R_f}}$

Combining this with our equation for the circular velocity:

$v_f = \left(\sqrt{\frac{R_0}{R_f}}\right) v_{f,circular}$

So, if $R_0 > R_f$, the station ends up with a velocity that's greater than orbital velocity (since $\sqrt{\frac{R_0}{R_f}} > 1$). This is enough to show that you would in fact create artificial gravity by doing this.

Improve this answer
$\endgroup$
3
  • 3
    $\begingroup$ Well I prefer this answer because it makes me feel like an idiot savant! :D $\endgroup$
    – wetcircuit
    Commented Jul 10, 2017 at 21:45
  • $\begingroup$ If I interpret Kyle's answer above correctly (and it seems logical), the inner surface of each orbiting station that is farthest away from the planet would "feel" the artificial gravity. This would seem to explain why it is hard for the spinning skater to hold the tighter, faster spin (due to the force radially outwards). $\endgroup$
    – Jack R. Woods
    Commented Jul 13, 2017 at 17:52
  • $\begingroup$ TL;DR: "Yes, if the station ends up with a velocity that's greater than orbital velocity." Correct? Which, if this thing is in orbit, a decrease in its radius WILL increase its angular velocity beyond that orbital velocity - no math needed... ? (just put the penultimate sentence at the top please ;) $\endgroup$
    – Mazura
    Commented Jul 21, 2017 at 4:56
2
$\begingroup$

Yes. A decrease in rotation radius will increase the apparent gravity.

Your entire station is orbiting a planet together. The habitat ring is spinning around the station, not orbiting it. (I think some of the other answers may have misinterpreted that.)

In order to keep the habitat stations spinning properly, each one has to have a cable connecting it to the center station. They could also be linked to each other, but that would require thicker cables, and make orbital station keeping difficult for the whole assembly.

The centripetal acceleration of a body rotating with linear speed, v, and radius, r, is r, is $a_c. Towing the rotating stations closer would decrease the radius while keeping the linear velocity constant. This would increase the apparent gravity.

Note that the linear velocity is constant but the angular velocity increases.

Proof that linear speed does not decrease as radius decreases.

The individual stations are towed towards the station, perpendicular to their velocity. Because the force and velocity are perpendicular there is no change in energy with the towing; work is the dot product of force and direction. A change in linear speed requires a change in energy, because E=0.5*m*v^2.

Improve this answer
$\endgroup$
10
  • $\begingroup$ v will decrease as r decrease and the closer it is, the lower the gravity would be in your situation $\endgroup$
    – A. C. A. C.
    Commented Jul 10, 2017 at 18:16
  • $\begingroup$ @A.C.A.C. Would you like to provide some of your reasoning for that? $\endgroup$
    – BobTheAverage
    Commented Jul 10, 2017 at 18:30
  • $\begingroup$ If your rotation rate stays the same (eg 1 rotation per hour), then the bigger your radius, the bigger the circumference you trace. The circumference directly proportional to the radius in a circle ( c = 2 * pi * r). In 1 rotation, a smaller radius will mean smaller circumference means smaller velocity. In the end it boils down to a_c = (r^2/r) * constant scalar. which mean acceleration scales linearly with radius positively. $\endgroup$
    – A. C. A. C.
    Commented Jul 10, 2017 at 18:41
  • 1
    $\begingroup$ Let us continue this discussion in chat. $\endgroup$
    – BobTheAverage
    Commented Jul 10, 2017 at 19:08
  • 1
    $\begingroup$ @A.C.A.C. Angular momentum is not a form of energy. Potential energy, however, is. $\endgroup$
    – Karl
    Commented Jul 10, 2017 at 20:25
0
$\begingroup$

The rotation rate increases and you could stand on the edge (head toward Earth, feet away). Since the components of your ring are no longer moving at circular orbital speed, the ring is unstable, and if some small force moves it off center, it will continue to go further off-center until one side of it starts to enter the atmosphere. It will need some sort of active stabilization -- small rocket thrusters or the like in order to correct any drift.

This is the same situation as Niven's "Ringworld" though on a smaller scale.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Comments are not for extended discussion; this conversation has been moved to chat. $\endgroup$
    – Monica Cellio
    Commented Jul 14, 2017 at 3:52
-1
$\begingroup$

The ring will not generate any artificial gravity no matter what orbit you are at because the orbit is achieved when the angular momentum balances the gravity of the object you orbit. Which is why there is no artificial gravity on our space stations orbiting the earth. You can think your contraption is the same as a bunch of ISS attached together and no matter how you go about that, none of them will have artificial gravity from just orbiting.

If you were to make the stations travel faster than they would in normal orbit and let the cables between them stabilize them instead, then you would achieve artificial gravity. The force the cables are able to stretch is the force of your artificial gravity.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Wouldn't the stations travel faster as the ring diameter decreases? $\endgroup$
    – wetcircuit
    Commented Jul 10, 2017 at 16:23
  • 6
    $\begingroup$ Re-reading your answer, I think perhaps you have misunderstood my question. $\endgroup$
    – wetcircuit
    Commented Jul 10, 2017 at 16:38
  • 5
    $\begingroup$ The rings must travel faster than the orbital speed to get gravity. $\endgroup$
    – A. C. A. C.
    Commented Jul 10, 2017 at 16:52
  • 2
    $\begingroup$ This answer and your comments below my answer demonstrate that you do not understand the basic physics of rotating bodies. $\endgroup$
    – BobTheAverage
    Commented Jul 10, 2017 at 20:01
  • 1
    $\begingroup$ This answer would be essentially correct if the segments were still orbiting freely — then, we would lower their orbits by removing some energy. But that's not what we're doing here. The bit of the question that makes this answer wrong is "the sections are connected by cables and winched together". Now the tension in the cables provides an extra inward force on the segments in addition to what they would experience if free-falling. Which also means a free-falling object will fall outward towards them — apparent gravity! $\endgroup$
    – hobbs
    Commented Jul 11, 2017 at 8:24

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .