TLDR
You can model the effect of two moons by summing two sine waves. To do this plot each moon as a function of Time and manipulate the gravity of the moon by changing the amplitude and the orbital period by multiplying the time variable.
A final plot showing the summation of the others gives the resulting forces on the tides.
Long Version
In reality calculating tides is VERY complicated, it depends on many factors involving river emptying into the sea, the terrain around the shore, currents, weather etc... However, we can come up with a rough approximation which will at least help us get a feel for how the tides would work.
The first thing to establish is whether the moons would have to have the same orbital period (do they both take either same amount of time to orbit the planet?) the answer is no, Europa has an orbital period of 85 hours and Ganymede of 172 hours. This means each moon can be independent to each other.
Next you need to determine whether both moon are on the orbital plane, I would suggest they are. After all the planets in the solar system orbit on the same plane and it keeps the maths easier.
So you've got two moons of different masses orbiting at different periods. You can represent this very easily as a graph with two sine waves on it. The high tides on any given day are the cumulative force from the moons.
For the sake of argument let's say moon A has a force of 10 and a period of 10 days, moon B has a force of 15 and a period of 15 days. You can plot this for time, now draw a third line which is moon A's force plus moon B's force. This is effectively your tide table. You'll notice that you have low tides and high tides as normal but every so often you have super high and super low tides!
Next all you need to do is decide the maximum and minimum tide heights and scale the graph accordingly. Using this technique you not only get a feel for what the tides would look like but also can calculate down to the day when the high tides will be.
In the example below there are two moons (Green and Red) on the graph, the net impact of these moons is shown in blue. As you can see one moon dominates the tides fairly dramatically (because it's significantly bigger) but the red moon has enough impact to warp the tides a little. I mocked this up with FooPlot:
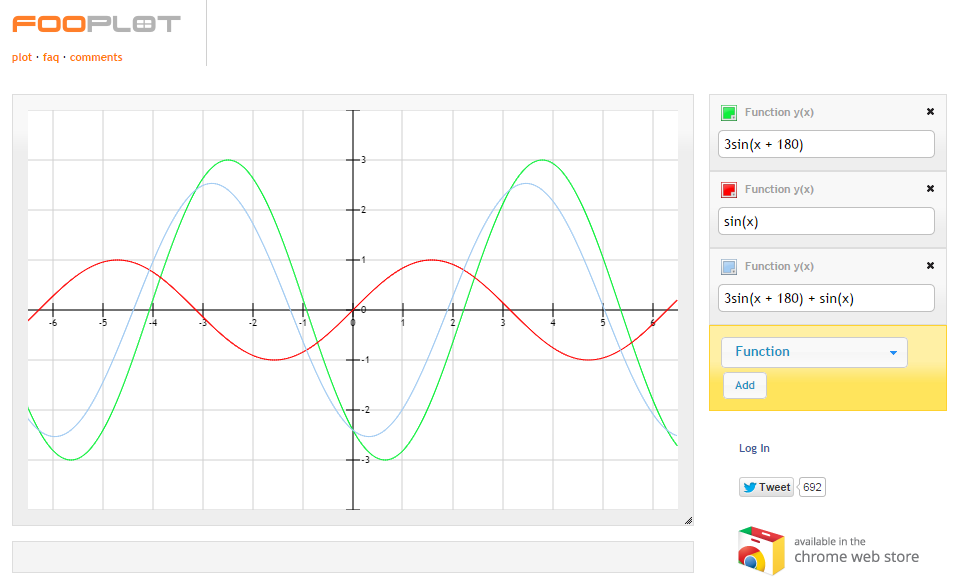
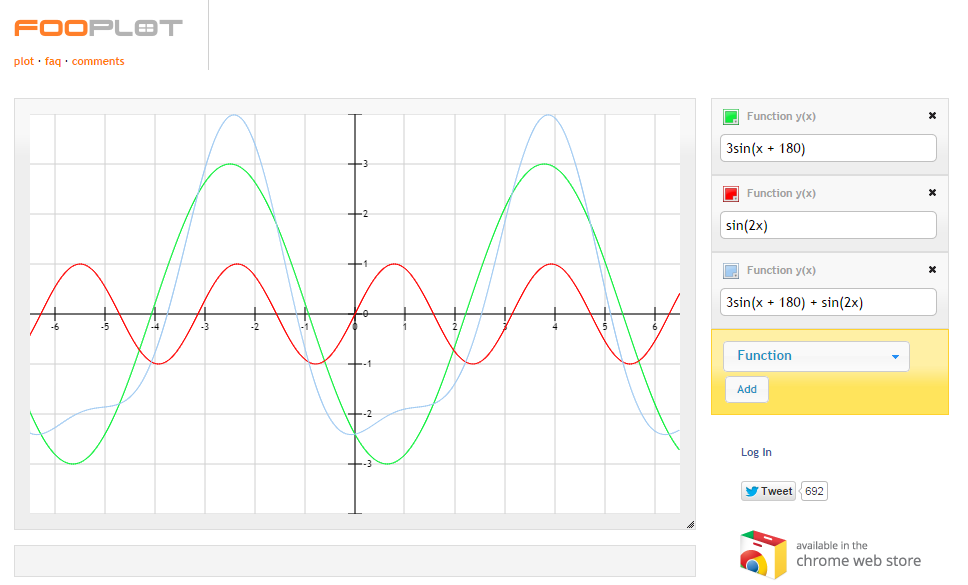
For sheer curiosity I've messed with the orbital period of the red moon — now you can see very dramatic impacts on the tide line (blue). It looks like tides with this lunar configuration have much longer low tides which suddenly rush in.
To add a little more maths for those of us (including me) who haven't studied trigonometry in a while. When messing with waves there are three values you can adjust Frequency, Amplitude and Phase. These represent
- How long it takes for the moon to orbit the planet
- The strength (related to gravity) of the moon's effects on the tide
- Synchronising the moons - slides the tides back and forward so you can line up double full moons and such.
You can plot this like so:
$$
\mathrm{TidalForce} = \mathrm{Amplitude} \times \sin((\mathrm{Frequency} \times T) + \mathrm{Phase})
$$
T in this case is the time from the start of the cycle, as you increase it you get the change in TidalForce. Phase manipulates T=0 and the Full Moon (using FooPlot at least is degrees so 180 is half a lunar cycle).


