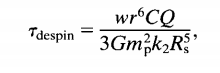3
$\begingroup$

I have a mars-sized "planet" orbiting a superearth-sized mother-planet. There is also another satellite with an abnormal elliptic orbit, but it is of minor concern for now.

This is the resume of their data.

Mother Planet

Radius: 11 633 km

Semi-major Axis: 506 687 988 km

Density: 5.730 g/cm³

Mass: 3.61965E+25 kg

Surface Gravity: 18.3g

Daylength: 32.40 earth hours [provisional]

Satellite Planet

Radius: 3 073 km

Daylength: 32.42 earth hours [?]

Semi-major Axis: 1 113 894 km

Density: 10.373 g/cm³

Mass: 1.26056E+24 kg

Surface Gravity: 8.905g

Revolution: (40d*)

Minor Satellite

Radius: 1 799.17 km

Daylength: ~1600 earth days

Semi-major Axis: 1 592 750.00 (elliptical)

Revolution: ~1600 earth days

As you probably noticed, daylength of the satellite is quite short for a satellite, and the revolution is far longer than the daylength, which is very counter-intuitive for a satellite scenario. At first I wanted to use this third body, the second satellite, as an excuse for gravitational perturbation on the system to speedup rotation whilst slowing down revolution, but this would be quite an assumption and would not look nice from a hard sci-fi perspective.

The general question here is:

Which scenario is the most science-friendly:

a) The third body controls the rotational speed of the binary system.

That being the case, what calculations I should use to get it working this 32h/40d scheme?

The parameters of the second body are irrelevant, it can be shrunk and expanded in size as long as it doesn't get past 2k kilometers radius, its orbit can be altered, etc. I don't have an exact desired setup for it, the only requirement is to be small and icy.

b) The satellite is tidally locked to the planet at 32h/32h.

In this case, a 32h revolution seems abnormally fast for a Mars-sized thing orbiting a two-Earth-sized thing. Would this have any serious side-effects?

And what would be the nature of such absurd speed?

c) It's okay to have the 32h/40d system

If so, how I can explain the nature of the system better? All i can think is a newborn planet, that has just been caught by the central planet and didn't have time to be tidal-locked, but considering that it must be inhabited while still in this configuration, this is pretty unlikely, as life needs billions of years to establish.

Improve this question
$\endgroup$
10
  • 1
    $\begingroup$ I did a significant edit for spelling and clarity. Hopefully I didn't change the author's intent anywhere. $\endgroup$
    – kingledion
    Commented Nov 3, 2016 at 13:57
  • 1
    $\begingroup$ np, everything is in place, I'm quite messy trying to convey my ideas properly, thanks for the edit $\endgroup$
    – Lucas Flicky
    Commented Nov 3, 2016 at 14:01
  • $\begingroup$ You know the orbital period is not arbitrary, but follows from the gravity of the system. So how do you mean to skow it down? $\endgroup$
    – JDługosz
    Commented Nov 3, 2016 at 14:12
  • $\begingroup$ Satellite planet is just a moon. Why not to call it a moon? $\endgroup$
    – Mołot
    Commented Nov 3, 2016 at 14:26
  • $\begingroup$ i aquired this quirk of calling it sattelite planet because the center of perspective is it, not the "mother planet" as usual. but essentially, it is a moon, but from their perspective, the mother planet that seems to be the moon. $\endgroup$
    – Lucas Flicky
    Commented Nov 3, 2016 at 14:55

1 Answer 1

Reset to default
1
$\begingroup$

After some research, I found the equation for obtaining a rough approximation of the time until a planet will get Tidally locked

( Spin Rate * [Semimajor Axis^6] * Rotational Inertia * Sat Dissipation Function ) / ( 3 * Gravitational Constant * [PlanMass^2] * Sat Tidal Love Number * [Sat Radius^6] )

enter image description here

Using some template numbers for info i dont have precisely now, based on a table at the ref. I managed to get that a lock would occur in ~2bi years considering the orbit is near-perfectly circular.

Eliptical orbits tend to resist tidal locking, and bodies with big oceans also tend to resist being tidally-locked, for that reason, i may in future shrink one of the axis of rotation to make it less prone to tidal lock. The fact that the mother-planet is not so dense, and that the moon planet is quite far away from it also helps.

Orbital ressonance from the second moon may be a valid option to manipulate the rotation speed of the first moon too, i need to dig deeper on that for precise numbers.

so I guess it is an inbetween of a) and b).

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .