Could möbius ring shaped devices deflect or possibly redirect bullets?

Question

As a rule, my sci-fi has no force fields. This is interesting, because every type of shielding that would normally have no mass are restricted to be physical devices. The most notable I'm working on is a type of defensive device I call "guardian rings", although they are technically metallic bands rotating around the user. This is essentially further bulletproofing on top of kevlar vests and body armour.

Guardian rings are wearable metallic bands, that when active start rotating around the user at high speeds (500 RPM or higher). The bands are made with a ferrous or otherwise magnetic component in their alloy that allows them to be magnetized. By the flick of a switch a persons armour generates a magnetic field that accelerates the bands while keeping them at a safe distances from the wearers body. Otherwise the armour provides some protection from potential friction as well. While not in use the bands are pressed against the wearers body armour (flexible metal strips are in order).

Now, I wonder if such a device could offer proper protection from bullets.

The goal of the rings is to deflect (potentially reflect) incoming projectiles. Ideally the projectiles get caught in the bands and are redirected towards the attackers general direction, discouraging use of firearms. Although I doubt this would work on larger caliber projectiles it might at least deflect them away from the user. Worst case scenario the bands (being metal) offer mild protection from attacks. They can also decapitate a human in close combat. Pretty cool, right?

A normal ring won't do. Behold! Möbius strips (or rings):

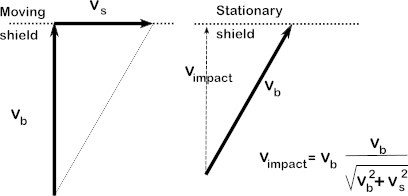
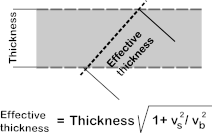
A shape with only one side reminiscent of the symbol for infinity. Its practical use is in conveyer belts, due to wearing all sides equally (makes sense as they only have one side). Guardian rings would thus rotate, deflect or catch a projectile which then revolves with the rings and gets shot back. Or something... Reality-check this please.

Answer 1

Now, I wonder if such a device could offer proper protection from bullets.

In reality, at 500rmp, it doesn't make too much sense, be them cylindrical (thin toroidal) or Moebius shaped.

I'll be modelling the impact speed that a bullet will exercise in a tangential moving shield as like the bullet hitting a stationary shield at an angle - on the grounds that the impact is not instantaneous and the moving shield will "drag" the bullet as it travels and that the bullet "sees" the shield with an "effective" larger "thickness". Something like in the figure below:

    

The "effective impact" speed of the bullet becomes

$$v_{impact} = v_b \frac{v_b}{\sqrt(v_b^2+v_s^2)} = v_b \frac{1}{\sqrt{1+v_s^2/v_b^2}}$$

Since "They [the shields] can also decapitate a human in close combat", it follows that they can also severe the limbs of the wearer, so they'll need to rotate at a safe distance from the body. Let's say a 1.2m (which is about double the average of an arm length) - a larger radius for the shield will have a larger tangential speed too, thus more able to present a larger "effective thickness".
At 500RPM, the angular velocity is $\omega = 52.36 rad/s$, with a radius of $R = 1.2m$, the tangential velocity becomes $v_s = \omega \cdot R \approx 63m/s$

To give the maximum chances for the shield to have a notable effect, I'll consider a low speed bullet. I don't know much about firearms, but I imagine that a large caliber weapon will have a lower muzzle velocity, so how about picking a shotgun - which is said to have a

relatively low muzzle velocity of slug ammunition, typically around 500 m/s

Plugging it into the formula above

$$v_{impact} = v_b \frac{1}{\sqrt{1 + {\frac{v_s}{v_b}}^2}} = v_b \frac{1}{\sqrt{1 + {\frac{63}{500}}^2}} = 0.9921\cdot 500 m/s$$

Which does get to show that your shield's rotation is, at best, very weakly effective (against an incoming bullet at regular speeds) in comparison with a stationary shield.

---

The fun fact: the shooter can even make the bullet (marginally) more effecting in piecing an armor with constant rotation. S/he just shoots at the angle incidence for which the addition of the two (vector) velocities gets again normal to the surface, so that the "effective width" becomes minimal (and equal with the actual width). In doing so, the relative speed on which the two object move will be greater than just the speed of the incoming bullet.

Granted, doing so, the bullet may not hit your heart, but the shooter will be shredding your (left or right, depending on the rotation of your armor) lung a bit more efficiently - if that's a thing you call an advantage.

---

The fact that your "rotating ribbon shield" is formed as a Moebius band will only introduce an extra weakness in the points where the ribbon $180^{o}$ turn happens and thus the apparent width of the ribbon (as seen by the incoming bullet) is smaller.