How can an Earth-like moon meet these angular diameter conditions?

Question

_{NOTE: If there is anything about this question that needs clarification or editing, please don't hesitate to let me know in the comments.}

---

Get ready, because you're in for quite a ride.

I have tried asking various questions based on different criteria, and I'm finally at my wits' end. In my (hopefully) last question about my solar system, I am providing the bare minimum constraints.

In my system, there is:

• A central star
• A gas giant orbiting the star
• An Earth-like moon orbiting the gas giant
• A sub-satellite orbiting the Earth-like moon

---

And here is what I am looking for:

• As viewed from the Earth-like moon; the star, the gas giant, and the sub-satellite have angular diameters of around 0.5 degrees. The gas giant may appear slightly larger. This means there are two types of solar eclipse: one where the gas giant moves in front of the sun, and one where the sub-satellite moves in front of the sun. There will also be an eclipse where the sub-satellite moves in front of the gas giant.
• The Earth-like moon is essentially an Earth clone; Earth-like habitability, surface gravity, seasons, atmospheric conditions, temperature, weather patterns, tidal forces, etc.
• The system must have been stable long enough for life to form on the Earth-like moon

---

Here are optional criteria that I would like to have, but I realize it might not be possible:

• The Earth-like moon has a cycle of seasons lasting 360 real-life days. This does not necessarily mean it orbits the gas giant in 360 days, it just means that winter, spring, summer, autumn last about 360 real-life days. (Earth-like seasons)
• The sub-satellite orbits the Earth-like moon in about 30 real-life days.

At this point, having asked so many questions trying to figure this all out, my only remaining question is this:

Are there any systems that will meet all of the above criteria?

Or should I just give up and cry?

---

What I am looking for in an answer:

• I'm looking for someone to actually help me find reasonable numbers for this system. I am looking for a hard-science answer.
• If there is a range of numbers that will work, please specify that and give me enough detail so that I may calculate or choose a suitable system for my world.
• Please do not say "it won't work" without explaining why it won't work. Also include the calculations you used that determine it is not possible.
• The more detail you provide in your answer, the better the answer is

---

Here's what can be changed, if necessary:

• If the tidal forces from a gas giant are too great, the mass and composition of the planet can be changed as long as it allows for the Earth-like moon to be Earth-like and have its own sub-satellite.
• If there is anything else that might need to be tweaked, please provide details in the answer. For example, if the angular diameter of the sun would need to be smaller (due to a greater distance from sun needed for orbital stability) in order to meet most of the criteria.

---

Some calculations I did to get you started

The smallest mass of a gas "giant" is around 6 Earth masses (a mini-Neptune), and the largest mass possible is 13 Jupiter masses (at which point it becomes a brown dwarf)

Looking at the planets in our solar system, the densities of the gas and ice giants are measured as being anywhere from 0.6 to 1.6 g/cm³.

With 6 Earth masses and a density of 1.6 g/cm³ (the smallest volume possible), the Earth-like moon would orbit at a distance of 4,025,050 km, having a period of 379 days.

With 13 Jupiter masses and a density of 0.6 g/cm³ (the largest volume possible), the Earth-like moon would orbit at a distance of 49,289,755 km, having a period of 620 days.

Increasing the angular diameter from 0.5 to 0.6 degrees, this changes the above two scenarios in the following way:

• Smallest Volume: Distance = 33,57,127 km, Period = 289 days
• Largest Volume: Distance = 41,110,541 km, Period = 472 days

Based on these calculations, it may be necessary to increase the angular diameter of the gas giant slightly.

NOTE: The mass and density allow me to calculate the volume, which allows me to calculate the actual diameter. From there, using the angular diameter I am able to calculate the distance, and then the orbital period.

---

Finally, here is a diagram I made in MS Paint:

---

If you would like to see my previous questions that are related, in order to gain more perspective on the matter (they also include the desired mass numbers, orbital configurations, and more) I have listed them here in chronological order:

1. Stabilizing Synchronized Orbit
2. Can planetary bodies have a second axis of rotation?
3. How can I have an Earth-like moon?
4. What kind of star will work for my system?

Answer 1

I'm pretty sure it can't be done with a gas giant. The problem lies in the stability of the habitable moon's orbit.

An object's orbit around its primary is stable as long as it is within the Hill sphere of the primary (the region dominated by the primary's gravity), while being outside the Roche limit (the distance at which tidal forces will break the object up). For long-term stability, the orbit should be no more than one-third to one-half the radius of the Hill sphere. The formula for the Hill sphere, assuming circular orbits, is:

$$ r_H \approx a\sqrt[3]{m \over 3M} $$

The first constraint is the requirement that the moon be habitable, while the sun has an angular diameter of 0.5 degrees. This pretty much requires putting the planet into an Earth-like orbit around a Sun-like star. Stellar luminosity increases far faster than stellar radius. As the habitable zone of a star moves out, the angular size of the star decreases; conversely, moving the habitable zone inwards increases the angular size of the star. Only stars of roughly one Solar mass will have an angular size of 0.5 degrees as seen from the habitable zone.

This sets the $M$ and $a$ terms of the Hill sphere equation. Only the $m$ term (the mass of the planet) is adjustable. Mass is proportional to the cube of planetary radius, the radius of the Hill sphere is proportional to the cube root of mass, making the Hill sphere roughly linearly proportional to the planetary radius.

For the given angular diameter, we can use the small-angle approximation to the tangent function. The angular diameter of the planet as seen from the moon is thus linearly proportional to radius, and inversely proportional to distance -- but the distance is constrained by the Hill sphere, leaving the planetary radius as the only adjustable parameter.

Put it all together, and to a first approximation, the angular diameter of the gas giant depends only on the density. In order to get an angular diameter of 0.5 degree, we need something a fair bit denser than Jupiter, or even Mercury.

I put together a spreadsheet to play around with numbers, and got the following result (all numbers are in meters/kilograms/seconds):

In the center is a very Sun-like star. Orbiting it, we've got a medium-large brown dwarf, 70 times the mass of Jupiter and 12% larger in diameter, taking about one Earth year to circle the star. Around that is a super-Earth, with nearly twice the mass and 25% larger giving an Earth-like surface gravity, in a 54.5-day orbit. And around that is an undersized Moon, one-third the diameter, zipping around in just under five days.

In terms of stability, Jove and Luna are both stable. Terra is borderline if there are other large bodies in the stellar system; if you need to increase stability, put it in a retrograde orbit.

In terms of habitability, Jove is squarely in the middle of the Goldilocks zone. Terra's orbit gives it about seven times as much variation in Solar distance as Earth's eccentricity currently does, but Earth has experienced more variation in the past (and will experience more in the future). This will give a distinct 54-day cycle to the weather, but a suitable orbital inclination (corresponding to Earth's axial tilt) can still give you distinct seasons.

Since the angular diameters of Luna and Jove are slightly larger than that of Sol, they are both capable of producing eclipses. The faster motion of Luna compared to that of the Moon means that eclipses will be much briefer, with totality never lasting more than a minute. Conversely, the slower orbital speed of Terra means that a Jovian eclipse of Sol can have a totality lasting up to 15 minutes.

Terra probably won't be tidally locked to Jove. Normally a moon in Terra's situation would be tidally locked, but the Terra-Luna system has far more rotational inertia than any moon.

Answer 2

TL;DR: maybe. Orbital stability is pretty borderline, and some fairly unlikely circumstances have to arise to produce something that looks like maybe it will fit your needs. Tidal effects and orbital resonances will mess with the figures below, so they're only approximate

---

Lets start with a star the size of the sun, putting the orbit of the planet at 1AU. We want a really big planet (because various orbital things tend to be more stable if the parent object is substantially more massive than its satellite), and for that we'll use a brown dwarf. A quick glance at wikipedia's list of brown dwarfs shows that there are many which are substantially more massive than Jupiter, but not actually much bigger. This is good, because it lets us maximise the size of the hill sphere around our planet, and minimise the orbital radius of the moon around it.

The Hill sphere of a body in the region in which its own gravitational attraction dominates that of its primary with regard to its own satellites, more or less. It is an approximation (because the three-body problem is Quite Hard to solve), and is defined for circular orbits as $$ r_H \approx r\sqrt[3]{m \over 3M} $$ where $r$ is the radius of the orbit, $m$ is the mass of the planet and $M$ is the mass of the primary.

If the brown dwarf is similar to COROT-15b, it'll be ~63.3 Jupiter masses, and 1.12 Jupiter radii. That means to satisfy the planet angular diameter constraint, your moon will orbit about 18.4 million kilometres from its parent. The brown dwarf has a Hill radius of 40.7 million kilometres, which is good... orbits more than about half the hill radius away from the parent tend to be unstable.

If your moon is the size of Earth, then given the mass and distance to the parent brown dwarf, it'll only have a Hill radius of 467828km. Taking half of this as the maximum safe orbital distance, the moonmoon would need to have a radius of ~1021km to satisfy the angular diameter constraint.

---

Your moon will have an orbital period of a little under 64 Earth-days. This might bir be quite right, because orbital resonances seem to be a thing and your moon gets quite close to the sun. I'm not going to rework this whole answer to provide a 6:1 resonance, but you might want to think about this. The moonmoon's orbital period would be a little over 13 days, and again: this doesn't fit into a neat orbital resonance, and so would probably be altered. Hopefully these peturbations won't completely destroy the system's hierarchy, but they'll probably ruin the nicely identical angular diameters.

The distance to the star will vary by nearly a quarter of an AU per cycle... that's quite a big change, and will have weird seasonal effects. It also means that the angular diameter of the sun will vary between 0.48 degrees and 0.61 degrees, which makes the nature of eclipses by the moonmoon quite different to the eclipses of the sun by our own moon.

Your moon will probably be tidally locked to its parent, which presents an issue in that this means that it will have a 32-day long period in which the star will not be visible from a whole hemisphere of the moon. I say probably because the presence of its own quite large, quite close moon is going to have odd effects in that regard. I'm not at all sure what will happen here, which probably means the situation is quite unstable.

The hemisphere facing its parent will always have a bright object in the sky which could be as big and as bright as our own moon (and maybe even significantly brighter, given the low albedo of the moon) and sometimes it will have its own moon in the sky too, so whilst the nights on the inner face will be long, they won't be dark.

The outer face (relative to the parent brown dwarf) will be alternately warmed by the sun and then cooled in the long dark night, and might not be very habitable except to quite specialised organism, but the inner face will be warmed by the heat of its parent which could be quite warm... perhaps as much as 1000K or more. I think you get to handwave its exact temperature, but the evolution of brown dwarfs might preclude this. I'm not going to work out the contribution of the brown dwarf's thermal radiation to the warmth of your moon, but you probably should (at least so you can get the dwarf's temperature right). There might be additional problems with the dwarf picking up a lot of heat from its parent star, given its size and proximity.

Really, you probably want the brown dwarf to be further from its parent to stay cool, but then the parent needs to be large to fulfill the angular diameter requirements, and so on and so forth. It is remotely possible that the working systems that satisfies all the angular diameter constraints and might have stables orbits and has a suitable habitable zone might actually have a red giant as a primary, but that whole thing just seems far too hard for me to even contemplate working out at this point.

---

NOTE: using a gas giant like Jupiter instead of a brown dwarf just won't work, because of the Hill sphere problem. I had a play with this, and the Hill radius is much too low for the sorts of moon orbital radii you need, and your moon will fly off into a solar orbit.

Answer 3

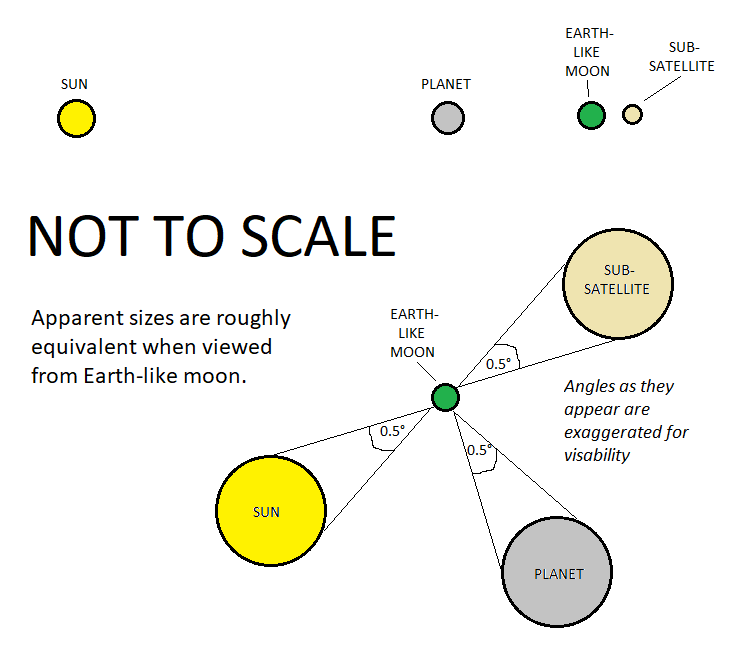
I note that astronomical bodies are requested with diameters and distances that give them angular diameters of about 0.5 degrees. An object will have an angular diameter of about 0.5 degrees when it is at distance of about 114.59165 times it's diameter.

The first thing that Overlord - Reinstate Monica should realize is:

The length of seasonal cycles on the Earth sized moon will depend on the orbital period of the planet around the star, not the orbital period of the Earth sized moon around the planet.

Overlord - Reinstate Monica wrote:

The Earth-like moon has a cycle of seasons lasting 360 real-life days. This does not necessarily mean it orbits the gas giant in 360 days, it just means that winter, spring, summer, autumn last about 360 real-life days. (Earth-like seasons)

The sub-satellite orbits the Earth-like moon in about 30 real-life days.

In real life a giant planet will exert tidal forces upon all its regular satellites - but not captured asteroids in distant orbits - that would adjust the satellite orbits so that the regular satellites, including hypothetical giant Earth like satellites, will have almost circular orbits in the equatorial plane of the giant planet. The tidal forces will also tidally lock the rotation of all the satellites so that their rotation periods or "days" will be the same length as their orbital periods around the giant planet or "months". Thus one half of each satellite will constantly face the giant planet and the other half of each satellite will constantly face away from the giant planet.

And the giant planet will do this very fast by astronomical standards, in a few tens of millions of Earth years. Since seasons are caused by the axial tilt of an astronomical body relative to its orbit around its star, and since the Earth like moon must have the same axial til as the giant planet, the cycle of seasons on the Earth like world will have the same length as the orbital period of the giant planet around its star, not the orbital period of the Earth like moon around the giant planet.

An orbital period of 360 "real days", presumably Earth days, of the giant planet around its star and within the habitable zone of that star is certainly possible.

The shortest known year of an exoplanet in the habitable zone of its star is 4.05 Earth days, for TRAPPIST-1d, while Earth is in the habitable zone of the Sun and has a year 365.25 Earth days long, and planets in the outer limits of the habitable zones of some stars should have years even longer than Earth does.

Our solar system has many examples of natural satellites of planets, dwarf planets, asteroids, etc. But there are no examples of any known natural sub satellites orbiting natural satellites in our solar system. There are no known natural satellites, or exomoons, in other solar systems, though there are a few candidates. If astronomers are not certain they have detected any exomoons yet, they certainly could not have detected any sub satellites in other solar systems.

And I believe that there are calculations of the orbital stability of sub satellites that indicate that natural sub satellites would be very rare and there would probably thus be a very restricted set of stable orbits that a sub satellite could have to orbit any particular specific satellite. Thus it would probably be very unlikely for a natural sub satellite to have a stable orbit in your fictional star system where you want it to be.

Assuming for the moment that there actually are a lot of sub satellites in other star systems and that our solar system is rare in not having any, we can make some assumptions about the orbits of your planet, your Earth like moon, and your sub satellite.

This article:

https://www.ncbi.nlm.nih.gov/pmc/articles/PMC3549631/ 1

Includes the statement:

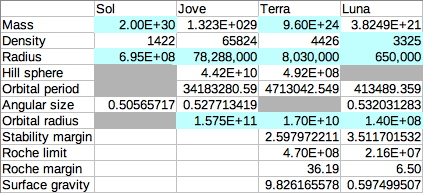
The longest possible length of a satellite's day compatible with Hill stability has been shown to be about Pp/9, Pp being the planet's orbital period about the star (Kipping, 2009a)

So if your planet orbits its star with a period of 360 days, your Earth like moon would have to orbit the planet with an orbital period equal to or less than one ninth or 0.11111 of the planet's year, or 40 days or less.

Assume for the moment that the orbital period of the sub satellite of the Earth like moon must have a similar relationship to that of the Earth like moon to that of the planet, in order to have long term stability. In that case the orbital period of the sub satellite round the Earth like moon would have to be one eighty oneth or less of the orbital period of the planet around the star, or 0.0123456 or less. With an orbital period of the planet around the star of 360 days, the orbital period of the Earth like moon around the planet would be 40 days or less and the orbital period of the sub satellite around the Earth like would be 4.444416 days or less.

Assume that the sub satellite has an orbital period of the desired 30 day length. Then the orbital period of the Earth like moon around the planet would have to be at least 270 days and the orbital period of the planet around the star would have to be at least 2,430 days.

Assuming that you meant that the Earth like moon would orbit the planet with a period of 360 days, and the sub satellite would orbit the Earth like moon with a period of 30 days. In that case the Earth like moon would have an orbital period twelve times as long as the orbital period of the sub satellite, and that seems sufficient to me. Then the planet would need to have an orbital period at least nine times as long as 360 days, or at least 3,240 days.

I suggest that you may need to give up the idea of having your Earth like world a moon of a giant planet and also have a sub satellite orbiting it. There would be no problems with making your Earth like world a satellite of a gas giant planet if your Earth like world didn't have a sub satellite orbiting it. And there would be no problems with making your Earth like world have a satellite orbiting it if the Earth like world was not itself a satellite of a giant planet.

But of course such a situation would result on only one astronomical body being able to eclipse the star of your Earth like world.

Or would it?

If your Earth like world is an Earth sized moon of a giant planet, maybe there are one or more other large moons orbiting that giant planet. And maybe one or more of the other large moons will sometimes be in position to eclipse the star of the system as seen from the Earth like moon.

Or possibly your Earth like world would be a planet orbiting its star. Such a planet could have no moon at all, like Venus, one moon, like Earth, two moons, like Mars, or possibly three or more moons. Of course the relative orbits of the moons would have to be such that they do not destabilize each other.

The Moon orbits the Earth at an average distance of about 384,399 kilometers. The Moon has an average radius of 1,737.4 kilometers & average diameter of 3,474.8 kilometers. At it's orbital distances, the Moon has an angular diameter of 29 minutes & 20 seconds to 34 minutes & 6 seconds, a range that includes the 0.5 degrees asked for.

If the moon was at a distance of about 192,200 kilometers, half of the distance in real life, it could have a diameter of about 1,737.4 kilometers and an angular diameter of about 0.5 degrees.

If the Moon was at a distance of about 96,100 kilometers, a quarter of the distance in real life, it could have a diameter of about 868.7 kilometers and an angular diameter of about 0.5 degrees.

If the Moon was at a distance of about 48,050 kilometers, an eighth of the distance in real life, it could have a diameter of about 434.35 kilometers and an angular diameter of about 0.5 degrees.

If the Moon was at a distance of about 768,798 kilometers, twice as far as in real life, it could have a diameter of about 6,949.6 kilometers and an angular diameter of about 0.5 degrees.

So it seems reasonable to suppose that the Earth could have two or more moons orbiting it in stable orbits at various distances that might all have angular diameters of about 0.5 degrees.

And there is this article https://planetplanet.net/2017/05/03/the-ultimate-engineered-solar-system/2 at the PlanetPlanet blog, that cites a scientific study showing that seven to forty two bodies with the same mass and equally spaced can share the same orbit with long tern stability.

So possibly a giant planet could have a ring of equally spaced Earth like moons around it, or an Earth sized planet could have a ring of moons around it.

Earth has a diameter of about 12,742 kilometers, so it should have an angular diameter of about 0.5 degrees at a distance of about 1,460,126.8 kilometers.

So if there could be seven to forty two equally space Earth sized moons orbiting a giant planet, the total circumference of their common orbit would be about 10,220,887 to 61,325,325 kilometers, thus giving the radius of their common orbit about the giant planet at about 1,626,706 to 9,760,236.6 kilometers.

And each of those seven to forty two Earth sized moons orbiting the giant planet might sometimes see the star eclipsed by one or another of the other Earth sized moons, and also sometimes see the star eclipsed by the giant planet.

The giant planets in our solar system have average diameters ranging from 49,532 kilometers (Neptune) to 142,984 kilometers (Jupiter). So they would have angular diameters of about 0.5 degrees at distances ranging from about 5,675,953.6 to 16,384,772 kilometers.

Combining the two calculations, a giant planet might have a ring of twenty five to forty two Earth like moons in a shared orbit with a radius of 5,809,664.8 to 9,760,236.6 kilometers. The two closest Earth sized moons to another would have an angular diameter of about 0.5 degrees, and if the planet had a diameter between about 50,698.8 kilometers and about 85,174.064 kilometers it would have an angular diameter of about 0.5 degrees in the sky of each of those Earth like moons.

I haven't calculated how long the orbital periods of the Earth like Moons at those distances would be.

And an Earth like planet could possibly have a ring of seven to forty two moons with distances about 114.59165 times their diameters, so that they appeared to have angular diameters of about 0.5 degrees.

Since such a ring of moons could be at most only a few times as far from the planet as the Moon is from Earth, the planet could have eclipses of its star by one or another moon several times as often as Earth does.

Note that the PlanetPlanet article more or less assumes that such a ring of equally spaced and equal mass objects orbiting a larger object would be extremely unlikely to occur naturally and would probably have been constructed by a highly advanced civilization.

Answer 4

This doesn't work around Sol, at the very least.

You calculated that a mega-Jupiter (13 Mj) would have the right angular diameter at ~49M km, which is right around 1/3rd AU. For it to eclipse the sun (which needs to be 1AU away to have the correct angular diameter) then the mega-Jupiter would need to be orbiting the sun with a semi-major axis of only 2/3rd AU, which puts it inside the orbit of Venus. This means that when the earth-like moon (ELM) is on its closest pass to the sun as it orbits the mega-Jupiter, it would be only 1/3AU from the sun, which is 1) inside the orbit of Mercury and 2) far too close to the sun for the ELM to stay in orbit around the planet (by comparison, the L1 point between the sun and the mega-Jupiter at 2/3AU would be about .1 AU from the planet)

The mini-Neptune (6 Me) at least does better in that respect -- Since the ELM is only orbiting it at ~0.027AU, it can orbit at ~1 AU without an issue. However, the problem then becomes that the mini-Neptune still can't hold on to the ELM gravitationally. The L1 point between the sun and a mini-Neptune at 1 AU is only 0.018 AU from the planet, which means it'd still lose the ELM to the sun's gravity.

I used this calculator to find the Lagrange-point distances.