There seems to be a lot of confusion and misunderstandings in the comments, so I thought a brief description of radioactive decay may be worthwhile. My goal is to explain how radioactive decay is tied to the fundamental laws of physics.
I'll start with a (very) brief description of the Standard Model of Particle Physics, which represents our best current understanding of matter and forces. In the Standard Model, there are 12 fundamental particles: six quarks and six leptons. The quarks are what make up nuclear matter; in particular, the two lowest-energy (and therefore stable) quarks are called up ($u$) and down ($d$). Similarly, the two stable leptons are electrons ($e$) and electron neutrinos ($\nu_e$). Each particle has an associated anti-particle which has opposite charge and opposite "lepton number" (for leptons) or "baryon number" (for quarks), but everything else is the same. The anti-particle of an electron is called a positron, and the anti-particle of a neutrino is imaginatively called an anti-neutrino. An interesting property of anti-particles is that, mathematically, they are equivalent to regular particles going backwards in time.
A proton is made up of $uud$ (each up quark has charge +2/3; each down quark has a charge -1/3). A neutron is made up of $udd$.
Of the four fundamental forces in the universe (electromagnetic, strong nuclear, weak nuclear, and gravity) the Standard Model talks about the first three and ignores gravity. Gravity, by the way, is described by General Relativity, which is a non-quantum theory that is fundamentally different from any quantum field theories such as those that form the basis of the Standard Model. In the Standard Model, the three forces are mediated (or, in a sense, caused or described by) by an exchange of a particle called a (vector) boson. Each of the forces has its own set of associated vector bosons:
- The electromagnetic force has one associated boson, called a photon. Photons only interact with electrically charged particles.
- The weak force has three bosons: $W^+$, $W^-$, and $Z$. These interact with all particles.
- The strong force has eight bosons, collectively called gluons. These only interact with quarks.
So, for example, an electromagnetic interaction happens when a photon is exchanged between two charged particles. Likewise, a weak interaction happens when a W or Z is exchanged between two particles. However, Weak interactions are a bit different in that, uniquely among all forces/interactions, they can change a particle from one type to another. So if a particle emits or absorbs a W boson, it will become a different type of particle.
For radioactivity, the most important example is a process called beta decay (https://en.wikipedia.org/wiki/Beta_decay), in which a down quark emits a $W^-$ boson and becomes an up quark; the $W^-$ then decays to an electron and an anti-neutrino. Equivalently, you can say the $W^-$ mediates a weak interaction between a $u$/$d$ and a $\nu_e$/$e$. There's also a variant in which a proton becomes a neutron via $u \to d \,\nu_e \, e^+$ mediated by a $W^+$ exchange. (The former is called $\beta^-$, and the latter $\beta^+$). The relevant Feynman diagram for $\beta^-$ decay is:
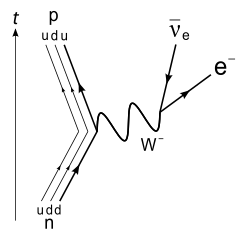
In the most general terms, radioactive decay is governed by all three forces in the Standard Model: strong nuclear, weak nuclear, and electromagnetic interactions. To quote the Wikipedia page (https://en.wikipedia.org/wiki/Radioactive_decay), "the combined effects of these forces produces a number of different phenomena in which energy may be released by rearrangement of particles in the nucleus, or else the change of one type of particle into others." However, for most unstable nuclei, beta decay is the most common cause of radioactivity.

This is what happens inside Carbon 14. Via beta decay, one of the neutrons in the nucleus becomes a proton (and therefore the carbon atom turns into a nitrogen atom), emitting an electron and anti-neutrino from the $W^-$ decay. The half-life is determined by strength of the weak force interaction, which is a function of the nucleons and their energy states. In principle, you could write down the Lagrangian and determine the half-life; in practice, this tends to be measured experimentally.
So if the half-life of carbon 14 were to change, it would mean some addition to the theory, i.e., you would have to add additional terms to the Lagrangian. This is not impossible -- there are many Beyond-the-Standard-Model theories that predict all sorts of changes, but the changes would have to be very subtle so as to have been missed all this time.
Edit
The process of actually calculating a half-life from first principles involves quantum field theory, which is too complex to fully explain here. However, I can explain some of the language that is used (with the perspective of someone with a particle physics background, however, rather than a nuclear physics background). Wikipedia links are included where appropriate.
In the language of QFT, radioactive beta ($\beta^-$) decay is a transition from an initial state ($udd$) to a final state ($uud + e^- + \bar{\nu_e}$). To calculate the transition rate (or half-life), we need to calculate the transition amplitude, which gives the probability of the transition between those two states. More specifically, we're interested in the S-matrix element, which is the transition amplitude from time $t=-\infty$ to $t=+\infty$. S-matrix elements are often expressed and visualized using Feynman diagrams. The transition rate can be calculated from the S-matrix element using a formula known as Fermi's Golden Rule.
Of course, for nuclear beta decay, the matrix element calculation isn't as simple as it is for free particles. There are complex nucleon-nucleon interactions that strongly affect the transition amplitude; these have to be modeled and accounted for in the QFT Lagrangian. Nuclear physicists have done this with good results, but it remains an area of active research (especially for isotopes far from stability). By the way, that same Lagrangian is where you would introduce any other interactions that might affect the half-life.



