Summary version:
So we're talking people living on top of 30 miles of topsoil, fake dino fossils and water on the outer surface on a hollow (but sturdy) unobtanium sphere with a radius about 1 AU. The sphere has an intermediate sized singularity at the core providing gravity of $1~g$ at sphere surface.
How can I provide sufficient lighting? I am not fussy about things like day length, I just want some light both equatorial and in my polar regions. How do I get it, without further liberal use of unobtanium?
Full Description
So I've been toying with the idea of a large Niven-like world. I don't like rings however. So instead, in an act of cosmic vandalism, I've handwaved in a hollow unobtanium sphere, using its frankly unbelievable compressive strength to effortlessly resist collapsing under $1~g$ of acceleration from a bikini-clad singularity at the center of the sphere, massing a moderate $1665~M_{☉}$ (solar masses). To make it more fun, my land, seas, tectonic simulators, dinosaur bone planters and other such-stuff is on the outside of the sphere.
This spares them from withering under the gaze of the bikini-singularity (for it is written that none shall see a near-naked singularity and live).
However, it does bring up the rather annoying question of providing adequate lighting for my inhabitants. First, however, a bit about the sizes we're talking about.
Let there be: $$g=G\frac{M}{r^{2}}$$
where
$g$ - local ("surface") gravitational field
$G$ - gravitational constant
$M$ - mass of another body
$r$ - distance from another body
$G=6.67428 \cdot 10^{-11}~\text{N}\left(\frac{\text{m}}{\text{kg}}\right)^{2}$
$M = 1,665~M_{☉}=3.33 \cdot 10^{33}~\text{kg}$ - our constructors like the number 3.
Solving for $g = 9.8~\frac{\text{m}}{\text{s}^{2}}$
We get $r=1.50 \cdot 10^{11}~\text{m}$, which suspiciously conveniently is right about 1 astronomical unit (i.e Sun-Earth distance).
So we're talking people living on top of 30 miles of topsoil, fake dino fossils and water on the outer surface on a hollow unobtanium sphere with a radius about 1 AU.
Now, I'm ok with the polar regions being a bit dark, but I don't want them in eternal darkness either, as it tends to do nasty things to my sphere's vapor circulation (think 60 mile glaciers). To provide lighting, I'll have a series of planets (I'm thinking somewhere in the range of Jupiter to Brown dwarf in size), which contain sufficient artificial fusion burners to provide day-like illumination.
I was thinking about having them in a nice Klemperer rosette (circling about sharing an orbit, with my burners causing occasional flaring to maintain orbits) like thus (not to scale, obviously):
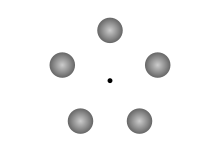
But assuming they're on the black-hole equatorial plane, this leaves my polar regions depressingly dark.
How can I provide sufficient lighting? I am not fussy about things like day length, I just want some light in my polar regions. How do I get it, without further liberal use of unobtanium?
