Let's say there's 2 planets orbit eachother as close as possible so they're oval shaped but not close enough to reach the roche's limit and be pulled apart. How would gravity be affected on the planets? Specifically, would one side of the planet have less gravity than on the other? And would you be pulled slightly sideways (towards the other planet) if you were standing on the poles of the planet?
Note: both planets share a similar mass and gravity.
Thanks in advance
Wikipedia's article on tidal forces has a nice diagram showing the orientation of the tidal force (= difference between compounded apparent gravity and the gravity due to the planet only) at different points. Long story short, the "inner" and "outer" extreme points have almost equal apparent gravity, which is less than the gravity due only to the planet; this tends to squeeze the planet.
Tidal force diagram. The body causing the tidal force is to the right, in the plane of the picture but outside the picture. The black arrows show the relative strength and orientation of the tidal force, that is, the difference between the apparent perceived gravitational force and the gravitational force due to the blue planet. Drawing by Wikimedia user Krishavedala, available on Wikimedia under the Creative Commons Attribution-Share Alike 3.0 Unported (CC BY-SA) license.
Intuitive explanation:
In a first order approximation, both the planet and a person or other object sitting on the surface of the planet are moving on an orbit around the barycenter of the system, so that the person or other object does not experience any force other than the gravity of the planet.
But in reality the planet is an object with non-zero size, so that most places on the surface of the planet are on the "wrong" orbit -- some move on an orbit with a smaller radius than what is right, others move on an orbit with a larger radius than what is right. Only the center of the planet moves exactly on the right orbit.
Objects placed at most points on the surface of the planet will therefore experience an apparent force tending to pull them away from the planet or to push them into the planet, because their movement on the orbit around the barycenter of the system does not balance perfectly the gravitational attraction of the other body.
This apparent force is called tidal force, and it is equal to the difference between the gravitationl force of the other body at that point and at the center of the planet. (Remember that the center of the planet is on the "right" orbit, so that the gravitational force of the other body is exactly cancelled by the orbital movement.)
The tidal force is maximum at the extreme points on the line between the centers of the two bodies, where it is directed opposite the gravitation of the planet; midway between the extreme points the tidal force is directed along the gravitation of the planet.
The tidal force decreases with the cube of the distance between the two bodies.
For example, the maximum tidal force due to the Moon experienced by an observer on the surface of Earth is at most about ten million times smaller than the gravitational force of Earth; that is, a 10 tonne elephant on the surface of the Earth will experience a tidal force due to the Moon of about 1 gram-force maximum, and a 75 kg human will experience a tidal force due to the Moon of about 8 milligrams-force maximum.
Bonus: The Explaining Science web site has a nice and gentle article explaining how to compute the tidal force.
Specifically, would one side of the planet have less gravity than on the other?
Trivially: yes. This is true on Earth... sensitive weighing scales can sense the moon, because as it passes overhead things get slightly lighter. On your binary world, you'll be lightest on the bits of a world's surface that have the other world directly above. Quite where you'd be heaviest I'm not quite sure... not quite on the point of a world furthest from the other (because the gravitational influence of the other world is at a minimum there), but I can't intuitively tell what the gravitational potential looks like as you travel around the world and I'm too lazy to simulate it.
You might lean a little sideways on the "poles"... but it isn't clear what would mark the poles anyway, as the bodies will be tidally locked (so no axis of rotation), and as they don't rotate nicely they might not have a geodynamo to provide magnetic poles, and they might not even have an axis of symmetry perpendicular to the line joining the two barycenters. The only real "poles" are the points on either world maximally distant from the other world.
Let's say there's 2 planets orbit eachother as close as possible so they're oval shaped
One key thing for two similarly massive bodies close together though is that you don't always get the two-tidal-bulge shape that AlexP included, but instead the bits of the two bodies that are closest together get drawn even closer together. This inside bulge is called a Roche lobe. The more fluid (or at least plastic) the body is, the more pronounced the effect will be. As you travel "up" the lobe towards the L1 Lagragian point between the two worlds apparent gravity will decrease until it becomes effectively zero.
I'm not sure how likely this is for a binary planetary system, but it isn't uncommon in binary stellar systems. Here's a diagram of the gravitational potential between two massive bodies... specifically stars in this case, but in a pinch it might do for planets, too.
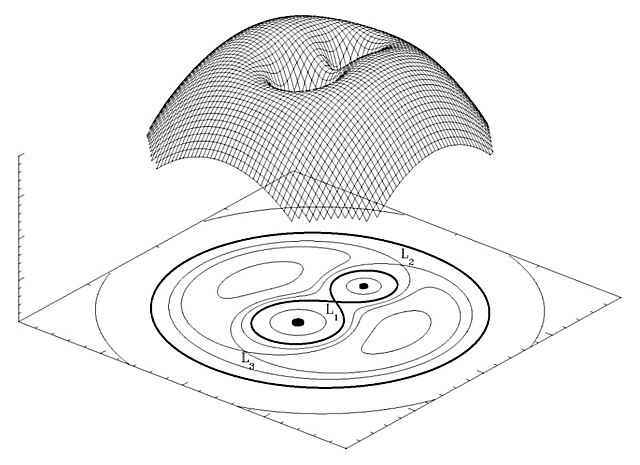
If you've not heard of Rocheworld, then that setting and discussion around it might be a good source of further information. There's plenty go on even on this site alone, eg. How would sunlight work in the area of shared atmosphere of a Rocheworld? or How Would It Feel To Walk On A Rocheworld?.
