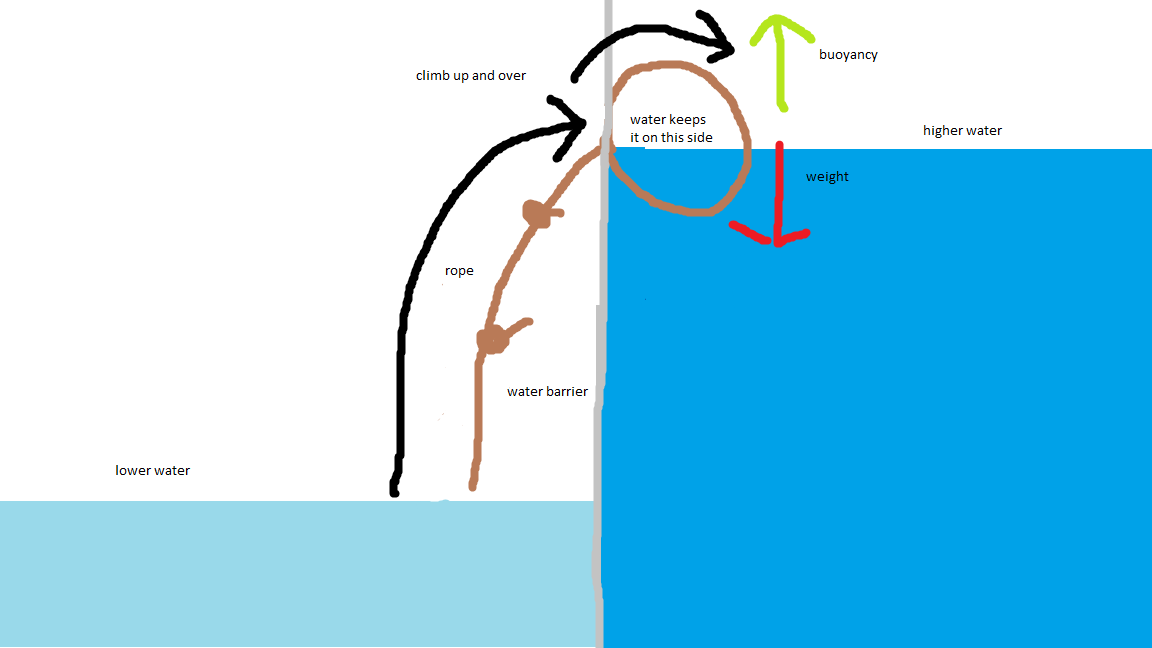
In my setting, there is an area that is usually filled with water at high tide and a land bridge at low tide. One day, a magical wall appeared that does not let water through, but does let through anything else. The shape of the wall is a vertical cylinder (with radius high enough that locally, it as well may be a vertical plane). The wall appeared during hight tide, so at low tide, there is now a vertical water surface.
- What is the safest way to cross this wall for a human during low tide in each direction? I can imagine that if a human is partially submerged in the water, then the water pressure would only create force on one of his sides and thus might try to eject him rather forcefully.
- How dangerous is it to cross in an "improper" way by a person who has no experience with this wall but is otherwise fit?
I figured when you would swim on the surface, you wouldn't be ejected sideways with a large force, but you will then fall form a really awkward position. On the other hand, trying to swim deep in the water will create a lot of sideways force but probably much more manageable fall. Still, you would be shot across the rough sea bed.
Things you can assume:
- The water at the border is about 10 feet deep.
- The floor is your average rocky beach, relatively lifeless, there aren't any large rocks but some of the smaller ones can be pointy.
- The people crossing it are a (relatively) weak high fantasy heroes in vaguely medieval setting (in fact, it's a low level DnD 5e party, but I am interested more in an real-world physics answer than a 5e mechanics answer, thats why I ask here. Assume Earth gravity, Earth-like salt water, regular physically fit human with no modern gear, also no other magic than whatever created the wall.)
- The wall behaves the way you'd expect it to without thinking too hard about it, eg. it lets through any liquid that is part of body of a living creature, and also the scenario where the hero gets hurt by a last drop of water that doesn't have anywhere to escape when it's pressed between the hero and the wall just doesn't happen. It also doesn't let through anything that is too small to be visible with a human eye (no pile of microbes/originally dissolved stuff right at the border) and the stuff that's dissolved in water doesn't sediment on it. At any time there aren't any major currents at either side of the wall, even if it would make sense for them to be present.