I want to have a realistic solar system as a home for my planet.
My planet must look like Earth, with the exception that each orbit around its sun takes 365 Earth years. Hence a season would last about 91 Earth years. However I want the planet to rotate on a normal cycle of 1 rotation per day and temperatures must resemble our planet's temperatures.
How big must the Sun be? How dense? Possibly another luminosity? And lastly is a moon (or multiple) still possible?
TL;DR: it would look much smaller than the Sun, and be bluer. It would also work like an apocalyptic germicidal lamp, and would blast away the atmosphere and sterilise the rocks underneath.
One problem here is that you have three unknowns, the mass of the parent star $M$, its luminosity $L$ and the orbital radius of the planet $a$. For the orbit itself,
$$T = 2\pi \sqrt{a^3 \over GM}$$
$G$ is the gravitational constant, and you'd like $T$ to be 365 years.
The amount of stellar irradiance your world receives is a factor of the luminosity $L$ of the star and the planet's distance from the star $a$. You want this to be more-or-less the same as it is on Earth.
$$I_s = \frac{L}{4\pi a^2}$$
Where $I_s$ is ~1361 W/m2 for Earth.
The mass-luminosity relation can help turn those three unknowns into two. On the assumption that your ideal star is between 2 and 55 solar masses,
$$L = 1.4L_\odot \left[\frac{M}{M_\odot}\right]^{3.5}$$
where $L_\odot$ and $M_\odot$ are the luminosity and mass respectively of our Sun.
With a bit of rejiggling, you can get a mass-orbital radius relationship for your star:
$$a = \sqrt \frac{1.4L_\odot \left[\frac{M}{M_\odot}\right]^{3.5}}{4\pi I_s}$$
And then you can throw that back into the first equation to give you a something that will let you compute the orbital period of a world with an Earthlike-irradiance around a star with a mass of between 2 and 55 Suns. (note Earthlike-irradiance, not merely Earthlike, because the spectrum of that radiation is likely to be quite different than that which falls on Earth)
Solving this equation analytically is much too hard, but it is easy to brute force solve numerically by entering values between 2 and 55 solar masses until the orbital period comes out about right. I got ~14.27 solar masses for your star. This would give you a B-type main sequence star that's over 15000 times more luminous than the Sun. Your planet would orbit it more than 123 times further away from it than the Earth is from the Sun.
Because the size of a main sequence star scales much more slowly than its brightness, the star in question might only be 6-7 times wider than the Sun... viewed from the distance of your planet, the star would have an apparent angular diameter about 1/17 that of the Sun. This tiny but intense lightsource would cast much more harsh and hard-edged shadows than those cast by our Sun.
There's nothing stopping you having moons.
Seasons are trickier though. That probably needs more careful thought about axial tilt and orbital eccentricity which I'm not going to do here.
There are also more serious issues with the formation of life-bearing worlds around stars which burn so hot and bright, because they have much shorter lifespans than cooler stars like our own. Your star might have only a thousandth of the Sun's lifetime, which doesn't bode well for nice planets.
edit: The UV Problem
Big stars aren't just brighter, they're hotter. As things get hotter, their black-body radiation spectrum gets bluer and starts having more and more short-wavelength radiation in the UV band. The surface temperature of the star I specced above is probably over 26000K, and using this handy calculator you can see that the peak of the emission spectrum is at ~100nm... extreme UV and perhaps more importantly vacuum UV that can't penetrate an atmosphere.
That means the sunlight hitting your world will rapidly heat and ionize its upper atmosphere, ultimately blasting it off into space leaving your world an airless sunbleached rock.
Sorry about that.
Based on your two constraints:
From the above you get $a = \sqrt{L\over L_{sun}}$ and $M=$$4\pi^2a^3\over GT^2$.
Substituting you get $M=$$4\pi^2(L/L_{sun})^{3/2} \over GT^2$
For $L/L_{sun}$ you can refer to the relationship listed here, with some iterations you should be able to figure out the values you are looking for: pick a relationship, calculate the mass and see if it falls in the domain where the chosen relationship is valid. If yes stop, if not move the next.
However this is a very simplified approximation: 90 years of insolation at summer regime will give a totally different outcome, climate-wise, than 90 days. And climate is chaotic system for which it's not easy to estimate what would happen.
Unless you really need to mingle in the nitty gritty details, just get an estimate of the distances and sizes involved and go on with those. There is no metal that can be melted only in a specific place on the planet, yet we enjoy the Lord of the Ring anyway.
I will preface this by noting my answers turned out very near what Starfish got. But my answers are without scary math things. I promise I did not cheat by stealing his deas. Except to think about the germicidal light piece he proposed and some sort of solution for that.
128 AU. And zodiacal light!
Mmm. Math. I struggle with it. I got out an online calculator. How far from our sun to have a 365 earth year orbiit?
https://www.calctool.org/astrophysics/orbital-period
51 AU.
Pluto is 39 AU away and a year of 248 earth years so that looks right. But Pluto is cold and dark. This sun needs to be brighter!
So backing into it - how luminous a star do we need to produce a habitable zone at ~50Au?
We need a star 1000 times the luminosity of the sun.
https://en.wikipedia.org/wiki/Stellar_classification
Looks like a class B star will do the trick. They get to 1000 the luminosity of our star and more.
But brighter probably means more massive. More massive means stronger gravity. If you are orbiting a more massive star you need to go faster to avoid falling in, and if you are going faster you need a bigger circle to do one circle in 365 years. Increasing sun mass means increasing distance from star. Those class B stars run between 2.4 and 16 solar masses. Let us assume 10 solar masses for Bright Blue. Let us see what 10 solar masses does to the orbital radius.
I think this does not match up with the habitable zone calculator because that calculator assumes masses comparable to our star. Let us max out the luminosity and mass of Bright Blue and see how far out the orbit is.
16 solar masses puts the orbit at 128 AU
Habitable zone for a maximally luminous B class is 164 to 290. Which means the 128 is too close and too hot.
We will keep 128 because we will use a shade to dim the star. I have in my mind @StarfishPrimes apocalpytic germicidal lamp. We do not want germicide. We want happy germs. We will use extinction. Not the apocalyptic kind. The astronomic kind.
https://en.wikipedia.org/wiki/Extinction_(astronomy)#Interstellar_reddening
Interstellar reddening In astronomy, interstellar reddening is a phenomenon associated with interstellar extinction where the spectrum of electromagnetic radiation from a radiation source changes characteristics from that which the object originally emitted. Reddening occurs due to the light scattering off dust and other matter in the interstellar medium... Reddening preferentially removes shorter wavelength photons from a radiated spectrum while leaving behind the longer wavelength photons (in the optical, light that is redder), leaving the spectroscopic lines unchanged.
Bright Blue kicks out a lot of short wavelength radiation: xrays, hard UV etc. That is what makes the germicide. But let us fiction up that between Bright Blue and your distant planet is a lot of dust. I envision a dust cloud. That cloud attenuates and scatters the hard radiation, leaving longer wavelengths to pass thru. Perhaps if the dust cloud is close to the star the cloud might itself be heated to shine in visible frequencies.
This is getting closer to @JohnWDailey ideas of an ultrabright object surrounded by an accretion disc, with a distant planet warmed by the radiation diffused (extincted?) by the disc. Example:
What would the climate of this habitable world be like?
The appearance of Bright Blue would not be a superbright tiny point (with sharp shadows; I liked that piece!) but a bright spot surrounded by a comparably bright ring of haze. Possibly with rainbow colors? Probably it would be much like the zodiacal light!
https://en.wikipedia.org/wiki/Zodiacal_light
The zodiacal light... is a faint glow of diffuse sunlight scattered by interplanetary dust. Brighter around the Sun, it appears in a particularly dark night sky to extend from the Sun's direction in a roughly triangular shape along the zodiac, and appears with less intensity and visibility along the whole ecliptic as the zodiacal band.6 Zodiacal light spans the entire sky and contributes7 to the natural light of a clear and moonless night sky.
One possibility of having a planet with with an orbital period of 365 Earth years, would be to have the planet orbit around a group of stars instead of a single star.
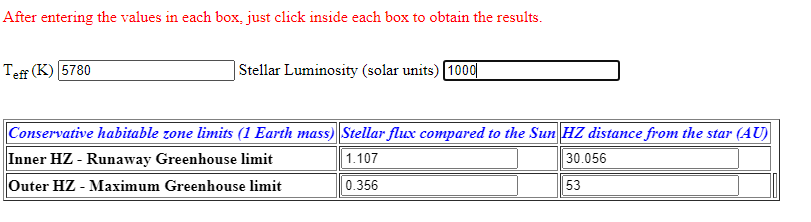
Assume that two stars orbit each other in an almost circular orbit with a semi-major axis of five million killometers.
Suppose the system has another very similar pair of stars with a similar orbit around each other. The two pairs might orbit each other with a semi major axis of twenty five million kilometers.
And then suppose that there are two such systems of four stars orbiting each other with a spearation of about one hundred twenty five million kilometers.
If there was a system with eight stars orbiting their common centers of gravity within about one hundred twenty five million kilometers of the common center center of gravity, a planet could orbit around the common center of gravity of the entire system with a stable orbit if it was far enough beyond the orbits of the stars.
It could probably have a stable orbit with a semi-major axis of six hundred and twenty five million kilometers five times wider than the separation between sets of stars.
It might be able to orbit with a stable orbit even closer to the stars.
For a circumbinary planet, orbital stability is guaranteed only if the planet's distance from the stars is significantly greater than star-to-star distance.
The minimum stable star-to-circumbinary-planet separation is about 2–4 times the binary star separation, or orbital period about 3–8 times the binary period. The innermost planets in all the Kepler circumbinary systems have been found orbiting close to this radius. The planets have semi-major axes that lie between 1.09 and 1.46 times this critical radius. The reason could be that migration might become inefficient near the critical radius, leaving planets just outside this radius.
https://en.wikipedia.org/wiki/Habitability_of_binary_star_systems
Two to four times one hundred twenty five million kilometers would be two hundred fifty million to five hundred million kilometers.
And of course stable orbits would be possible out to distances of tens or hundreds of billions of kilometers, so there would be a wide range of orital distances possible.
So if you assume that all the eight stars have very similar masses and luminosities, you can choose a mass and calculate an orbital distance where the planet has an orbital period of 365 Earth years with that total mass of the eight stars.
And you can choose a mass and thus luminosity for the stars and then choose an orbital distance where the star receives enough radiation from the combined eight stars to have the proper surface tempertures.
But the problem would be combining the right orbital period and the right amount of radiation. Choosing a combined mass and luminosity of the eight stars so that the planet has an orbital period 365 Earth years long and also receives the right amount of radiation for the right temperurature might be impossible.
But replacing a central star with a group of two, three, four... and maybe up to eight central stars, may make the problem more flexible than using only one star.
Fortunately, I have thought of a diferent type of year a planet could have. I will add that to my answer later.
This part was added the 11-19-2022.
Suppose that the world in the story is a giant moon, large enough to be habitable, orbiting a giant planet, or maybe a brown dwarf, which in turn orbits around the star (or stars) in the system.
So the giant planet with the habitable moon could orbit the star at the corret distance to give it an orbital period of 365 Earth years based on the mass of the star (or stars).
But if the central star has a low enough mass to shine fairly steadily for billions of years in order for the planet to become habitable for oxygen breathers, the planet and the moon would have orbit out where the radiation from the star would be very faint and insufficient to make the planet warm enough for liquid water using life.
If only there was some other potential sources of heat for a giant moon orbiting a giant planet.
Tidal interactions between the moon and its planet can cause sufficient tidal heating for liquid water oceans on the surface.
Theoretical studies of the possibiity of habitable exomoons of giant exoplanets show that the extent of tidal heating on those exomoons depends on their distance from their planets, among other factors. As a rule the closer to the planet, the greater the tidal heating on the moon.
If the moon orbits too close to the planet, the tidal heating will be enough to initate a runaway greenhouse effect, turning all the surface water into atmospheric water vapor. If the moon orbits even closer, the tidal heating will cause excesse vulcanism on the moon, making it a volcanic hell like Io.
Thus the phrase "habitable edge" for the inner limit of how close a moon can orbit to its planet while avoiding excessive tidal heating.
And it should be obvious that if the planet and moon orbit too far from their star for stellar radiation alone to warm the moon enough for liquid water, the moon could still be warm enough if it orbits outside the tidal edge but close enough for enough tidal heating for liquid water oceans.
There would still be a problem with the moon getting enough light from the star for photosynthesis on the surface of the moon for plants to produce an oxygen atmosphere.
Fortunately, a small increase in the mass of a star will cause a greater increase in the luminosity of the star. Thus the correct distance to receive a specific amount of radiation from the star will increase more than the star's mass and graitational pull on a planet. So the more massive and luminous the star is, the longer the orbital period of a planet receiving exactly as much radiation from the star as the Earth will be.
Using the most massive and short lived star that I dare to, the more massive end of spectral class F stars, I found that a planet at the Earth Equivalent Distance from such a star would have an orbital period several Earth years long. I also found that putting two stars at the center of the star system would inrease Earth Equivalent distance, but it would increase the combined stellar gravity more and would make the orbital period at the Equivalent Distance shorter than for a single star of that mass.
And of course you would want a planet in an orbit so far that it would have a year 365 Earth years long, so it's orbit would be several times as far from the star as an Earth Equivalent Distance would be anyway. But that might possibly help with giveing the habitable moon enough light for photosynthesis.
With years 365 Earth years long, the astronomical seasons would each be 91.25 Earth years long. And some of the comments express fear that such long hot summers and long cold winters would be deadly for life on the planet, or in this case, exomoon.
The way to handle this is to give the planet a vert low axial tilt, so that the inclination of incoming solar rays is almost exactly the same in every season of the year.
And in the case of a giant habitable exomoon orbiting around a giant planet and getting most of its heat from tidal heating, the astronomical seasons wuld not cause climatological seasons on the Moon. The temperatures would be quite constant all year.
So why would the people on a planet or moon with such even and unnoticeable seasons bother with counting orbital periods around the star and making them the years that their calendars are based on?
Because of the changing night time stars. At any given moment one side of a planet or a planet's moon will be facing toward the star of the system, and the atmosphere on that will scatter the bright star light and making the sky appear opaque and hide the stars. And on the opposite side of the planet from the star, the atmosphere will have light from it to scatter, and the sky will be transparent, and the stars will be seen. The stars which are in the opposite direction to the star in that system.
On Earth, as the Earth slowly orbits the Sun, the direction opposite to the slowly changes, and so the stars which are visible at night slowly change over the year.
And so the stars visible at night on that planet will slowly change over the course of a 365 Earth year orbit. So possibly the stargazers on that planet treasure star charts handed down from earthly decades and centuries earlier during the 365 year long orbit and calculate how long wit will be until eachs uch set of stars is visible again.
So that might give the natives of the planet a reason to have a calendar period equal to 365 Earth years, or one orbital period of the planet, even without dramatic seasons.
