26
$\begingroup$

I have built a Tchou-Tchou 'hyperloop' wagon that reaches 310 km/h on a 500 meter test rail. One of my investor customers wants to try the Tchou-Tchou, but I am a bit concerned about his safety. To impress him, the train is configured to reach 310 km/h with constant acceleration after 250 meters, then slow back down to 0 km/h with constant acceleration at 500 m.

Will he survive the best case scenario? And what is the best case scenario?

Improve this question
$\endgroup$
17
  • 7
    $\begingroup$ If I'm not completely mistaken, that should somewhat over 2G, nothing a human body cannot handle, especially since it's only a few seconds. $\endgroup$
    – Florian Schaetz
    Commented Aug 4, 2017 at 12:07
  • 2
    $\begingroup$ I've been wondering the same while watching The Flash. That guy's body has been altered to handle the high speeds. He even has a special suit to help with it. Sometimes his shoes catch fire if he runs with his regular shoes. He picks up people and runs around with them. Those people's bodies aren't altered the way the Flash's body is, nor are they wearing special clothes. I've been wondering why they don't suffer damage, and why their clothes don't catch fire the same way the Flash's shoes do. $\endgroup$
    – Raf
    Commented Aug 4, 2017 at 13:08
  • 2
    $\begingroup$ @Raf the Flash uses the speedforce to shield them frlm the effects I guess? (But then, why can't he shield his own shoes,I know.....) $\endgroup$
    – Patrice
    Commented Aug 4, 2017 at 13:16
  • 7
    $\begingroup$ @Raf In a lot of the scenes you see in movies where people are snatched up at hyper-speed before a vehicle hits them, or while falling just before hitting the ground, they actually would die from the sudden acceleration of it, or in the ones that aren't quite as severe they would still be injured even if they did not die. Falling from 100 stories, or from a plane, there is no way to rescue someone once they are only a few feet from the dirt or road, no matter how fast you can move; they are seriously injured or dead even if you are superman or flash. Movies require suspension of disbelief. $\endgroup$
    – Loduwijk
    Commented Aug 4, 2017 at 16:43
  • 2
    $\begingroup$ @Aaron - There is a way, but I never seem to see it. If someone can excavate fast enough, they can create enough space to decelerate a falling person more gradually. Some versions of Superman certainly would have been capable of this (but I don’t think they ever used it). $\endgroup$
    – Obie 2.0
    Commented Aug 6, 2017 at 23:51

8 Answers 8

Reset to default
13
$\begingroup$

To expand on @a4android s Comment

For simpler numbers the following is calculated for a top speed of 100m/s

You can travel 500m in 10s with all of the following regimes:

  1. constant acceleration of 20m/s2 for 5s then -20m/s for 5s. This solution has the lowest maximum acceleration/deceleration, but it has aprupt changes of the acceleration which are dangerous to the passenger.

  2. Linearly increase the acceleration to 40m/s2 for 2.5s then linearly decrease to -40m/s2 for 5s then linearly increase to 0 for 2.5s. Here the acceleration is a continuous function without aprupt changes, but you need double the maximum acceleration. At around 4g this is still in the roller-coaster range.

The best is probably an intermediate solution, for example:

  1. Linearly increase the acceleration to 25m/s2 in 1s then keep it constant for 3s then linearly decrease acceleration to -25 for 2s, keep it constant for 3s and go back to 0 in 1s. This has a much more moderate top acceleration of around 2.5g and also has no aprupt changes in acceleration.

enter image description here

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Excellent! Glad to see my comment expanded and built upon into a good answer. Plus one for being well done. $\endgroup$
    – a4android
    Commented Aug 6, 2017 at 4:52
  • $\begingroup$ "aprupt changes of the acceleration which are dangerous to the passenger" mmm, no, it's the abrupt changes in velocity, which are acceleration peaks, that are dangerous to the passengers. $\endgroup$
    – theGarz
    Commented Jul 20, 2018 at 8:54
  • 1
    $\begingroup$ @theGarz both are (Jerk) $\endgroup$
    – user19058
    Commented Jul 24, 2018 at 5:49
77
$\begingroup$

310 Km/h is 86 m/s. This means that on your 250 meters track (for acceleration), you'll have a mean speed of 43 m/s, meaning that you'll reach your 250m in 5.81 seconds. Now, 86 m/s reached in 5.81 s is 14.8 m/s², or about 1.5 g (same for deceleration). Maybe not really comfortable, especially for "regular" people not used to this kind of acceleration during transportation (except for rollercoasters), but undoubtely survivable.

Improve this answer
$\endgroup$
16
  • 19
    $\begingroup$ Thanks for your great math. We survive! You will be invited next test! $\endgroup$
    – Drag and Drop
    Commented Aug 4, 2017 at 12:28
  • 25
    $\begingroup$ Be aware that the start and end of the track, and especially the switch from acceleration to braking in the middle, are prime opportunities for your investors to sue you for causing whiplash if you haven't strapped them in properly. $\endgroup$
    – Steve Jessop
    Commented Aug 4, 2017 at 12:52
  • 18
    $\begingroup$ The switch from accelerating to decelerating will not by very comfortable. That is an sudden 3g changes from laying on your back to hanging upside down. I hope the investor is strapped down well and doesn't get launched away :) $\endgroup$
    – Dorus
    Commented Aug 4, 2017 at 12:52
  • 19
    $\begingroup$ I suggest a higher constant acceleration & deceleration and allow more time for the change from acceleration to deceleration. Say, three seconds acceleration, change from acceleration to deceleration with three seconds braking. Undoubtedly uncomfortable, but less chance of whiplash. $\endgroup$
    – a4android
    Commented Aug 4, 2017 at 13:05
  • 5
    $\begingroup$ To expand on @a4android s Comment: With a top speed of 100 m/s = 360 km/h (simpler numbers): you can travel 500m in 10s with all of the following regimes: constant acceleration of 20m/s2 for 5s then -20m/s for 5s. Linearly increase the acceleration to 40m/s2 for 2.5s then linearly decrease to -40m/s2 for 5s then linearly increase to 0 for 2.5s. The best is probably an intermediate: linearly increase the acceleration to 25m/s2 in 1s then keep it constant for 3s then linearly decrease acceleration to -25 for 2s, keep it constant for 3s and go back to 0 in 1s. i.sstatic.net/zZbGk.jpg $\endgroup$
    – user19058
    Commented Aug 4, 2017 at 14:24
34
$\begingroup$

Tsts, it's easy peasy.

Colonel John Stapp made progressively harder and harder experiments with deceleration himself to find out what the human limits are (He advocated the safety belt, by the way).

enter image description here Wikimedia, Public Domain

He stopped at December 10, 1954 with the rocket sled Sonic Wind from 1,017 km/h (632 mph) to zero in less than 1.4 seconds, experiencing a deceleration of nearly 46 g, meaning that the straps fixing him needed to hold the weight of an Indian elephant (Stapps weight was 77 kg (170 pounds), so the equivalent force was 3,5 t!).

For the more pragmatic people: The shorter the timeframe, the more g the human body can tolerate.

  • 3 g = -30 m/s^2 is something even old people can manage.
  • 5-7g = -50/70 m/s^2 will pass out most people if prolonged depending on fitness, roller coasters are in the vicinity of 5g.
  • 7-9g = This is really uncomfortable now; untrained people will stay conscious only for a few seconds and prolonged exposure will cause death.
  • 9-12g = Only extremely fit and trained people are able to handle this for a longer time (minute range): astronauts, fighter & aerobatics pilots.
Improve this answer
$\endgroup$
21
  • 16
    $\begingroup$ literally an elephant was not on his back... literally he experienced a force equivalent to an elephant being distributed through his body $\endgroup$
    – matt
    Commented Aug 4, 2017 at 14:47
  • 17
    $\begingroup$ @matt - he experienced a force equivalent to an elephant's weight being distributed throughout the area, on his front, where the safety belt was. $\endgroup$
    – user11864
    Commented Aug 4, 2017 at 14:50
  • 4
    $\begingroup$ If you don't mind having your eyeballs filled with blood, 50 g is just fine. Also, further complications including death could arise (do NOT try this at home) $\endgroup$
    – Keelhaul
    Commented Aug 4, 2017 at 14:54
  • 27
    $\begingroup$ I say I want to "impress" my investor, not "In a press". I a common mistake. $\endgroup$
    – Drag and Drop
    Commented Aug 4, 2017 at 15:00
  • 4
    $\begingroup$ @AgapwIesu 60kg?! Are you a pygmy? German men have an average weight of 84 kg, German women a weight of 68 kg, average is 76 kg.And if you start nitpicking, for an Indian elephant the mass is 4t/2.7t. So the ratio of 1:50 is a good fit. $\endgroup$
    – Thorsten S.
    Commented Aug 4, 2017 at 22:13
7
$\begingroup$

The correct formula to use here, given constant acceleration, is $v_f^2 - v_i^2 = 2ad$.

So $$a = \frac{v_f^2 - v_i^2}{2d}$$ with $$\begin{align} v_f &= 310\ \mathrm{km/h} = 86.11\ \mathrm{m/s} \\ v_i &= 0\ \mathrm{m/s} \\ d &= 250\ \mathrm{m} \end{align}$$ you get an acceleration of $14.83\ \mathrm{m/s^2}$ or about $1.50g$. This is well within human limits.

Improve this answer
$\endgroup$
1
  • $\begingroup$ PS: there are roller-coasters that pull over 6 g. And I imagine most roller-coasters for adults hit more than 1.50 g, although they probably do it more by changing the direction rather than the magnitude of the vehicle's velocity. And they probably hit the higher g's in smaller, even tiny bursts. $\endgroup$
    – user11864
    Commented Aug 11, 2017 at 15:31
6
$\begingroup$

I have done some math...

The distance you travel while accelerating with constant acceleration is

$d= 1/2 a t^2$

while the velocity you reach in the same time is

$v = at$

since you state the distance and the velocity, we can solve it in acceleration and time.

$1/2 at^2 = 250$

$at = 86$

Which gives $a = 86^2/500 = 14.792 m/s^2$, almost exactly 1.5 g for a total of 12 seconds.

Improve this answer
$\endgroup$
4
  • $\begingroup$ if 1/2at<sup>2</sup> = 250, how do you get at=86? $\endgroup$
    – user11864
    Commented Aug 4, 2017 at 14:39
  • $\begingroup$ @AgapwIesu Because 250 / 2 = 86, obviously. $\endgroup$
    – corsiKa
    Commented Aug 4, 2017 at 15:45
  • 1
    $\begingroup$ @Agapwlesu It isn't one to the next, they're separate equations (which lets us solve them later). One is distance traversed, the other is velocity achieved. We know the distance and the velocity, so we can plug those in for d and v. $\endgroup$
    – Delioth
    Commented Aug 4, 2017 at 18:09
  • 1
    $\begingroup$ > how do you get at=86? — by using a fact "train is configured to reach 310 km/h". 310 km / h = 310'000 m / 3600 s → 86,1(1) m/s $\endgroup$
    – poige
    Commented Aug 5, 2017 at 8:39
3
$\begingroup$

Top fuel dragsters currently accelerate from 0 to about 335 MPH (~540 KPH) in 1000 feet (~305 meters), taking about 3.5-4 seconds to do so (giving a little over 5 G's of acceleration). They then decelerate back to 0 in about another 5 seconds or so (around 3 G's of deceleration).

This is fairly impractical though. To do it, the cars use engines that produce around 10,000 horsepower. That puts enough wear and tear on the engine that it's standard practice to completely rebuild the engine every run.

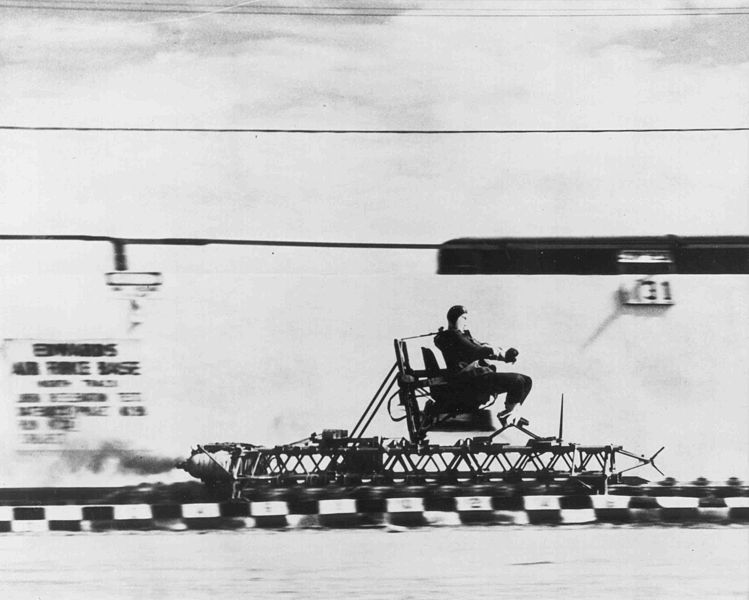
Fighter jets can generate quite a bit more acceleration than that in a tight turn. Most have acceleration limiters, so they won't exceed about 8 G's, and will only maintain that for a very short time, then the jet will automatically "loosen" the turn to keep the pilot from passing out.

In this case, the acceleration as felt by the pilot is normally "downward"--i.e., pushing him/her down in the seat, rather that backward like the acceleration in a dragster. This has a significant effect--since it's pulling "downward", it's more difficult for the heart to pump blood to the brain. This leads to a "grey out" effect, where the brain (and eyes) are receiving little enough blood that vision becomes somewhat impaired.

Even achieving that takes fairly drastic measures--pilots wear "speed pants" to "squeeze" their legs, helping force blood upward instead of pooling in their legs. The "seat" in a modern fighter is also fairly reclined (e.g., around 30 degrees) to make it somewhat easier for the heart to pump blood to the pilot's head.

Getting to your actual question: these are probably close to the limit of what you can expect people to endure on a semi-regular basis. Accidents are often catastrophic, and even in the absence of catastrophic accidents the acceleration and deceleration take a substantial toll on drivers/pilots. A common injury among top fuel drivers is detached retinas. Don Garlits (top fuel driver, now retired) had surgery to fix a detached retina, and has admitted that it was fairly routine that the initial launch left him feeling "woozy" until he reached around the 300 foot mark.

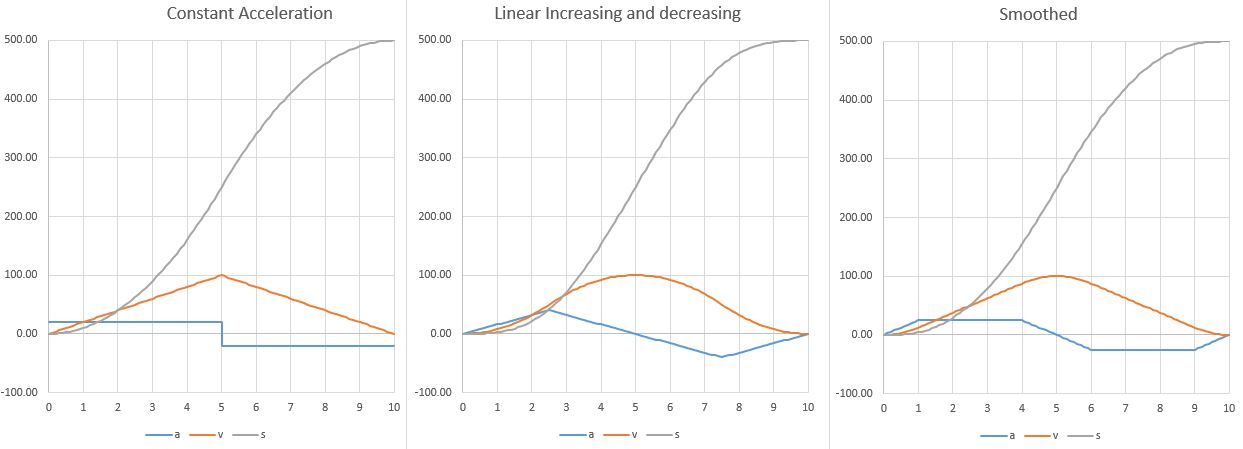
So, getting to your specifications: accelerating at 1.5 G's should be no problem for any reasonably healthy adult. If you double that to 3 G's, there's still little likelihood of its being life threatening (especially given the relatively short track your postulating).

Tripling the acceleration to 4.5 G's gets you into the range where it's still entirely survivable, but you'd want to ensure the investor had a physical quite recently--it's getting to the point that you'd want to ensure that s/he was healthy enough rather than being able to take it for granted just because you didn't know of his/her being particularly unhealthy.

Improve this answer
$\endgroup$
1
$\begingroup$

Top fuel dragsters can reach speeds of upwards of 400 km/h in under 3.2 seconds while traveling a distance of just 201 meters. They then decelerate quite rapidly using a combination of drag chutes and then wheel braking systems. The experience is no doubt extremely violent and uncomfortable, but drivers generally emerge from their cars unscathed.

Improve this answer
$\endgroup$
-4
$\begingroup$

Bugatti Veyron

This is a real world example using the fastest production automobile. It's not as fast as your requirements, but I think it gives some great information on the physics and power requirements to do what you want and the video link is entertaining.

The Bugatti Veyron has a top speed of 408.47 km/h (253.81 mph) and can go from 0 to 408 km/h to 0 in 90 seconds. Yes, it's going to require slightly more track than your Tchou-Tchou, but if you can do this in a production car, you really could do this in your hyperloop.

There are engineering challenges to consider.

You don't describe your top speed of your Tchou-Tchou. I assume it will do more than 100 mph. There are some parallels you should keep in mind to improve the story.

In the case of the Bugatti, it requires 250hp to achieve 100 mph. To achieve 253 mph, it needs another 750hp for a grand total of 1000hp. This means the engine powering the Tchou-Tchou needs to quadruple it's output just to make your challenge work.

Much of that is the resistance caused by the air in front of the car which creates friction and slows it down. You will have the same issues in a hyperloop tube because even if it's a vacuum and lower air pressure, it's going to be really hard to make complete vacuum. The biggest challenge with pushing what becomes in essence a ram down a tube is how to displace the air in front of the vehicle. In subways or rail tunnels, they build ventilation shafts to give the air somewhere to go besides forward. If you ride a subway regularly in an underground station, you already experience this to a degree when the air in the tube blows by you when a train is coming into the station.

Others covered stopping already, which is possible, tolerable, but maybe not so much fun.

Video of speed test

I really recommend the video. It's an engineer and television host explaining the engineering challenges of developing a car which is able to achieve significantly higher speeds than most vehicles.

Good luck.

Improve this answer
$\endgroup$
5
  • 6
    $\begingroup$ This device is on the order of seconds, the Veyron takes much more time, and thence much more track. If much more track is needed, the acceleration is obviously much lower, making this response unhelpful at best, and largely gratuitous. $\endgroup$
    – Nij
    Commented Aug 4, 2017 at 21:14
  • $\begingroup$ I just outlined the physics problem of going from zero to 400 to zero, defined the power requirements, the reasons why so much power is needed, issues with accelerating in a tube and wrapped it up in a pretty package and you criticize the distance? VW spent $1 billion developing this car and learned a lot about the physics challenge. Stop looking at the pretty name badge and apply the lessons from the building the car to strengthen the story. $\endgroup$
    – gwally
    Commented Aug 5, 2017 at 0:04
  • 8
    $\begingroup$ The point remains that a car taking minutes to achieve a worse result is clearly not operating at the same level as a device that takes mere seconds to achieve greater accelerations. You may as well talk about bicycles for all that it's the same "physics challenge". Finally, the question is about whether a human being can survive the acceleration: technology for doing so is irrelevant. $\endgroup$
    – Nij
    Commented Aug 5, 2017 at 1:00
  • $\begingroup$ I just fail to see the point of the answer. But it could be because of my English skill. 1/. " require slightly more track " five mile track vs 500 meter. 2/. " you can do this in your hyperloop" It's already happening, Xp-1 Hyperloop One have made it. It's not Production but they are already trying to beat that speed on the same track. 3/. "engineering challenge " I get this one! But still not really revelant to the question but you re right, But the issue is no the one you are thinking. When at 0.01 Atm if the tube break the amount of energy that will be generated is Big. $\endgroup$
    – Drag and Drop
    Commented Aug 7, 2017 at 14:38
  • $\begingroup$ The hardest part is to make a viable tube for long distance. 4/. "250 hp " well Horse power is quite interesting but still not related to the question. It's like talking about the price So 5/. "Good Luck Buying One" How? Why? I m mean try to answer mentaly to the newt question with "Good Luck Buying One" .. 1 ..2 ..3 Can I die falling from my chair? .. There is no way this could be an answer to the question. I appreciate that you bring this beauty(Chiron). But still . I don't see any way to edit your question to make it fit. $\endgroup$
    – Drag and Drop
    Commented Aug 7, 2017 at 14:44

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .