45
$\begingroup$

For example, a street this wide to have enough blood run down the length shown in this photo? Assume that the blood isn't going down storm-drains for whatever reason and assume the stretch of road is 100 feet long. There need not be literal 'rivers' of blood, just enough that the entire street is coated enough to obscure the road beneath. The bodies the blood comes from can be included in the answer.

enter image description here

Yes, this is a morbid question but to be fair, it's for a very dark part of a story. If it breaks some rule I would be fine with it being removed.

Improve this question
$\endgroup$
12
  • 28
    $\begingroup$ I'm noticing a pattern with your questions... $\endgroup$
    – Joe Kissling
    Commented Apr 19, 2017 at 20:19
  • 21
    $\begingroup$ Why are you stopping to count? $\endgroup$
    – SRM
    Commented Apr 19, 2017 at 20:24
  • 14
    $\begingroup$ depends on the weather, 2/3 rain 1/3 blood is still pretty red. How smooth the road is is also a factor. $\endgroup$
    – John
    Commented Apr 19, 2017 at 21:34
  • 8
    $\begingroup$ Since it depends heavily on the weather, (it takes very little blood to color existing water a dark red) really your answer is "how many do you want to kill?" As long as it's hundreds or more and you've got some rain showers going on, you'll be fine to have your streets running red with blood. Well, just so long as you don't kill them by strangulation or asphyxiation, I suppose. $\endgroup$
    – HopelessN00b
    Commented Apr 20, 2017 at 5:36
  • 14
    $\begingroup$ You just need one person, preferably the guard to the red paint storage (they will run both red and with blood) $\endgroup$
    – xDaizu
    Commented Apr 20, 2017 at 8:36

4 Answers 4

Reset to default
38
$\begingroup$

River method (most deaths)

Width - Pretty simple, 12$\times$4=48 so 48' (~15m for anyone not familiar with imperial.)

Length - Apparently it is a 100' (30.5m) long road.

All work in the section below is based on old, incorrect numbers. Left in to provide context for comments below. See end of blockquote for accurate numbers.

! Depth - Blood has a pretty bright, strong colour. A coating 5mm (0.2") thick would be more than enough to obscure the colour of the road. (1mm (0.04") could probably do it but I'm not sure so I'll play it safe). I assume there is no vertical slant but roads often have a horizontal slant as a flood reduction measure and the slant is usually 4%. I doubt this holds true on a road your size but I'm going to go with it just in case. The road is 48' so at 4% every foot across we go 0.04 feet down. 0.04$\times$48=1.92 so we drop by ~2' (0.5m) this means at the deepest the blood must be 2' 0.2" (0.6m) thick. An average depth of 1' (0.3m) thick

! Volume - This gives an overall volume of 48$\times 1\times$100 or 4 800 cubic feet of blood (136 cubic metres). Converted to a more standard measure that is nearly 30 000 imperial gallons (136 000 litres).

! Humans - An average adult contains up to 1.2 gallons (5.5 litres) of blood. If we completely drain every human (see @Flummox for method) we would need 30 000/1.2 = 25 000 people. We might not be able to fully drain every human as I don't know what wounds you're using. As it is you need at least 25 000 people and probably far more. I expect you will struggle to drain even a quarter of each person's blood in a street fight so you might require over 100 000 people. On the other hand I have gone for higher estimates for depth where I can so the actual number may be significantly lower. I suggest anywhere between 25 000 and 100 000 people is definitely a safe bet for this method.

More accurate numbers

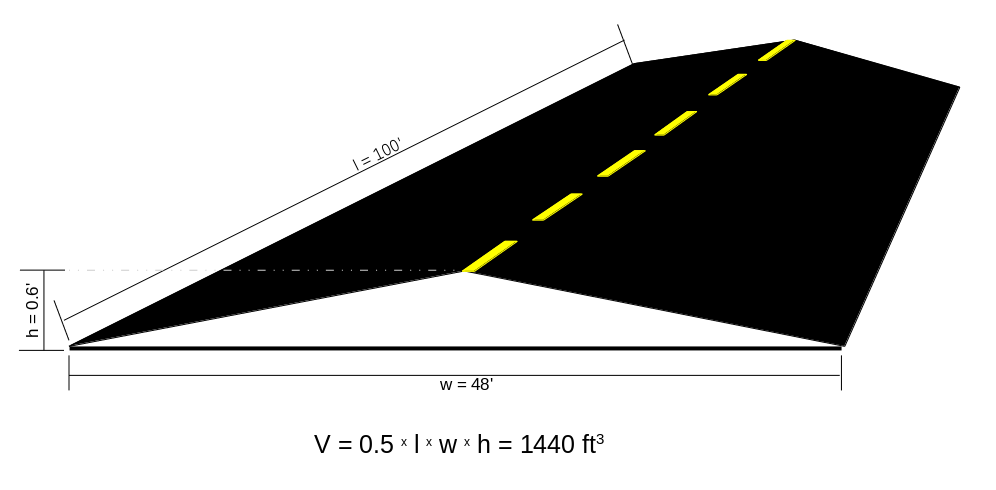
Depth - Blood has a pretty bright, strong colour. A coating 5mm (0.2") thick would be more than enough to obscure the colour of the road. (1mm (0.04") could probably do it but I'm not sure so I'll play it safe). I assume there is no vertical slant but roads often have a horizontal slant as a flood reduction measure and the slant is usually 2.5% (thanks to @AndyT). The road is 48' so at 2.5% every foot across we go 0.025 feet down. 0.025$\times$24=0.6 so we drop by 0.6' (0.2m) this means at the deepest the blood must be 0.6' 0.2" (0.2m) thick. An average depth of 0.3' (0.1m) thick.

Volume - This gives an overall volume of 48$\times$0.3$\times$100 or 1 440 cubic feet of blood (41 cubic metres). Converted to a more standard measure that is nearly 8970 imperial gallons (40 800 litres).

Humans - An average adult contains up to 1.2 gallons (5.5 litres) of blood. If we completely drain every human (see @Flummox for method) we would need 8970/1.2 = 7475 people. We might not be able to fully drain every human as I don't know what wounds you're using. As it is you need at least 7475 people and probably far more. I expect you will struggle to drain even a quarter of each person's blood in a street fight so you might require over 29 000 people. On the other hand I have gone for higher estimates for depth where I can so the actual number may be significantly lower. I suggest anywhere between 7475 and 29 000 people is definitely a safe bet for this method.

This can be reduced further if you take into account the displacement by the bodies.I can't find a value for how wide a human body but I'm going to assume it will let us fit in one row of humans on each side before they start sticking up over the level of the blood. The average human height is roughly 5' 6" (5.5' or 1.65m). In a 100' road we can fit 100/5.5=18 people per row. Two rows gives a total of 36 people. (We could possibly fit more in by curling people up but that is too complicated for me to work out.) The average human has a volume of approximately 13 gallons (62 litres)(Might not be accurate as our bodies have been drained of blood which may effect their volume.) We have 32 people so the volume of bodies is 32$\times$13=416 gallons (1890 litres). We can subtract this from the gallons of blood needed leaving us requiring 8554 gallons of blood (38 880 litres). Redoing the body count with this new maths gives 8554/1.2=7128 people as our minimum and 28 500 as our maximum so a small reduction.

Other methods (less deaths)

That is a lot of deaths. As pointed out in the comments this can be significantly reduced.

Dilution - Dilute the blood and you need less blood. Blood also maintains its colour pretty well so it can be diluted quite a lot. Using a blood:water ratio of 1:2 should be o.k reducing the minimum from 7128 to around 2376 and the higher estimate from 28 500 to 9500.

Painting - This is probably the most practical method although it won't give a river of blood and is pretty unimpressive although it will still obscure the street. By painting it on we can reduce the depth required from 1' (0.3m) to 0.2" (5mm) reducing the lower estimate to just 8 people and the higher estimate to 32.

Grinding the bodies - Further up I worked out the number if you included the bodies. That involved lying them down so reduced the number we could use. If we grind up the bodies and mix them with the blood we can reduce the blood usage significantly. This means we are effectively getting 13 gallons (62 litres) per body instead of 1.2 gallons (5.5 litres). Our sum is now 8970/13=690. This time there is no range as we get 100% of every body's volume.

Appendix

A diagram drawn up by @MadPhysicist may help clear up the first method. I used a slightly different method by using half of the height instead of putting a half in the whole sum but other than that the methods are the same.

Diagram of road

Summary Table

$$\begin{array}{|c|c|c|} \hline \text{Method name} & \text{Minimum} & \text{Maximum}\\ \hline \text{River method (no bodies)} & 7475 & 29000\\ \hline \text{River method (corpses)} & 7128 & 28500\\ \hline \text{River method (ground bodies)} & 690 & 690\\ \hline \text{River method (dilute)} & 2376 & 9500\\ \hline \text{Painting method} & 8 & 32\\ \hline \end{array}$$ Disclaimer - My maths is probably wrong. Please point out my mistakes.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Comments are not for extended discussion; this conversation has been moved to chat. $\endgroup$
    – user3652621
    Commented Apr 21, 2017 at 18:37
51
$\begingroup$

enter image description here

This is the city of Dhaka, Bangladesh last year. Streets running red with blood. There is a lot of rainwater too which hopefully is not cheating. Still pretty bloody looking.

I read that 100,000 animals are sacrificed there for this ceremony each year. Probably not all on this particular street though. I read that the city set up 1000 places for sacrifices to happen throughout the city but people still went ahead and did their sacrifices at handy street sites.

It is hard to find even one sacrifice site in the city where I live.

An article about with more pictures and some additional information:

Rivers of blood flow on streets of Dhaka after Eid animal sacrifices

Improve this answer
$\endgroup$
1
  • $\begingroup$ Comments are not for extended discussion; this conversation has been moved to chat. $\endgroup$
    – user3652621
    Commented Apr 21, 2017 at 18:37
20
$\begingroup$

First....Ewww.

Bellerophon probably has a more precise method, but here is an alternative method:

We could use the coverage of paint as a baseline. Typical household paint will cover about 400 sq feet per gallon $(9.8 \frac{m^2}l)$. It's less when the paint has a lower amount of 'solids', and blood doesn't contain titanium dioxide as a white hiding base, so lets cut that number by 1/4. now we have something to work with. 100 sq ft per gallon $(2.5 \frac{m^2}l)$. If the street width is about 48 feet $(15m)$ wide, you could get 2 linear feet per gallon $(0.16 \frac{m}l)$. The question asks to obscure the roadway, not completely inundate it.

Each human body has a bit more than 8 pints of blood, so that's about 1 gallon plus a bit, up to a little more than $(4l - 5l)$ per person.

Working it the rest of the way out, your psychopath would need 1 victim for every 2 linear feet of roadway $(1,4 \frac{persons}{m})$...and a long handled roller to make sure he covers everything evenly.

Improve this answer
$\endgroup$
10
  • 2
    $\begingroup$ Corpses aren't long handled, nor are they good rollers, but they sure are available in quantity in this scenario... $\endgroup$
    – kingledion
    Commented Apr 19, 2017 at 23:51
  • 3
    $\begingroup$ A little blood will dilute a long way and still be bright red, as anyone who has washed a small wound will know. So if it were raining ... still, Ewww! $\endgroup$
    – nigel222
    Commented Apr 20, 2017 at 12:56
  • 3
    $\begingroup$ Especially since a psychopath who uses this scientific approach to killing, will also likely use proper SI units and will thus appreciate our efforts :-) $\endgroup$
    – Falco
    Commented Apr 20, 2017 at 15:08
  • 1
    $\begingroup$ 1gal < 4L. Not sure where you get 5L. $\endgroup$
    – Mad Physicist
    Commented Apr 20, 2017 at 18:03
  • 1
    $\begingroup$ +1 for use of a reference that's already been thoroughly tested, i.e. wall paint. $\endgroup$
    – user3067860
    Commented Apr 20, 2017 at 21:08
3
$\begingroup$

There are already some excellent answers here. Let me try it from a historical perspective.

The streets run red with blood.

Is mostly used for massacres. And to get the Rivers of Blood claim you need lots of dead people. The most famous seems to be the Siege of Jerusalem (1099). At the same time there is the connotation of a heavy, brutal loss to the nation. Sometimes unrecoverable, like the Destruction of Baghdad (1258).

These horrors are not confined to the middle ages, the 20th century has more then it's fair share. When you have the Red Terror, it's not only the colour of their flag that make it so.

To answer your question, you need at least thousands of people dead or heavily bleeding to make the streets run red with their blood.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .