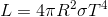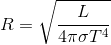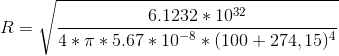Too close for comfort

Question

I'm writing a sci fi thriller and I'm including an inter-species romance scene which involves a pair of star crossed lover, a middle aged man and a mutated botfly no larger than a penny both drifting at a non-lethal distance away from the surface of Pistol Star, a blue hypergiant or V4647 Sgr after their interstellar spaceship got stranded in space during a daring exploration to the center of Milky Way galaxies.

Long story short, the mission ended in disaster and most of the crews as well as their pet companions were killed but that's not the main issue right now, I thought that the survivor and his fetish could somehow get into an outdated astronaut suit made from the 21st century A.D. due to an unforeseen circumstances before making their escape as the ship is about to explode, I just want to know how close can our main protagonist and his fiancee stay in orbit at a safe distance from the surface of the hypergiant apart from the lethal stellar wind before becoming crispy.

Let's assume that the Pistol Star is at least 100 solar mass, the human and the insect are sharing 1 spacesuit together and last but not least please provide your working to support your answer. Remember they should survive long enough to exchange their vows say between 10 to 30 minutes.

Answer 1

I am not a specialist but I can try :

Circumstellar Habitable Zone Based Answer

This answer is based on the circumstellar habitable zone. Because the Earth is in the Sun's one, and our spacesuits are efficient in this zone. So my guess is if they are in the HZ of the Pistol Star, the spacesuit should be enough to endure the heat.

To calculate the center radius of the HZ, you just have to do sqrt(Star Luminosity/Sun Luinosity), this relies on the fact that the Earth is pretty much at the center of the Sun's HZ, that the Sun-Earth distance is 1 A.U. and that the energy received from a star is inversely proportional to the squared distance from this one.

Furthermore, the Pistol Star luminosity is estimated around 1,600,000 L☉, so 1,600,000 times higher than the Sun.

So by making sqrt(1600000) ~= 1265 A.U. So the center of Pistol Star HZ is around 1.8924e+11 km. I think that a good start, you can get them a bit closer of course, because spacesuits could endure the heat a little closer than just above the Earth.

---

Edit :

Star Luminosity and Spacesuit Capabilities Based Answer

I was wondering if there is a more physics-based answer and I think I found another way to calculate this orbit distance, but I am really unsure about this reasoning.

Firstly, a classic current space suit can hold from −156 °C (−249 °F) to 121 °C (250 °F). Let's say that we want our spacesuit to be exposed at 100°C. We just have to calculate how far from the star the temperature is 100°C.

(This is the unsure part) Then, there is a formula giving luminosity of a black body (a star can be approximated to a black body) depending on its temperature and the distance from it, it is called The Stefan–Boltzmann equation , L in Watt, R in meters, T in Kelvin and σ = 5.67×10−8 W·m−2·K−4. We just reverse the equation to get the radius : .

Finally, with the data of Pistol Star, we just have to calculate. L = 1,600,000L☉= 1600000 * 3.827e+26 W = 6.1232e+32 W.

= 2.105e+14 m = 2.105e+11 km = 1337 A.U.

Less than 100 A.U. different ! (Yep 80 light-minutes is not that far :P)

But once again I really don't know if my second reasoning is well done.