Calculating Living Area on a Concentric Shellworld

Question

I'm not sure if I should be asking this here on on the Maths Stack Exchange, because this is a sort of mathematics problem but here goes:

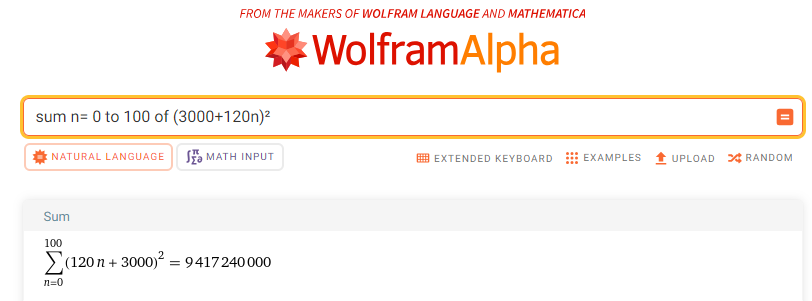
A civilization lives on multiple concentric shellworlds, each with a solid, dense core, followed by a series of shells at a given interval. For example, a Type-1-Alpha Shellworld has a solid core 3,000km in radius, and an additional shell every 120km up, so one at 3,120km, another at 3,240km, and so on, up to 100 Shells at a full radius of 15,000km.

The viability of the shells or how they work is not in question, and I wanted to calculate area to properly get some viable population numbers for such worlds (heat is not an issue).

I usually calculate living area by reverting to relative Earth values and taking the square, so at 15,000km that's roughly 2.35x Earth's Radius and so around 5.5x the living area, but the problem I ran into was the different areas within each shell; The lowest shell would only have 0.22x the living area.

The brute force solution would be to add up all the shells manually, but that's A. a pain, and B. unfeasible as shellworlds get to far larger sizes rapidly, many having thousands of shells.

My current formula is to take whatever the middle distance is between the smallest and largest shells, find that area, and multiply it by the number of total shells, but I think I'm low-balling the figure because in the Type-1-Alpha instance the middle shell has about 2x Earth's area, which is 1.78 more than the lowest shell, but 3.5 less than the highest.

Is there any value or formula or something that I'm missing when trying to average the area of these shellworlds? Pardon my shoddy maths and lack of formulas, they are not my forte.

Answer 1

Considering that probably you do not need a twenty-digit precision, you can calculate with volume.

What you need:

1. Calculate the volume of a 15000km sphere
2. Substract the volume of a 3000km sphere
3. Divide with a 120km height

Result will be the living area. As a simplified formula:

$$\frac{4\pi}{3}\frac{R^3-r^3}{h}$$

That approximates sum with integration, but again the context clearly shows that it is not a big problem here.

The result is $1.168\cdot 10^{11} km^2$, which is a good match to the wolfram result.

Note that the surface area of our Earth is $5.1\cdot 10^8 km^2$, but only about a third of it is land.

Answer 2

1.183*10¹¹ square km

The total area is $4\pi \sum_0^{100}(3000+120n)^2$.

We ask Wolfram Alpha (leaving out the 4$\pi$):

Now we just have to multiply the result by 4$\pi$:

4$\pi$ * 9.417.240.000 = 1.183*10¹¹ square km.

QED.