How deep underwater can red photosynthesis work?

Question

The Setting:

I have a binary star system.

First star: K5.2V class Orange Dwarf, 60% the mass of Sol, 17% luminosity of Sol, and at 4.5 billion years old. This star is the only one with a planetary system.

Second star: an M-Class Red Dwarf that orbits 300AU away.

The planet: 90% the mass of Earth, with an iron core to provide a magnetosphere. Orbits 0.5AU from the K5.2V star, within the habitable zone. Similar composition to Earth, though it has a moon which causes tides roughly 2.5x greater. (Orbital mechanics of the moon aren’t important here, this is just to describe the setting.)

Life forms are carbon-based, using sugar-phosphate based genetic biopolymers.

The atmosphere is mostly comparable with that of early Earth, during the Ediacaran/Cambrian.

The Problem:

I’m looking to develop photosynthetic organisms on this planet. I’ve deduced that, based off of the star’s luminosity and distance, it’s most likely that a red pigmentation would evolve for plants. This is because, as I understand, plants reflect the most intense wavelengths of light from the sun, and absorb all the other wavelengths.

Click on image for larger view.

Our star, Sol, outputs more green light, and thus plants reflect the green light to protect themselves.

The problem I’ve encountered is that, as is evident by biology on Earth, red light tends to be absorbed rather readily by water, such that many deep-sea creatures are bright red in color, simply because red light normally doesn’t shine that far down.

Click on image for larger view.

I’ve run some (admittedly slap-dash & possibly inaccurate) calculations, and have deduced that most red-based photosynthesis would be impossible below 30m (90’) underwater. By comparison, phototrophic organisms in Terran oceans are cited as being found above 150m, using green chlorophyll. That logic would indicate that most reef-building organisms on my planet would be heterotrophic - filter-feeders, decomposers, or other such organisms that obtain nutrients by consuming organic matter.

The Question:

Given the limitations stated above, how deep into an ocean would red photosynthesis be able to reach?

Answer 1

This is a Frame Challenge

And I think it's the first time I've proposed a frame challenge to a hard-science question. It feels... desiderious. Like I've lost some kind of innocence.

I understand that you want to know to what depth red could penetrate seawater to promote successful photosynthesis. My frame challenge is, it doesn't matter.

Note #1:

@ErikHall has made the claim that I'm wrong. He's incorrect, we're just looking at things in two different ways. As we work back and forth to perfect our answers, this will be a benefit to the OP and others on this issue. I'm presenting real and credible results from studies of electromagnetic transmission in the ocean. That means the results are contemplating distances much longer than the average depth of the ocean, which is 3,688 meters (3.7km). Erik's data is looking only at absolute statistics, showing that the green spectrum absorbs after 50–180 meters (approx. avg. 120 meters) and the red spectrum in 0–40 meters (approx. avg. 20 meters). Using rough averages, that's a difference of only 100 meters in depth. That's only 2.7% of the ocean's depth. But it begs the question, and it's an important one for the OP, is that 2.7% significant? Think about it this way. First the OP is talking about photosynthesis. As an example to illustrate the problem, if we assume that 90% of photosynthetic oceanic life occurs in the green spectra absorption zone, then Erik is correct in asserting that I've over analyzed the problem. (Actually, he's simply said, "you're wrong," which is, itself, wrong.) On the other hand, if we assume that the green and red photosynthetic zones represent only, say, 50% of photosynthetic life in the ocean, then I'm right. What might occur here, though, is that we discover that 90% of photosynthetic life in the ocean only exists in the top five meters of ocean water. In that case, and at this moment, both Erik and I would be wrong with one exception: I'm presenting a frame challenge that the OP's question doesn't matter. So, stay tuned, I'm doing a bit more research.

Note #2:

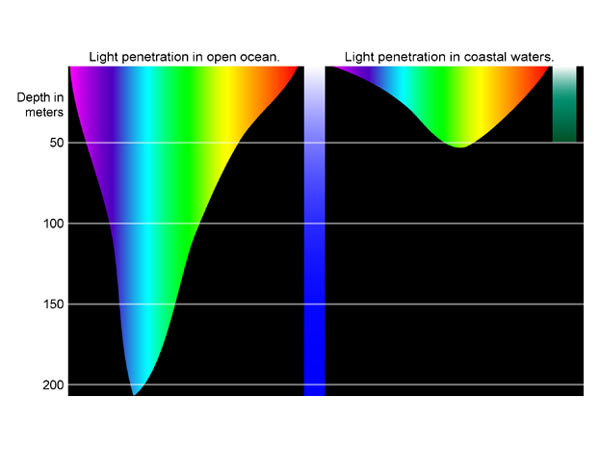
The chart from NOAA presented by Erik, when seen in its complete context, strongly supports the idea that additional research is needed. I don't know at this time if the majority of photosynthetic life in the ocean is coastal or open ocean. However, I'm impressed by the two bar graphs shown in the chart. The one demonstrates that the majority of penetrating spectra in open ocean is blue. The other demonstrates that the majority of penetrating spectra in coastal waters is green.

Note #3:

It's going to take some days to complete my research. The issue the OP has asked about is much more complex than simply asking how deep red spectra photosynthesis can occur. Indeed, it might prove to be the least relevant aspect when it comes to the potential evolution of red-spectra-photosynthetic-life. There are substantial interactions due to temperature and nutrient flow that, surprisingly, place some of the most photosynthetic productive regions at the poles and not at the equator. Indeed, it appears that deep ocean areas are almost irrelevant when it comes to photosynthetic life due to these and other issues. Therefore, the amount of data to be presented will increase considerably, but it will not change my frame challenge. It doesn't matter.

Reference #1: Propagation Characteristics of Electromagnetic Wave in Seawater Channel for Submerged Buoy

Reference #2: Propagation of Electromagnetic Waves in Seawater

The mathematics of simulating the propagation of electromagnetic waves through seawater are horrific.¹ But we can derive a simple truth, notably from reference #1, Figure #2:

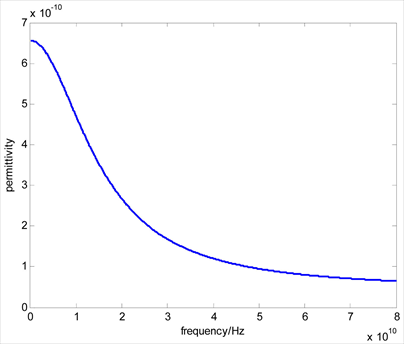
This graph is showing the change in permittivity (the "willingness" of a medium to allow something to travel through it) through a sample of seawater vs. electromagnetic frequency.

You'll notice the graph ends with a frequency of $8x10^{10}$.

• The frequency of "red" is $40,000x10^{10} - 48,400x10^{10}$.
• The frequency of "green" is $52,600x10^{10} - 60,600x10^{10}$.

Both those frequencies are way, way, way off the right edge of the chart. Why don't I care? Because the chart is flattening out such that the difference in permittivity between the red and green spectra is, for the intent of your question, zero.²

But couldn't we get a hard number?

Well... yes and no. The problem is that the word "seawater" is deceptive. The permittivity of seawater changes with

• Salinity
• Temperature
• Density (you should be thinking, "oh, great... currents and turbulence...")
• The amount and type of organic stuff emulsified in the water
• The amount and type of inorganic stuff emulsified or dissolved in the water

Therefore, any number I gave you would have a very high chance of being wrong for a location only a few kilometers away. But thanks to that graph, above, whatever that number might be, the difference in depth between green and red frequencies would be fundamentally meaningless due to the very high frequencies involved.

Conclusion

There is no useful difference between the ocean depth penetrated by green light and red light. Therefore, there is no evolutionary advantage for the development of one spectra of photosynthesis vs. another.

Answer to your question:

As deep as it does for green. But that's actually good for you. Insofar as we understand evolution, it isn't as deterministic as people like to believe. There was no guarantee that primate-based humans would be standing on the Earth today. It could have just as easily been crab-based humans. My point is, you're good to go to develop your plant life because there isn't a valid challenge against it when it comes to photosynthesis.

---

_{¹ That was a fun read, but it's dealing with mathematical formulas, laws, and models I haven't dealt with since college. Despite my background as an electrical engineer, to fully comprehend everything in that article would require me to pull at least two textbooks off my shelves. I wasn't willing to go to that extreme for this answer because the consequence is so obviously "zero."}

_{² Back when I was actively designing circuits we had to overcome problems with our simulations based on a conflict between large-numbers and small-numbers. In a nutshell, we would simulate gargantuan variations in incredibly small numbers. Which meant the gargantuaness of the variations had no useful meaning: the level of precision was cranked up too high to be useful and it was distracting (read: corrupting) the results of our simulations. We're running into that same problem here. The frequencies compared to AM radio are so large and the difference between the two frequencies so small that the resulting variation in permittivity becomes irrelevant.}

Answer 2

I disagree with the answer given by @JBH. Let's begin with the graph below.

Absorption coefficient of water of the visible region from Wikipedia.

We can perform a quick sanity check on this. According to this Graph. Red light is absorbed more than Green, blue and Violet wavelengths. Which would imply as depth increases, red objects should become black. You have done this, since you use the same image;
I'm using this because the OP has used it, but I cannot find a definitive and scientific source for it. Using Tineye to reverse-lookup the image, I discovered that it's not credible as it has been reposted many times with a variety of depths from 5 feet to 155 feet.

But, Color is strange. Very strange. Objects which are totally red ? Yeah they will turn black. But that is hardly duo to Depth. If you have Green LEDs, anything Red will also look strange. Virtually no color in nature is a pure Wavelength. They are composites because most objects are not actually 2D. Duo to stuff like Subsurface scattering, it is very hard to make something only a single wavelength or Color.

Now, the math for this is pretty cringe. But thankfully people have done it.
Basic illustration of the depth at which different colors of light penetrate ocean waters courtesy NOAA.

This graph shows you exactly what you want to know, and Imo is why JBH´s answer is not true. They say "There is no useful difference between the ocean depth penetrated by green light and red light.". Which is wrong. There is a huge difference. Green and Blue light penetrates 200 meters, Red light fizzles out at 25 meters. If that is not a "useful difference" idk what is.