Imagine if all of earth's interior were suddenly replaced with a volume of atmosphere (78% N, 21%O, etc), and by some fantastic force the crust remained perfectly rigid and stable.
My question is: if a hole, say with 200 m radius, were made through the crust, how far would light penetrate the hollow, air-filled interior? Would it reach the other end?
TLDR: Not far, only about 1/200th of the way.
We need a few assumptions here, but I think we can work this.
If all of Earth's interior were replaced with air, Earth would have so little gravity that all of the atmosphere would immediately fly off into space, so let's assume there is a brick in the dead center with the correct density to keep the mass of the Earth the same.
As we move down through the atmosphere and into the middle, the density of air is going to increase. Engineering ToolBox has a nice equation to estimate air pressure, but breaks down pretty quickly if the elevation is too large and negative, so I grabbed Jupiter's info to get a sense of the pressure, and scaled that back against the mass of Earth compared to Jupiter to get a core pressure of approx 14080391 kPa (which sounds like alot, but Jupiter is about 1000x that, so I think we're ok), and then fit a log curve to it so that we get intermediate pressures.
Here is the same graph, zoomed in to display pressures near 0 elevation.
There are some nice absorption data generated by some university researchers you can find at this link to a pdf of their paper that we can use with our newly found pressure curve and ideal gas law to estimate absorption, which we can fact check at sea level with NASA's estimate to see how close we are.
Using that data, we find that 1% is absorbed by about 22 km elevation, and we are about 31% absorbed by sea level, and NASA states its 23%, so the rough math is close enough.
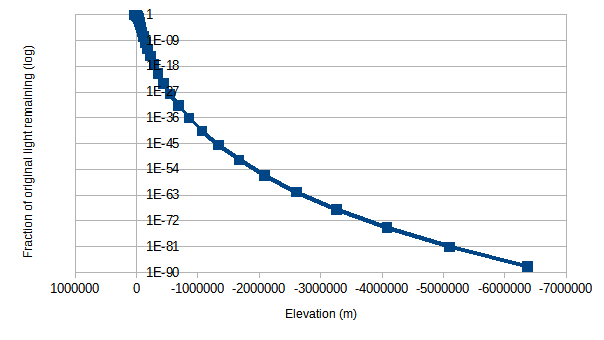
So, moving down to the interior of the earth, only 1% of the original light remains by 40km below sea level (about 0.5% of the way to the middle), only about 1 billionth of the light remains at 120 km below sea level (about 2% of the way to the middle), and only 1 in 8.7396*10^87 remains by the time it reaches the middle.
Here is a plot of the fraction of light remaining (log) vs the elevation, which shows about what we expected, that very little is absorbed at the very beginning, but rapidly ramps up as density increases.
Jumping onto the points made by @jeffronicus, @Daron and @JBH above, the vast majority of the day, no light would even illuminate the inside of the hollow Earth.
Given that the Earth's crust is approximately 20 km thick, and assuming this hole is punched on the equator to maximise the amount of sun light that gets in, only angles of incidence between positive and negative 0.57 degrees will shine into the middle of the ball through your described 200m diameter hole, which is about 4 and half minutes a day, or about 0.3% of the time.
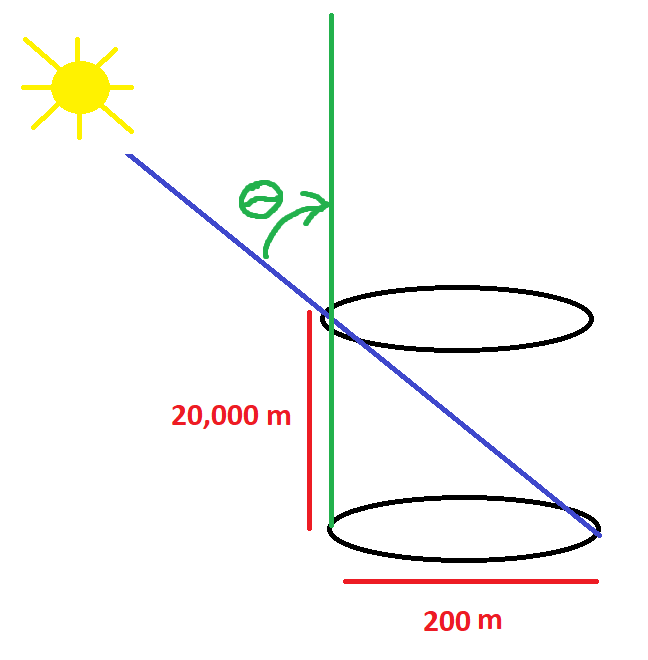
As pointed out by @AlexP in the comments, that is the maximum amount, and only happens on the equinoxes, the majority of the year the sun never passes within 0.57 degrees of zenith. Checking a table from Solar Energy Conversion (they have a table with actual values), we find the sun indeed only passes that close to zenith two days a year, once ascending, once descending, so only two days a year get any light, and only 4 and half minutes of it, so of the total time only about 0.002% of the time does the sun even illuminate some part of the bottom of the opening.
About a tenth of the way
Of course some negligible amount of light will hit the other side. But only 1% of the starting light gets more than a tenth of the way through.
Googling around I was told the Troposphere and Stratosphere contain about 99% of the atmosphere. They are about 30 miles or 50km thick. I also found the following:
The sun’s radiation must make it through multiple barriers before it reaches Earth’s surface. The first barrier is the atmosphere. About 26% of the sun’s energy is reflected or scattered back into space by clouds and particulates in the atmosphere [34]. Another 18% of solar energy is absorbed in the atmosphere.
which suggests 50km of atmosphere will absorb about 20% of the light you shine through it. In other words $0.8$ of the light gets through.
If you double the atmosphere then only $0.8^2 = 0.64$ of the light gets through. If you triple it then $0.8^3 = 0.512$ of the light gets through. At 20 atmospheres only $0.8^{20} \simeq 0.01$ or 1% gets through.
Those 20 atmospheres are about 1000km thick. In comparison the Earth's diameter is 13,000 km. So by the end we only have $0.01^{13} = \text{very small number}$ of the light left.
