21
$\begingroup$

I've been reading about stellar engines. The Wikipedia article on them says this about the acceleration they could provide to a star:

Such thrust and acceleration would be very slight, but such a system could be stable for millennia. Any planetary system attached to the star would be 'dragged' along by its parent star.

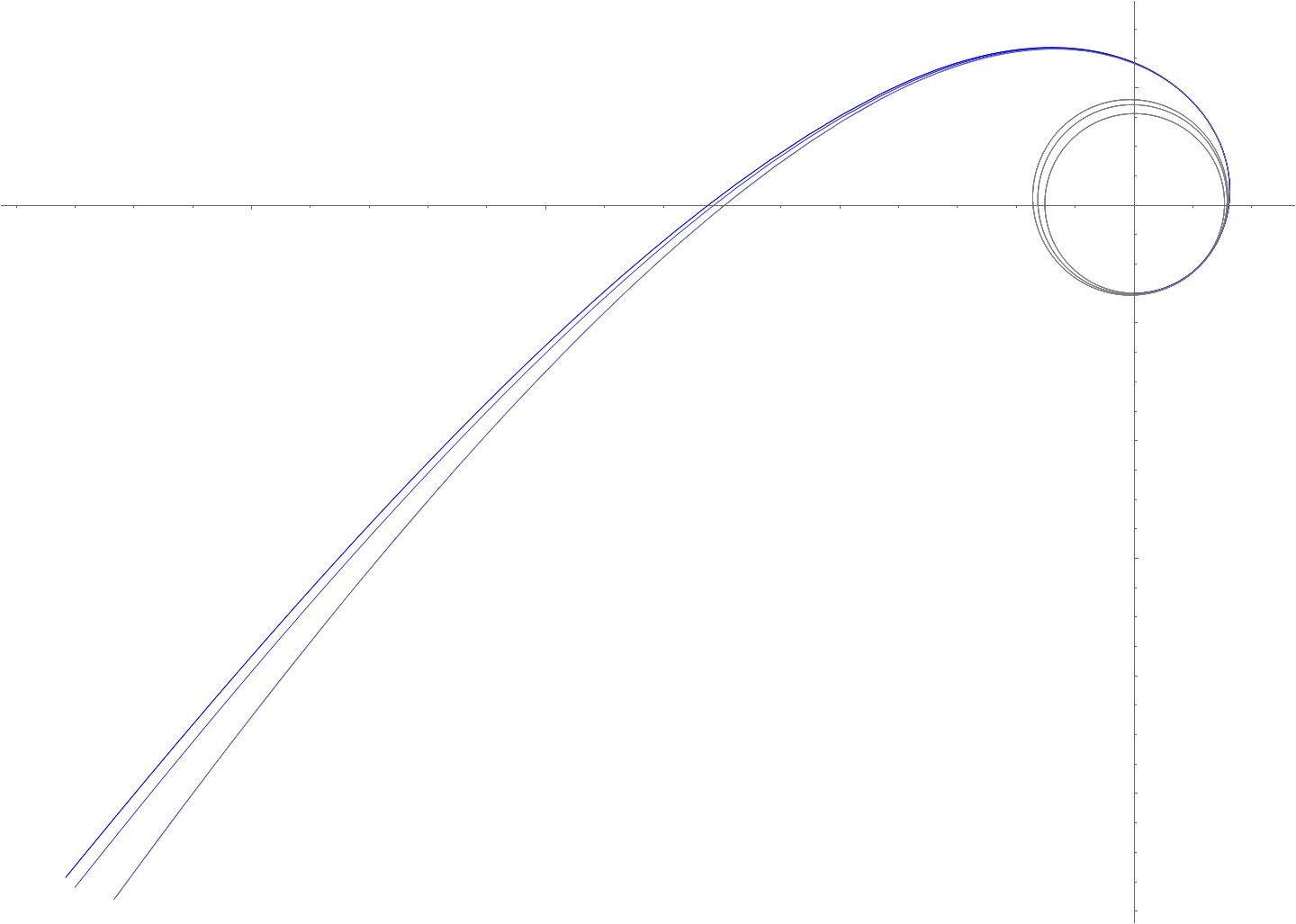
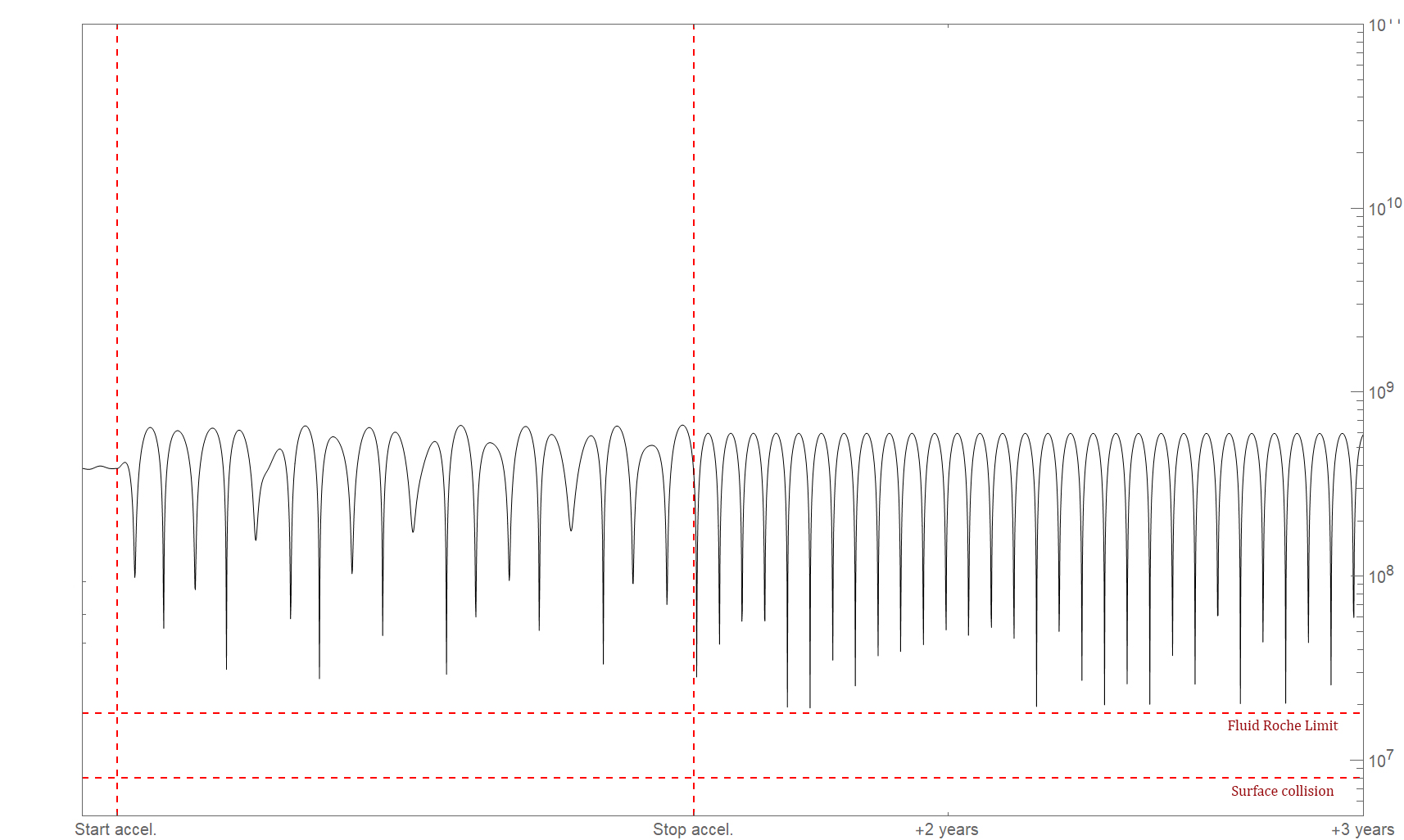
I'm writing a sci-fi novel about Earth going rogue. I would like to know if, by the same principles, if the Earth gets accelerated prograde out of the solar system, would the Moon be dragged along? I imagine so since the Earth is two orders of magnitude more massive, being the dominant mass in the Earth-Moon system.

Further details:

  • The Earth is accelerated by a mysterious force, giving it a push of ~0.025 g for twelve hours;
  • The force acts only on Earth and has a gravity gradient of 0.0001% (less tidal effect than the Sun).

I imagine a set of possibilities:

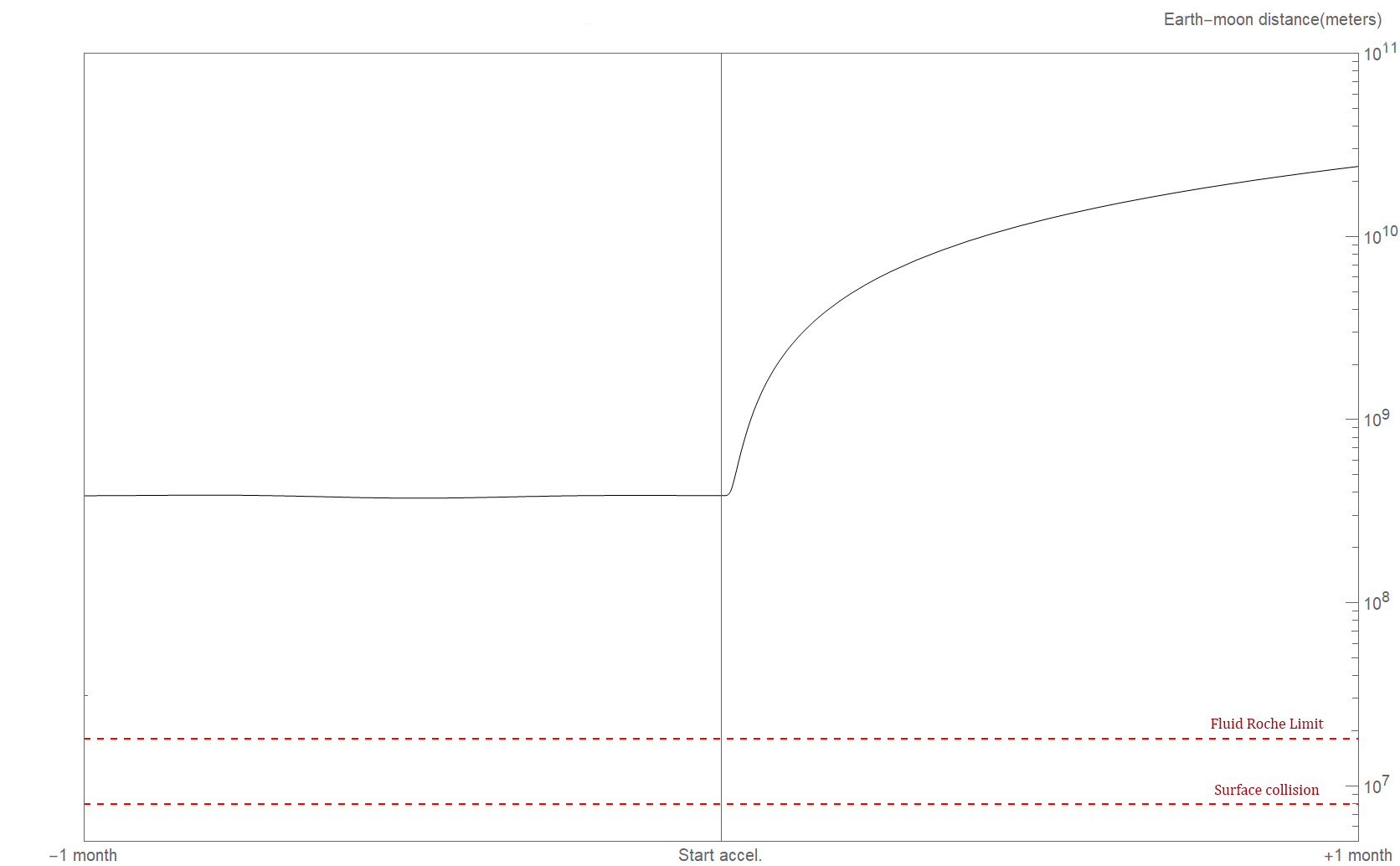
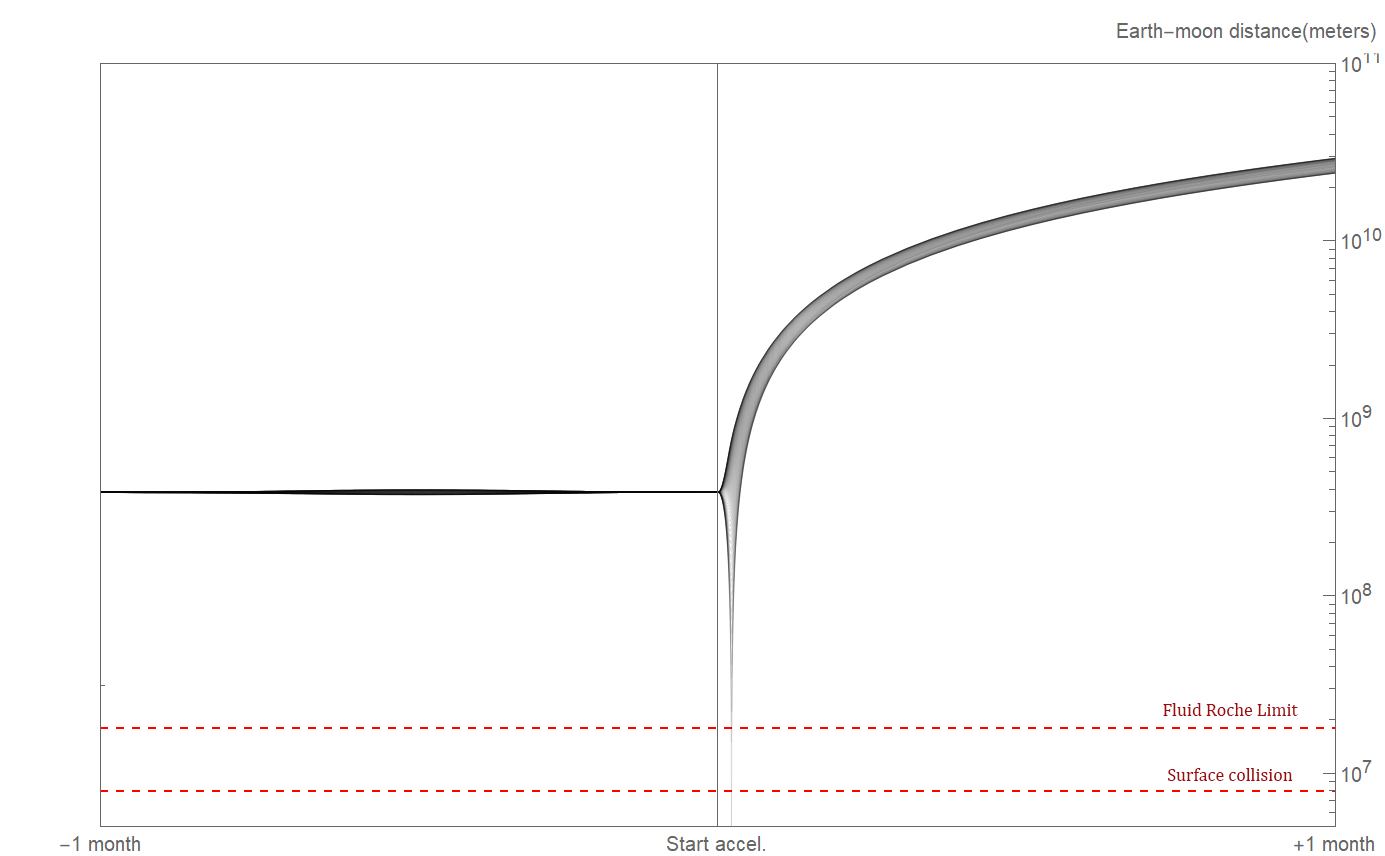
  • Small or negligible effect on the Moon's orbit;
  • Very strong effect on the Moon's orbit, changing it into a much more eccentric shape;
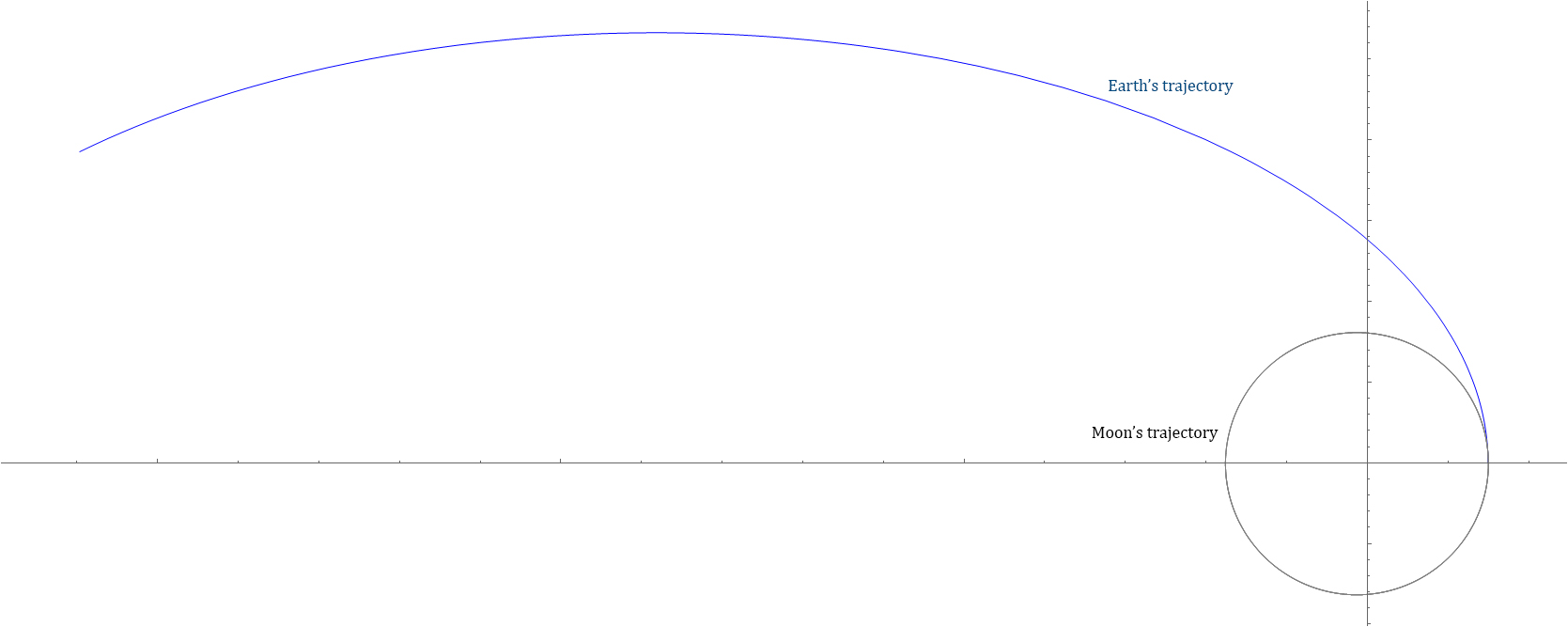
  • The Moon escapes the Earth-Moon system on a tangent - tides get weaker and weaker as she leaves;
  • The Moon does a very close flyby, leading to one devastating extinction event with earthquakes, tsunamis and volcanic eruptions of biblical proportions - then she escapes the Earth-Moon system;
  • The Moon's orbit becomes very eccentric with a very low periapsis - same as above but on infinite repeat;
  • Collision. That would be a very short and anticlimatic story;
  • ???

I don't have the math or the physics in me to figure what would most probably and realistically happen, so I'm coming here for help because you guys always make my day :)

Improve this question
$\endgroup$
12
  • 1
    $\begingroup$ Have you already done the math if 0.25g applied for 12 hours would yeld escape velocity from the Sun? (42km/s) $\endgroup$
    – Duncan Drake
    Commented Jan 25, 2021 at 17:57
  • 2
    $\begingroup$ @DuncanDrake ~2.5 m/s^2 for 12h will give a ∆v of 108 km/s. That's actually bat out of hell! I had a mistake of one or der of magnitude there, which I just fixed. 0.025 g will get close to escape speed in 12h. That's why I say approximately - just a little bit more should surely get the Earth to 42 km/s. $\endgroup$
    – The Square-Cube Law
    Commented Jan 25, 2021 at 18:05
  • 1
    $\begingroup$ tidal force 101: the moon will continue to move along in a straight path unless an external force is acting on it (Newton's law), Earth (Sun and other planets too) is (are) acting on it so it stays in orbit (actually drifting further away) but if you were to keep increasing the force like accelerating the Earth (gravity is a fictional force BTW) the moon will be perturbated. TL;DR goodbye moon ;O $\endgroup$
    – user6760
    Commented Jan 26, 2021 at 3:45
  • 1
    $\begingroup$ Losing the Moon would be the least of your worries in such a scenario. Losing the Sun is far worse, since it's directly or indirectly the source of nearly all energy on the planet. Sure you've got a little bit in the way of geothermal and nuclear energy, but not enough to sustain an entire planetary ecosystem. First thing that would happen is all the plants would die from lack of photosynthesis. Then everything that eats plants, then everything that eats things that eat plants, etc. Earth would be a lifeless frozen wasteland in a matter of weeks. $\endgroup$
    – Darrel Hoffman
    Commented Jan 26, 2021 at 15:02
  • 2
    $\begingroup$ @DarrelHoffman that's the plot of my novel :) I have a couple questions about that: Which countries/organizations are most likely to ensure survival on a freezing Earth? and Can we live off hydrothermal vents? $\endgroup$
    – The Square-Cube Law
    Commented Jan 26, 2021 at 15:13

9 Answers 9

Reset to default
10
$\begingroup$

Farewell Luna. But, there might be a way...

I simulated the Sun-Earth-Moon system to determine the trajectories of Earth and moon. With your given acceleration $a=0.025g$ and $T_{accel}=12\ \text{hours}$, the Earth categorically, and quickly, leaves the moon behind. One parameter we have to play with is the angle of the relative position of the moon, $\phi$, at the time when acceleration begins. It is simplest to see in a diagram.

enter image description here

Earth is orbiting CCW around the sun, and the moon is orbiting CCW around the Earth. If $\phi=0$, then the Earth and moon are moving in the same direction when acceleration begins. If $\phi=\pi$, then the Earth and the moon are moving in opposite directions when acceleration begins. Here is an example trajectory with $\phi=0$, over five years. It's in the reference frame of the sun, which is at the origin of axes:

enter image description here

We see the moon continues to orbit the sun, and is very much left behind. It is interesting to look at the Earth-Moon distance over time. Here is a plot with $\phi=0$:

enter image description here

For $0 \leq \phi < 2\pi$, I overlaid the Earth-moon distance plots. Each thin line on the plot below is a distance time graph for a particular $\phi$.

enter image description here

We see that most trajectories (dark lines) have the Earth-moon distance increasing forever. A few lead to close encounters or impacts. After a close encounter, the moon is still lost. The range of $\phi=\frac{\pi}{40}(10 \pm 1)$ lead to approaches below the fluid Roche limit of the moon. Impact/ closest approach happens about 16 hours after the start of acceleration.

From this plot we learn: Independently of the initial configuration ($\phi$), the Earth either leaves the moon behind, or collides with the moon.

...to save her

Maybe you want to keep the moon around? The acceleration would need to be drastically reduced. In order to put the Earth on a hyperbolic trajectory, it will also need to be applied for much longer. Here are 4 trajectories overlaid ($\phi=0,\ \pi/2, \ \pi , \ 3\pi/2$), with $a=0.025g/600$ and $T_{\text{accel}}=500 \ days$.

enter image description here

For the trajectory with $\phi \sim 3\pi/2$, here is the Earth-moon distance time graph:

enter image description here

Other values of $\phi$ still lead to the moon being lost. When the moon is retained, the lunar period is reduced to about 20 days, and the lunar orbit is highly eccentric. At perigee, the moon would appear huge, with an angular diameter of about $5^{\circ}$; ten times what we are used to!

From this we learn: the forces and timescales in the question are two or three orders of magnitude away from that required to retain the moon, and even then, the start of the acceleration needs to be at a (somewhat) specific time.

Improve this answer
$\endgroup$
3
  • $\begingroup$ In which reference frame is the first chart displayed? $\endgroup$
    – L.Dutch ♦
    Commented Feb 2, 2021 at 4:09
  • 1
    $\begingroup$ Welcome to World Building :) and what an amazing answer! $\endgroup$
    – The Square-Cube Law
    Commented Feb 2, 2021 at 8:44
  • 1
    $\begingroup$ @L.Dutch-ReinstateMonica The trajectory plots are in the frame of the sun. @ TheSquare-CubeLaw, thank you! $\endgroup$
    – Sal
    Commented Feb 2, 2021 at 11:39
27
$\begingroup$

You're going to lose the Moon.

At the Moon's current distance, the Earth's gravity can only change its velocity by $0.002m/s^2$

The mentioned acceleration of about $0.25m/s^2$ dwarfs that, and if at any time during this acceleration, the Moon's relative velocity to Earth exceeds Earth escape velocity for its distance, Earth loses it. At a distance of 384,000 km, Earth Escape velocity is about $1.44 km/s$.

Earth can't drag the Moon hard enough to catch up, and you're planning to put more than 10km/s on the Earth. It's gone.

Improve this answer
$\endgroup$
11
$\begingroup$

Goodbye, moon!

The moon's centripetal acceleration is always pointed at the earth, and has a value of just 0.0027 m/s^2. If you accelerate the earth in the opposite direction at 0.025g (0.245 m/s^2), the earth will move away from the moon with a net acceleration of 0.242 m/s^2. As the earth moves further away, the force of gravity lessens, resulting in a larger net acceleration, only ever increasing the distance between the earth and the moon. The earth's pull on the moon is not strong enough to overcome the push you're imparting on the earth itself.

This covers the simplest situation of accelerating directly away from the moon. One could also change the direction and timing of acceleration to generate a collision between the earth and the moon, or a close flyby. I suspect you could do some interesting things to slingshot the moon in certain ways, but I don't think it'll be possible to keep the moon in a stable orbit throughout the earth's acceleration.

Improve this answer
$\endgroup$
4
  • 2
    $\begingroup$ Could a close flyby be used to slingshot the moon in the same direction we're accelerating the Earth? $\endgroup$
    – Criticizing Israel not allowed
    Commented Jan 26, 2021 at 19:02
  • $\begingroup$ @user253751 Possibly, but it'd be a heck of a shot to get it just right, and I'm not 100% sure that the earth wouldn't just whiz past the moon without making much of a difference. The earth will approach the moon with ~10km/s of relative velocity, and a slingshot could lessen than by transferring some of the earth's momentum to the moon. But unless you can get that relative velocity below about 1.4km/s, the moon isn't sticking around. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 26, 2021 at 21:52
  • $\begingroup$ if you get the moon to go, say, 8km/s straight ahead, maybe the Earth could catch up to it later because the Earth continues accelerating. $\endgroup$
    – Criticizing Israel not allowed
    Commented Jan 27, 2021 at 20:21
  • $\begingroup$ @user253751 According to the question, the earth only accelerates for 12 hours. With the given parameters, the earth finishes accelerating when it's about 2/3 of the way to the moon, which is almost surely too far to slingshot the moon. The slingshot would have to occur after the acceleration is done, so there would be no catching up to it later. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 27, 2021 at 20:27
9
$\begingroup$

Depends on direction of thrust and relative position of bodies

Firstly, the Earth-Moon system will definitely be disturbed. The most likely scenario is ejection of the moon, though collision is possible if you happen to... well, accelerate the Earth into the Moon.

You are applying the force to Earth only, which means that you are disturbing the parent-satelite system by adding an external force to one of the bodies only. If you wanted to keep the Moon in current orbit, you'd need to accelerate the system as a whole. Otherwise you are changing the relative velocity of the two bodies. As a simplification that might be easier to imagine - accelerating the Earth by X km/s in a particular direction should effectively be the same as accelerating the Moon by x km/s in the opposite direction - what matters is the relative change in velocity.

As you're accelerating the Earth (by your calculations) to 42km/s that means about 12km/s increase in speed over 12 hours. With a Moon orbital velocity of about 1km/s that change means that Moon's velocity with regards to Earth is anywhere from 13km/s (well over Earth escape velocity) to 11km/s retrograde (Moon's orbit is reversed). EDIT: Correction regarding escape velocities. At the escape velocity of the Moon from its current orbit would be about √2*current orbital velocity. Which will be about 1.44km/s. Hence, the Moon will be ejected.

And of course accelerating directly into the Moon (which will move about 1/60th of its original orbit over the course of Earth's acceleration) will also lead to a collision.

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ Interesting fact, the escape velocity from any circular orbit around any body is sqrt(2) times the current orbital velocity. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 26, 2021 at 13:50
5
$\begingroup$

Probably not with your criteria that the force only affects the Earth, for 12 hours, and at 0.025g. Others have explained well why that wouldn't work. We'd leave a lot of satellites behind as well. If the force affected the Earth/moon system, then sure.

If the force only affected the Earth, it would have to be controlled to keep the moon with it. If you have a force capable of accelerating the Earth at 0.025g, you could move it into a position so that the moon is heading straight for it and in the direction you want to go. Start accelerating away so that it isn't close enough to ruin the Earth. If the moon were only 1/3 as far as it is today, you could accelerate Earth at 0.0245g away from the moon, and the moon would be accelerating towards the earth at the same rate. The tidal forces of the Moon on the Earth would be 9 times as strong.

Of course when the acceleration of the Earth stopped, you'd need to do some maneuvering to get the moon back into a stable orbit to keep them moving through space in a happy little system.

Improve this answer
$\endgroup$
3
$\begingroup$

There should be a way to do it without losing the Moon, but there would be a very narrow range of timing. If the acceleration is started just right before the Moon is in the part of its orbit away from the sun, the Earth would come towards the Moon. If it just passes the Moon, the distance could be small enough that the gravitational attraction comes close to matching the acceleration.

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ There's exactly one spot where the moon could sit behind the earth and get pulled along by gravity at the same rate the earth is being pushed. The problem is that there's no way to get the moon into that spot without pushing it. If the moon isn't close enough, it won't get pulled enough and it'll just drift away. If it's too close, it'll get pulled too hard and crash into the earth. The moon has to sit at an unstable equilibrium point, and there's no way to get it there without putting it there yourself, since the moon only ever naturally moves away from the equilibrium point.. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 26, 2021 at 14:03
  • 1
    $\begingroup$ You would need the Earth-Moon attraction to precisely match the Earth's acceleration, with the Moon at a pretty close distance (which already could not work, because of reasons). Also the magic field should unilaterally neutralize the Moon's attraction of the Earth, otherwise disaster would ensue (think The Incredible Tide, but written really, really large. In molten lava). The additional problem is that when the magic ceases, the Moon is murderously close to the Earth, and if that is now magically a stable orbit, as it needs to be... $\endgroup$
    – LSerni
    Commented Jan 26, 2021 at 15:20
3
$\begingroup$

You can do a rough calculation for the general case by calculating the Moon's kinetic energy with the Earth at rest, and let that be P. The Moon gravitational potential energy in respect to the Earth can also be calculated and let that be U. From the virial theorem stability yields 2P + U = 0 (approx).

You can approximate by ignoring the drag effect of the Earth on the Moon during the Earth acceleration phase (this could be roughly estimated however), and at the end of the acceleration you can get the new Earth-Moon distance and the new Earth speed. That allows you to calculate the new values of P' and U'.

Or you can also apply the equations to calculate the characteristic energy of the Moon after the acceleration, assuming neither distance nor position change during the acceleration (i.e. the Earth is teleported at the acceleration-ending point with the final velocity in zero time).

In both cases you'll find the same result - the Moon is lost.

(This of course assuming the Earth doesn't collide with the Moon)

Improve this answer
$\endgroup$
1
$\begingroup$

Answer: No, we loose the moon and all satellites too.

Consider that your moving of the planet will also cause all LEO satellites to enter the atmosphere and burn up in first few hours. ISS is dead too, unless the astronauts take the life-raft down to earth in the first few hours.

The only satellites to survive physically would be in Geostationary or high graveyard orbits, and I suspect they'll be left behind as well, eventually to orbit the sun or maybe the moon in a very wide circle, which itself will have a vector approximately parallel to the solar plane, but its direction depends where the moon was in its orbit as the earth left.

In a perfect world, the moon would end up in orbit of the sun, but its much more likely to be going out or toward the sun.

Improve this answer
$\endgroup$
0
$\begingroup$

I think that the answer is that the moons orbit would be changed into a more eccentric orbit, since the gravity of earth will poon on the moon and she will come on our way, but the centrifugal force will make it stay in orbit, although maybe more eliptic. This, of course, is only if the earth were to speed away slowly. If it were to go away faster, the moon would stay behind.

Improve this answer
$\endgroup$
7
  • 3
    $\begingroup$ If you accelerate the earth fast enough, the moon will just get left behind. It's true that the earth will always pull on the moon, but the two bodies only get further apart if the 0.025g push on the earth is stronger than the earth's pull on the moon. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 25, 2021 at 19:30
  • 1
    $\begingroup$ @NuclearHoagie You're assuming that the direction the earth is accelerated is away from the moon, if instead, the earth moves closer to the moon, then the gravitational pull would become stronger and the moon could be dragged along, and it's possible that the answer's result could happen (though it's not guaranteed for the reason you stated). $\endgroup$
    – Mathaddict
    Commented Jan 26, 2021 at 16:57
  • 1
    $\begingroup$ @Mathaddict Even if you move the earth closer to the moon, you at some point move right past and start to increase the distance. You can either continue to close the distance (collide) or increase the distance (leave behind), but there's no way for the earth to get closer to the moon and then just stop, as it's an unstable equilibrium point. If you're too far, you get further, if you're too close you get closer. The earth and moon would have to have zero relative velocity in exactly the right configuration, but there's no way to get to that configuration while having zero relative velocity. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 26, 2021 at 17:07
  • 2
    $\begingroup$ @NuclearHoagie You don't need to have zero negative velocity, you just have to be close enough to the moon after the 12 hours the earth is accelerating (which only increases its orbital speed by ~30%) so that the moon can avoid being lost entirely from orbit, and can eventually catch up after the earth is done accelerating. $\endgroup$
    – Mathaddict
    Commented Jan 26, 2021 at 17:20
  • 2
    $\begingroup$ From an escape velocity perspective, if the earth-moon relative velocity exceeds 1.41km/s (with the moon at its current distance), the moon will escape and never come back. In this scenario, the earth is moving at about seven times that speed before it's covered even 10% of the distance to the moon, far faster than even the increased escape velocity at the lower radius. There is simply no way to keep the relative velocity below 1.41km/s when the earth is gaining 10km/s of velocity, no matter where you point the acceleration vector. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 26, 2021 at 18:26

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .