A world with 1/3rd gravity but 1/2 atmospheric pressure. Would it be easier to fly?

Question

Let's say on an alien planet called Thindor, the surface gravity is 3.27 m/s² (which is 1/3rd of Earth's). But the atmospheric pressure is only 50 kPa (which is 1/2 of Earth's). Assume the same 20/80 ratio of oxygen/nitrogen in the atmosphere, and the same air temperature.

The question is, Is it easier to achieve powered aircraft flight in this world?

My instincts say yes, because gravity is one of those exponential things (and pressure seems to be more linear in the equations I've seen). HOWEVER, aircraft engines need to breathe oxygen from the atmosphere (and there is only half the oxygen flow available), plus air cooling would be reduced too. And besides, propellers and jet engine compressors basically take the existing air and push it backwards, so I'm thinking thrust might be reduced by 1/2 as well.

So is it easier or harder to fly with conventional aircraft?

Answer 1

Yes - it is easier (all other things being equal)

If you want the entire derivation of my below summary, please read this previous answer of mine.

Producing Lift
The ease of flying (overcoming weight with lift) = f($\frac{\rho}{g}$) and $\rho$ = f($\frac {p}{T}$).

Overcoming Drag
The drag equations are identical to the lift ones after swapping the correct coefficient so you got the same forms and drag = f($\frac{\rho}{g}$) and $\rho$ = f($\frac {p}{T}$).

However, thrust is produced by sucking in atmosphere and expelling it at higher velocity (or pressure - but this is less efficient). It is most simply approximated as Thrust (T) = f($\dot{m}$). $\dot{m}$ is known as mass flow rate and $ \dot{m}$ = f($\rho \times v$).

Since you'll need T = D the atmospheric density ($\rho$) on each side cancels out. As long as there's enough chemical to burn (fuel or oxidizer) and introduce energy into the working fluid (which is the atmosphere), thrust production isn't an issue.

Your Planet
Assuming your planet has the same temperature as Earth, then it would be about $ \frac {1 \div 2}{1 \div 3} = 1.5x $ as easy (meaning it is easier) to fly on your planet than it is on Earth.

Titan as an Example
One other note, if your world possesses a reducing atmosphere (hydrogen, methane, ethane, etc.), then your "air breathing" aircraft would carry an oxidizer (like oxygen) and use the "fuel" the atmosphere provides. This sort of configuration would work great on a body like Titan.

Titan's properties:
Gravity ~ 1/7 Earth's
Pressure ~ 1.4 Earth's
Temperature (K) ~ 1/3 Earth's
Density ~ $ 3 \times 1.4 = 4.2$ Earth's
Ease of flying = $ \frac{4.2}{1/7} = 4.2 \times 7 = 29.2 $x

Flying would be 29.2 times easier (much MUCH easier) on Titan than on Earth.

Answer 2

According to Wikipedia (which is citing a textbook I do not have, but the equation looks accurate), the upward force on an aircraft is:

$$L=\dfrac{1}{2} \rho \nu^2 A C_L $$

Of these, $\rho$ is directly proportional to pressure by the ideal gas equation, which is a good estimate at around 1 atm:

$$ \dfrac{P}{RT}=\dfrac{n}{V}=\rho$$

Combine these and you get:

$$F_L=\dfrac{1}{2} P \dfrac{\nu^2 A C_L}{RT}$$

I will note that according to the same Wikipedia page, $C_L$ contains the Reynolds Number. I do not have experience dealing with such in the context of compressible gases, but some (fairly questionable) sources I find online suggest $Re$ is not greatly influenced by pressure. As such, I am assuming $C_L$ is constant between both cases.

As you likely know, the force of gravity is simply $mg$. As such, we can calculate a generic measure of the lift force vs. the force downward:

$$\dfrac{F_L}{F_g}=\dfrac{\dfrac{1}{2} P \dfrac{\nu^2 A C_L}{RT}}{mg}∝\dfrac{P}{g}$$

To get the change in these between your two cases, using the proportionality:

$${\dfrac{F_{L,2}}{F_{g,2}}}-{\dfrac{F_{L,1}}{F_{g,1}}}∝\dfrac{P_2}{g_2}-\dfrac{P_1}{g_1}=\dfrac{0.5}{1/3}-\dfrac{1}{1}=1.5-1=0.5 $$

This shows that there will be a greater ratio of lift to gravity in your hypothetical case of 1/3 g and 1/2 pressure. Meaning, if we can assume everything else remains constant, it will be easier to fly at the same speed.

Now, the issue of attaining the same speed is more complex. If we're talking about propellers, it would be substantially harder to generate forward speed. Jet engines, on the other hand, already compress air - they would require a doubled compression ratio. I am not sure how to go about calculating the weight increase of the plane with respect to the lowering of pressure. However, two other factors make me question if this is that important:

1. Drag is proportional to density (and thus pressure) and proportional to the square of velocity. Ergo, it would take about half the forward force to keep a plane in the air at half pressure. If we assume halving the pressure also halves the engine performance, these two cancel, and we're left with the answer above.
2. The answer I gave indicates that the lift to gravity ratio increase by 50%. If you look at the final calculation, 2/3 of the lift will attain the same lift:gravity ratio as the original case; ergo 82% of the speed will give the same lift.

Based on these observations and assumptions, I conclude it would be easier to fly in your theoretical conditions than on Earth.

Answer 3

Think about one planet: Earth and Earth 7 miles up. The gravity is roughly the same in both places, but the atmospheric pressure is 75% less 7 miles up. Since long-haul jets fly 7 miles up to reduce fuel costs, one can conclude that halving the atmospheric pressure alone would make it easier to fly. The 1/3 gravity is a bonus.

Answer 4

I'm attempting to answer my own question, so please point out anything that I might've done wrong since I am not an aircraft engineer.

The first thing I do is simplify it to this: The same airplane taken to 2 different environments. In which environment does it fly better? Note this is not necessarily equivalent with the general question, because an airplane designed/optimized for my environment will be a different craft, if for no other reason than the wings and structural loads can be reduced since it only needs to hold up 1/3 weight. More on that later.

Let's call the first environment Earth, and the second environment Thindor. So Thindor has 1/3 the surface gravity of Earth, 1/2 the air pressure, but the same air composition and air temperature.

$$L = 1/2 \cdot \rho \cdot v^2 \cdot A \cdot C_L $$

Now using $V \cdot P = n \cdot R \cdot T$ and $\rho = \frac{P \cdot M}{R \cdot T}$, we see that density and pressure are exactly linearly related given the same temperature (and same volume, same n, and same universal gas constant lol).

In a 1/3 gravity environment, the craft only needs 1/3 of the lift, and the density is only 1/2. So:

$$1/3 \cdot L = 1/2 \cdot 1/2 \cdot \rho \cdot v^2 \cdot A \cdot C_L$$

This is where my lift analysis differs from the previous two answers

A and $C_L$ are the same, but the same airplane is not necessarily moving at the same airspeed to get 1/3 of the lift. Since we've changed lift and density, kept the same A and $C_L$...but the equation is not balanced yet (because we've taken 1/3 of the left side but only 1/2 of the right side), the only other thing we have left to play with is v.

(there's room for confusion here because I said "all else being equal". I guess that's not a well-defined phrase after all. If you think about it, all else can't be equal. We've already established that air density, for example, is not equal in both environments, even tho the only 2 original parameters i changed was gravity and pressure.)

In order to balance it, we need to make 1/2 become 1/3. So $1/2 \cdot x = 1/3$. x = 2/3. But we have to put this inside v squared. Meaning we need $ \left( \sqrt{2/3} \cdot v \right)^2$. So the factor is now approximately 0.816.

$$1/3 \cdot L = 1/2 * 1/2 \cdot \rho \cdot \left( \sqrt{2/3}*v \right)^2 \cdot A \cdot C_L$$

So we only need 81.6 percent of the airspeed to achieve the necessary lift. This means our engines can be a little less powerful.

But there's more, because drag has changed (less drag means even less engine power needed). Here's the drag equation I'm using:

$$D = 1/2 \cdot \rho \cdot v^2 \cdot A \cdot C_D $$

(Wow that looks almost exactly like the equation for lift!? The only difference seems to be the coefficient of lift has been replaced with a coefficient of drag).

Anyway in both environments, A and C_d should be the same, but Thindor density is 1/2 and Thindor v is only 81.6% of Earth v.

$$D_{Thindor} = 1/2 \cdot 1/2 \cdot \rho \cdot \left( \sqrt{2/3} \cdot v \right)^2 \cdot A \cdot C_D$$

So 1/2 and $\sqrt{2/3}$, multiplied together, show that the drag on Thindor is now only 40.8% of the drag on Earth!

I believe this means the required thrust is only 40.8% of the airplane's thrust on Earth.

Now Begin Engine Analysis

I'm not even sure where to begin, and we'd probably have to do a turboprop example (propeller) and a turbofan example (jet engine). ...Not sure how to begin either one...but I know both of them breathe oxygen.

I suppose we can make the assumption that half of the available oxygen will produce half engine power and therefore half thrust. But 50% is greater than 40.8%. Therefore, the same craft can fly at Thindor, with 50/40.8 = 1.225 better efficiency?

ON TOP OF ALL THIS, remember that a craft built on Thindor will have less deadweight, because it doesn't need to be as structurally strong. The wings, for example, can be less strong since they're only holding up 1/3 as much as the weight. Same thing for landing gear and fuselage, and empennage too since torques are less from control surfaces in half air pressure. So now that we're not dealing with the same airplane anymore, we can make ours lighter, meaning Thindor lift can be even less than 1/3 Earth lift, meaning airspeed can be even less, and drag less, and engine power requirements are even less...

So I have to lean towards a positive answer. Yes, it is easier to fly at Thindor.

Can anyone point out anything wrong with my analysis? Because it doesn't agree with the original two answers, both of which got a 1.5 lift-to-gravity ratio and didn't change airspeed (v). Mine, however, got a 1.225 improvement in efficiency.

Also, can someone please edit my equations, because I don't know how to make them look fancy in the right font with subscripts and superscripts. EDIT: thanks Jim2B

Answer 5

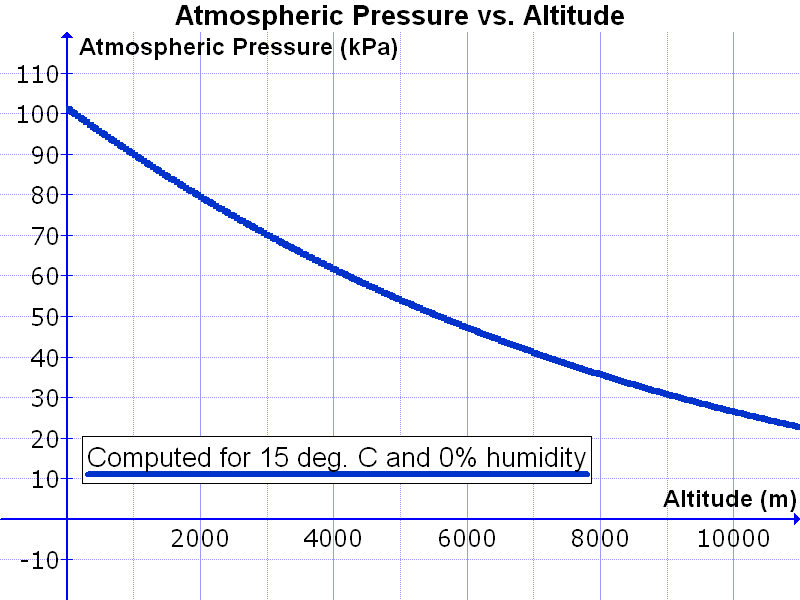Mr Baloney had the right idea, I'll give a somewhat more specific answer. Is there anywhere on earth where the air density is 1/2 that of sea level? Of course. From http://en.wikipedia.org/wiki/Atmospheric_pressure#/media/File:Atmospheric_Pressure_vs._Altitude.png pretty much anywhere at an altitude of about 6 km will do. Any aircraft which operates at this altitude on earth will do just fine on Thindor. No only that, but since it weighs 1/3 of what it does on earth, it can now carry twice its weight in cargo. Alternatively, it can have 3 times the mass it does on earth and still fly. This means it could be built out of much simpler (but heavier) materials, and still get off the ground.

Answer 6

Okay but when you assume the same athmospheric composition, and therefore also mass of any given quantity of air, you can't just say "gravity is such, AND/BUT air pressure is such", you have to calculate air pressure (at any given height) from gravity, because it is dependent on how much air is above it in the athmosphere, which in turn is dependent on how much air the planet can hold by ist mass/gravity. Sorry, I can't seem to find how to do that just this Moment, (which is why I can't even tell if that 1/3 - 1/2 Thing you started with is maybe correct), but I'm quite sure that is how it works. Unless you factor in Magic/some weird substance or structure that stably exists in the upper athmosphere/a mechanism by which gravity affects gases differently than solids...