Short answer:
There is a difference between a planet's sidereal day, the length of time that the planet rotates 360 degrees relative to distance stars and galaxies, and that planet's synodic day, the period between the planet's own star or sun appearing in the same position relative to a point on it surface two successive times, such as the period between too successive midnights at that point.
And I think that it is highly unlikely that any habitable planet could have a sidereal day
longer than its orbital period around the star or year, and very unlikely that any habitable planet could have a year more than about half of ten Earth years long.
Thus it appears highly unlikely that a habitable planet could have a sidereal day as long as 3,768 Earth days long.
Fortunately, what you need for your story is a planet with a synodic day, the period between two successive midnights or dawns in the same location, that is 3,768 days long.
And in my opinion, a synodic day 3,768 Earth days long can happen even if the sidereal day and the year of the planet are only a fraction as long as 3,768 Earth days. A synodic day 3,768 Earth days long can happen if the sidereal day and the year of the planet are almost exactly the same length, so that the apparent position of the sun in the sky of the planet appears to move only about 0.0955414 of a degree every Earth day, or 0.0039808 of a degree every Earth hour, etc., etc.
Long Answer:
Part One, life on a planet with a long day:
You can have a planet with a synodic day infinitely long, if the planet is tidally locked to its star so that one side always faces the star and one side always faces away from the star. And some planets are known to have days shorter than one Earth day.
Thus a synodic day ten Earth years long is perfectly possible physically.
What about the planet being habitable? The longer the day on the planet lasts, the hotter it will get on the day side, and the colder it will get on the night side. There is some fear that when the length of a planet's day gets too long, all the water on the day side will evaporate and the water vapor will flow to the night side, condense, and freeze.
If the planet is tidally locked, the water may all end up as ice on the night side and life may be impossible on the planet.
However:
This pessimism has been tempered by research. Studies by Robert Haberle and Manoj Joshi of NASA's Ames Research Center in California have shown that a planet's atmosphere (assuming it included greenhouse gases CO2 and H2O) need only be 100 millibars (0.10 atm), for the star's heat to be effectively carried to the night side.[77] This is well within the levels required for photosynthesis, though water would still remain frozen on the dark side in some of their models. Martin Heath of Greenwich Community College, has shown that seawater, too, could be effectively circulated without freezing solid if the ocean basins were deep enough to allow free flow beneath the night side's ice cap. Further research—including a consideration of the amount of photosynthetically active radiation—suggested that tidally locked planets in red dwarf systems might at least be habitable for higher plants.[78]
https://en.wikipedia.org/wiki/Planetary_habitability#Size1
So if a tidally locked planet might be habitable according to some studies, a planet with a synodic day 10 Earth years long might possibly also be habitable, since conditions would be a little more Earth like on that planet.
I note that if the day lasts 10 Earth years the water on the day side might all evaporate and blow to the night side and freeze. Thus thee would be no liquid surface water on the day side for plants, animals, and the natives to use. Except that at a specific time of day, sometime in the morning, as that region warms up, ice exposed to the sun will melt and become liquid water for a while before evaporating. Plants and animals will flourish while there is liquid water, and then die, leaving seeds and eggs, or go dormant, when it gets hot enough for the water to evaporate. Or the animals could follow the sun and the melting ice.
That provides a motive for the natives to migrate and keep the sun in the same relative position - they will die of thirst if they stay in the same place, and their domestic animals or prey species will die of thirst, causing them to die of starvation even if they save a little water to drink.
Part two, how long can the year of a habitable planet be?
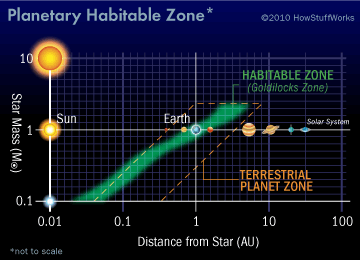
For various reasons, not all stars are capable of having habitable planets in orbit around them. If a star can have habitable planets in orbit around it, planets can only have the right temperatures to be habitable within the star's circumstellar habitable zone.
To find the inner and outer edges of a star's circumstellar habitable zone, one can multiply the inner and outer edges of the Sun's circumstellar habitable zone by the square root of that star's luminosity compared to that of the Sun.
Unfortunately, this table shows that there is considerable disagreement about the inner and outer edges of the Sun's circumstellar habitable zone:
https://en.wikipedia.org/wiki/Circumstellar_habitable_zone#Solar_System_estimates2
Furthermore, most of those estimates are for planets being habitable for some forms of life that use liquid water, not necessarily for planets habitable for lifeforms with the same requirements as humans. Lifeforms similar to humans require an atmosphere with enough oxygen for humans to breathe, for example, while some of the studies involve planets with large amounts of hydrogen in their atmospheres, incompatible with oxygen in the atmosphere.
The only estimate for the limits of the Sun's circumstellar habitable zone that I know was limited to planets habitable for humans was Dole's in 1964, the oldest, and likely to be obsolete in some parts.
With that in mind:
Some studies show that there is a possibility that life could also develop on planets that orbit a F-type star.3 It is estimated that the habitable zone of a relatively hot F0 star would extend from about 2.0 AU to 3.7 AU and between 1.1 and 2.2 AU for a relatively cool F8 star.3 However, relative to a G-type star the main problems for a hypothetical lifeform in this particular scenario would be the more intense light and the shorter stellar lifespan of the home star.3
F-type stars are known to emit much higher energy forms of light, such as UV radiation, which in the long term can have a profoundly negative effect on DNA molecules.3 Studies have shown that, for a hypothetical planet positioned at an equivalent habitable distance from an F-type star as the Earth is from the Sun (this is further away from the F-type star, inside the habitable zone), and with a similar atmosphere, life on its surface would receive about 2.5 to 7.1 times more damage from UV light compared to that on Earth.4 Thus, for its native lifeforms to survive, the hypothetical planet would need to have sufficient atmospheric shielding, such as an ozone layer in the upper atmosphere.3 Without a robust ozone layer, life could theoretically develop on the planet's surface, but it would most likely be confined to underwater or underground regions.3
https://en.wikipedia.org/wiki/F-type_main-sequence_star5
So the habitable zone of a F0 type star could extend out to 3.7 AU from the star, which is 3.7 times the distance Earth and the Sun. A orbit with 3.7 times the radius would have 3.7 times the circumference so if the planet orbited the star at the same speed that Earth orbited the Sun, the planet's year would be 3.7 Earth year's long.
But the farther away from the Sun a planet or other object is, the slower it will need to travel in order to stay in orbit. Mars orbits the Sun at a distance of 1.523 AU but has an orbital period of 1.880 earth years, Vesta orbit at at 2.361 AU but has a year 3.63 Earth years long, Ceres orbits at a distance of 2.769 AU and should have a year 2.769 Earth years long but has a year 4.61 Earth years long, Hygeria orbits at 3.141 AU but has a year 5.57 Earth years long, and so on.
Thus it seems that an object orbiting the Sun at a distance of 3.7 AU might have a year as long as 6 Earth years long.
But a spectral type F0 star which had a habitable zone extending out to 3.7 AU would be more massive than the Sun, and thus objects at 3.7 AU from that star would have to orbit the star a faster speed and thus have a year shorter than 6 Earth years.
A few exoplanets have been found orbiting in the habitable zones of their stars, and most of them have years much shorter than Earth years, as short as 4.05 Earth days in the case of TRAPPIST-1d. One, Kepler-452b, has an orbital period of 384.8 Earth days, and another, Kepler-1632b, has an orbital period of 448.3 Earth days.
https://en.wikipedia.org/wiki/List_of_potentially_habitable_exoplanets6
http://phl.upr.edu/projects/habitable-exoplanets-catalog7
So it seems that it is unlikely that a planet in the habitable zone of your fictional star could have a year anywhere near as long as the ten years you require for the day of your planet. Thus it seems like the day of your planet will be much longer than its year, and possibly many planetary years long.
Part Three, could a planet have a sidereal day longer than its year?
But a planet would form with a rotation period that would be gradually slowed by tidal interactions with its star, with any moons it might have, and with neighboring planets.
In the case of Earth, the Moon is much closer to the Sun and has stronger tidal pull, and thus has slowed the rotation of Earth and lengthened its day much more than the Sun has.
A planet in the habitable zone of a much dimmer K type or M type star would orbit much closer to the star, and thus would have much stronger tidal forces from the star, slowing its rotation much faster than the Sun slows the rotation of Earth. If the star is dim enough, and the planet orbits close enough, the star will slow the planet's rotation down so much that the planet will be tidally locked to the star, with one side always facing the star and the other side always facing away. And then it should be impossible for the star to keep on slowing the rotation of the planet, so the planet would never have a rotation period longer than its year.
As far as I can tell, the only way a planet could have a sidereal day longer than its year is if it was struck by a giant object and the impact drastically slowed the planet's rotation rate. Such an impact would be many times greater than necessary to kill all life on the planet, so for the planet to be habitable billions of year later the impact should have happened very early in the history of the planet before the first life forms arose there.
So how can your planet have a day longer than it's year?
Part Four, how a planet can have a synodic day longer than its year:
Define day.
One definition of a day is a period of light followed by a period of darkness or night.
Another definition of a day is a combined day and night, a period from sunrise to the next sunset, or noon to the next noon, or sunset to the next sunset, or midnight to the next midnight, at the same location.
And the second definition of day is the one that you mean. You desire that your planet has a period from midnight at one place to the next midnight at that place which lasts ten Earth years, or about 3,652.5 Earth Days.
But there is another question. There are several types of days, including sidereal days, synodic days, and solar days.
Sidereal time /saɪˈdɪəriəl/ is a timekeeping system that astronomers use to locate celestial objects. Using sidereal time, it is possible to easily point a telescope to the proper coordinates in the night sky. Briefly, sidereal time is a "time scale that is based on Earth's rate of rotation measured relative to the fixed stars".1
Viewed from the same location, a star seen at one position in the sky will be seen at the same position on another night at the same sidereal time. This is similar to how the time kept by a sundial can be used to find the location of the Sun. Just as the Sun and Moon appear to rise in the east and set in the west due to the rotation of Earth, so do the stars. Both solar time and sidereal time make use of the regularity of Earth's rotation about its polar axis, solar time following the Sun while sidereal time roughly follows the stars.8
A sidereal day is approximately 23 hours, 56 minutes, 4.0905 seconds (24 hours − 4 minutes + 4.0905 seconds = 86164.0905 s = 23.9344696 h). (Seconds here follow the SI definition and are not to be confused with ephemeris second.)
https://en.wikipedia.org/wiki/Sidereal_time9
A synodic day is the period it takes for a planet to rotate once in relation to the star it is orbiting (its primary body). For Earth, the synodic day is known as a solar day, and its mean length is 24 hours (with fluctuations on the order of milliseconds).
The synodic day is distinguished from the sidereal day, which is one complete rotation in relation to distant stars.1 A synodic day is from "sunrise to sunrise", whereas a sidereal day is from one rise of a given star of reference to the next. (Thus, the word day denotes the orientation relative to the main "parent" star that the observer is orbiting.) These two quantities are not equal because the revolution of the body around its parent star would cause a single "day" to pass, even if the body did not rotate itself.
https://en.wikipedia.org/wiki/Synodic_day10
So on Earth a solar day is the synodic day of Earth relative to the Sun.
Earth rotates 360 degrees of arc in one sidereal day of 23.9344696 hours.
The line between the centers of Earth and the Sun rotates 360 degrees of arc in one sidereal year, which is:
It equals 365.256 363 004 Ephemeris days for the J2000.0 epoch.1
https://en.wikipedia.org/wiki/Sidereal_year11
An ephemeris day is a period of 86,400 SI seconds.
https://en.wikipedia.org/wiki/Ephemeris_day3
Which is 24.0000 hours. So a ephemeris day is 1.0027379 sidereal days long. Thus a sidereal year should be about 366.25639 sidereal days long.
So Earth turns 360 degrees during a sidereal day, or 15.041069 degrees per hour, or 0.2506844 degrees per minute.
But during a sidereal day the planet Earth travels 360 degrees divided by 366.25639, or 0.982918 of a degree, along its orbit. So a point on Earth that was pointed directly at the Sun and was the sub solar point will not be pointed directly at the sun after one sidereal day, but will be pointed 0.982918 of a degree off the new direction to the Sun. So Earth will have to turn another 0.982918 degrees for the former sub solar point to point directly at the Sun, which should take another 3.920938 minutes, making a synodic day a little longer than a sidereal day.
So what you want is for the synodic day of your planet to last for about 10 Earth years, or about 3,652.5 Earth days. That means that the position of the star in the sky should change by 360 degrees in the planet's day or by 36 degrees per Earth year, or by about 0.0985626 degrees per Earth Day, or by about 0.0041067 degrees per Earth hour, etc., etc.
And it seems to me that if the tidal force from the star in that star system has slowed down the rotation of the planet so the sidereal day of the planet is only slightly less than the orbital period of the planet around the star, the planet's year, the synodic day of the planet can be many times as long as the sidereal day or the year of the planet.
As nearly as I can calculate, you need to make the position of the star or sun in the sky of your planet change by about 0.0985626 of a degree each Earth day in order for the position of the star or sun in the sky of the planet to change by 360 degrees, one synodic day, every ten Earth years.
Actually, looking at the question again, the target is for the synodic day to last 3,768 Earth days, so the position of the star or sun in the sky of the planet has to change by 360 degrees every 3,768 Earth days, or 0.0955414 of a degree every Earth day, or 0.0039808 of a degree every Earth hour, etc., etc.
The tidal forces of the Sun on Earth have been strong enough to slow earth's rotation noticeably over a period of 4,600,000 years, but only a tiny fraction of the amount of slowing that would be necessary for the sidereal day to be almost as long as the year, and for the synodic day to thus equal several years.
But if a planet orbited in the habitable zone of a spectral class M star, or a dimmer member of the spectral class K stars, it would have to orbit so close to the star that the star's tidal forces on the planet would slow down it rotation until it was tidally locked with one side perpetually facing the star, long before the planet was old enough to have become habitable for humans or for aliens with similar requirements.
Stephen H. Dole, in Habitable Habitable Planets for Man (1964,2007) calculated what mass a star would have to tidally lock any planet in its circumstellar habitable zone, which Dole called its "ecosphere". Dole discusses the tidal braking effects of a star upon a close planet in pages 68 to 72 of the first edition.
A "full" ecosphere can exist around primaries of stellar mass greater than about 0.88 solar mass, but the ecosphere is narrowed by the tidal braking effect for primaries of lesser mass until it disappears when the stellar mass reaches about 0.72. The range in mass of stars which could have habitable planets is thus 0.72 to 1.43 solar masses, corresponding to main-sequence stars of spectral types F2 though K1.
https://www.rand.org/content/dam/rand/pubs/commercial_books/2007/RAND_CB179-1.pdf4
So we can deduce from Dole's statement that if his calculations are correct, if a star has a mass between 0.72 and 0.88 solar masses, any planet in the inner part of its ecosphere or circumstellar habitable zone will be tidally locked by the time it is billions of years old, while a planet in the outer part of the zone will not be tidally locked.
A spectral type G8V would have a mass of 0.87 solar masses, and type G9V star would have a mass of 0.84 solar masses.
https://en.wikipedia.org/wiki/G-type_main-sequence_star12
And according to Dole a type K1V star would have a mass of 0.72 solar masses.
Tau ceti e might orbit Tau ceti, a G8V star, in the optimistic habitable zone.
Tau Ceti e is a candidate planet orbiting Tau Ceti that was detected by statistical analyses of the data of the star's variations in radial velocity that were obtained using HIRES, AAPS, and HARPS.9 Its possible properties were refined in 2017:[57] it orbits at a distance of 0.552 AU (between the orbits of Venus and Mercury in the Solar System) with an orbital period of 168 days and has a minimum mass of 3.93 Earth masses. If Tau Ceti e possesses an Earth-like atmosphere, the surface temperature would be around 68 °C (154 °F).[60] Based upon the incident flux upon the planet, a study by Güdel et al. (2014) speculated that the planet may lie outside the habitable zone and closer to a Venus-like world.[61]
https://en.wikipedia.org/wiki/Tau_Ceti#Tau_Ceti_e13
82 G Eridani, or HD 20794, is another G8V star. Planet e is supposed to orbit within its optimistic habitable zone at a distance of 0.509 AU and with a year 147.02 Earth days long.
https://en.wikipedia.org/wiki/82_G._Eridani14
Kepler-1090 is a K0V type star. Planet Kepler-1090b is supposed to orbit in its obptimistic habitable zone with a period of 198.7 days.
https://en.wikipedia.org/wiki/List_of_potentially_habitable_exoplanets6
http://exoplanet.eu/catalog/kepler-1090_b/15
Therefore, I think that you need to get someone to calculate a planetary orbit within the habitable zone of a spectral type G8V to K1V star, situated at the edge of the zone where the star will have slowed down the rotation of the star almost enough to make it tidally locked. And the difference between the planet's sidereal day and its year should be so slight that the apparent position of the star in the sky will move by only 0.0955414 of a degree every Earth day, or 0.0039808 of a degree every Earth hour, etc., etc., in order for the synodic day to be 3,768 Earth Days long.
As far as I can tell the only other possibility would be for a giant impact billions of years earlier to have slowed the planet's rotation rate. But at most for your purposes it could only have slowed the planet's rotation rate to one where its sidreal day was only a tiny little bit longer than its year, because a tiny little difference between the sidereal day and the year is what is needed to have a synodic day much longer than the year.
The direction that the natives have to travel to keep up with the sun may depend on whether the sidereal day of the planet is a little bit longer or a little bit shorter than the planet's year.