Would two moons of equal size and distance on opposite sides of a planet counteract each other's tidal pull?
$\begingroup$
$\endgroup$
8
-
$\begingroup$ What do you mean? $\endgroup$– JustSnillocCommented Apr 19, 2018 at 16:37
-
$\begingroup$ JustSnilloc i mean would they counteract eachothers effect on tides $\endgroup$– Christopher VoidCommented Apr 19, 2018 at 16:38
-
$\begingroup$ Counteract the other's pull against the planet they orbit? $\endgroup$– RonJohnCommented Apr 19, 2018 at 16:38
-
2$\begingroup$ @Mołot That would not be a stable configuration. Compare Counter-Earth. Technically that's the L3 Lagrangian point, which is unstable. (Using Wikipedia for reference because I don't really have the time to dig out better references right now.) $\endgroup$– userCommented Apr 19, 2018 at 16:41
-
4$\begingroup$ Definitely related: How would having multiple moons affect tides? $\endgroup$– userCommented Apr 19, 2018 at 16:46
|
Show 3 more comments
3 Answers
$\begingroup$
$\endgroup$
Setting apart any consideration on the long term stability of such configuration, we can look at the situation on Earth, where tides are generated by two bodies: the Moon and the Sun.
The highest tides happens when the Moon, Earth and Sun are aligned (new Moon or full Moon), while when the Moon-Earth line is perpendicular to the Sun-Earth line (first quarter and last quarter) the tides are at a minimum/
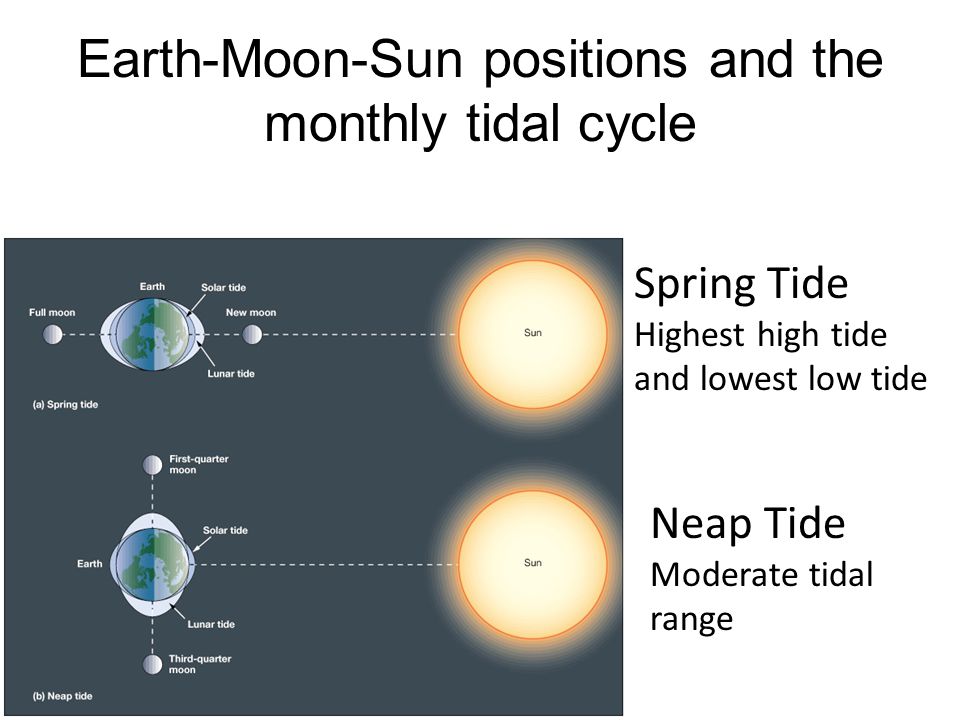
By analogy, having two moons facing each other with the planet in the middle would give higher tides than what would be with the two moons shifted by 90 degrees in the sky.
answered Apr 19, 2018 at 17:26
$\begingroup$
$\endgroup$
The 2 moons would not be in the same orbit - one would be further away from the planet than the other (unless you have something very weird going on with your planet). The further away moon would take longer to go once around the planet than the near one - the same way that Mars takes longer to go around the Sun than the Earth does.
So even if the two moons had the mass to completely balance each other's pull when they were at 90 degrees (see L.Dutch's answer), they would only do so now and then, not continuously.
$\begingroup$
$\endgroup$
2
No, they would not counteract eachother's tidal pull because the pull of gravity does not increase linearly with distance. The planet would still have two tides, as water will be attracted to each moon.
The formula for determining gravitational force between two objects includes the gravitational constant, the masses of the two objects, and the square of the distance between the two objects. Because of the formula's inverse proportionality, water on one side of the planet will feel a significantly smaller attraction to a given moon than water on the other side of the planet.
So, if Moon A was on one side of a planet and Moon B was on the opposite side, water on Moon A's side would feel a stronger force towards Moon A than Moon B, leading to a tide on Moon A's side. Similarly, Moon B's side would have its own identical tide, as water on Moon B's side would be more attracted to Moon B than to Moon A.
-
2$\begingroup$ Not only they wouldn't counteract each others tidal pull, but actually their tidal effects would add up. For the tidal effects to compensate each other the moons would have to be at 90 degrees orbital separation. $\endgroup$– AlexPCommented Apr 19, 2018 at 17:31
-
1