Is this model possible? Fast axial precession + tidal locking

Question

I'm working on a story set in a planet of permanent dusk/dawn, and with a dark side that never sees the light of sun. I know that a planet tidally locked to its star would (roughly) look like this, but I also want a day and night cycle.

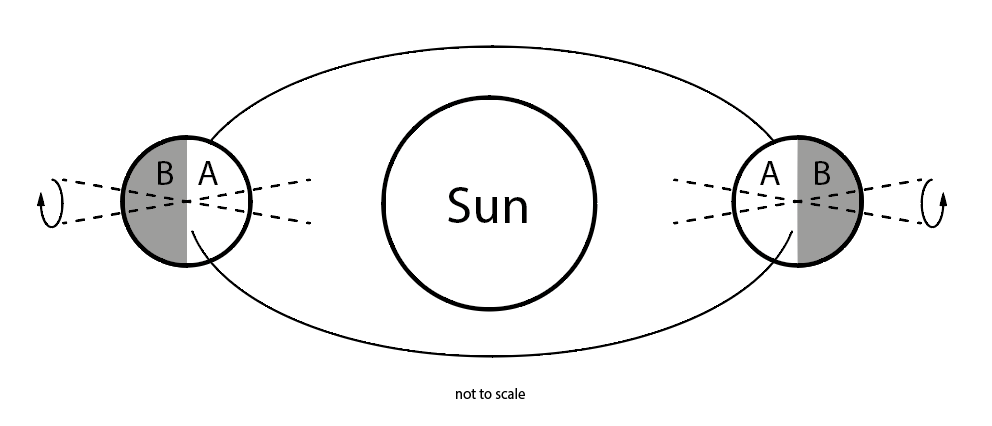
For this cycle the only idea I came up with is that this planet has a very fast precession, in cycles of about 24 hours or so. I've drawn the following model:

The requisites for my setting are:

1. The brightest the day gets is a dim light similar to dusk/dawn.
2. There must be a day/night cycle, though it doesn't have to be too pronounced.
3. There must be a dark side of the planet the sunlight never reaches (and, inevitably, a bright side).
4. Obviously, human life has to be possible.

Consider the mass, gravity and size (and other factors) of the planet similar to Earth's. The distance from the sun may vary in order to made this planet neither too hot nor too cold to sustain human life.

Is this model possible? Can a planet wobble like this (about 10 degrees) and maintain a balance?

Answer 1

The supposedly-precessing axis of your planet is pointing the wrong way. The rotational poles of a tidally-locked, synchronously-rotating world do not point towards the star. They point very nearly perpendicular to the plane of the orbit--but not necessarily exactly perpendicularly, and that is where you can get your day/night cycle from. You don't need precession--you need libration.

You will only get a perfectly stationary sun, with a perfectly stationary twilight zone, if the planet's orbit is perfectly circular, and its rotational axis is perfectly orthogonal to its orbit. Neither of those conditions are ever going to be true in nature, so you're all set!

Imperfections in the circularity of the orbit will cause the sun to appear to wobble back and forth on the east-west axis, completing one cycle per orbit. Meanwhile, inclination of the planet's axis will cause the sun to appear to wobble up-and-down on the north-south axis, also once per orbit. The two effects combined result in the sun tracing out an ellipse in the sky once per orbit, and the twilight zone wobbling around a bit accordingly. The same effect, seen from the other end, is what lets us see more than 50% of the Moon's surface from Earth over the course of a month--the Moon appears to wobble back and forth and up and down, periodically hiding and revealing regions near the edges.

So, make the planet's orbit approximately 24 hours long (perfectly reasonable if it's tidally locked and orbiting a small red star), and you can easily get bands around the twilight zone which go between night and day every 24 hours, with the sun never rising very high above the horizon.

Answer 2

Such a short period of precession would give the planet two orthogonal axes of rotation, which (as I understand) is unstable because it violates the conservation of momentum. Simultaneous movement along two axes adds to a third axis, the average of the two. There's a reason the Earth's precession is just 1° every 72 years.