I know that humans' eyes are adapted to detect some electromagnetic waves, and their ears are able to detect sound waves. What I would like to ask is: Is it plausible for a species to biologically detect gravitational waves?
My first idea is that they would probably have to evolve near a black hole rich area and not be fried by radiation.
The strain, $h$, of a gravitational wave is $$h\sim\frac{1}{R}\frac{GM}{c^2}\left(\frac{v}{c}\right)^2$$ to a relatively decent degree of accuracy. Here, $R$ is the distance to the source, $M$ is the combined mass of the black holes, and $v$ is the orbital speed of the binary. If we gratuitously assume that $v\sim0.1c$ and $M\sim50M_{\odot}$, then at one astronomical unit (AU) away, the same distance Earth is from the Sun, we find that $h\sim5\times10^{-4}$. In order to detect the waves, the species' "eyes" would need to detect a change in a meter-long object of half a millimeter - and that's with them living dangerously close to the black hole binary! Maybe extremely small lifeforms - bacteria, perhaps - would notice such a change, but macroscopic, human-sized lifeforms would not.
Now, this $h$ is greater than the strain measured by LIGO, which was on the order of $10^{-21}$, so in general, it should be easier to measure. However, it seems highly improbable that the species would involve mini laser interferometers as eyes.
It's true that the orbital speed of the black holes would increase as they begin to coalesce. Abbott et al. (2016) - the discovery paper announcing LIGO's observations that was published earlier this year - showed a maximum speed of about $0.6c$ (see Fig. 2):
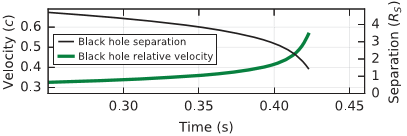
Given that $h\propto v^2$, this causes an increase in $h$ of about 36 (also, $M\sim70M_{\odot}$ for the LIGO binary), giving us a length change of 1 cm for 1-meter object, which is certainly detectable, but and it lasts for only a very short amount of time before the source stops producing gravitational waves (post-coalescence).
If we put these aliens near an area full of black hole binaries - never mind the absurdity involved in the formation of such a cluster, or the likely instabilities that could break it apart - we have the problem that different binaries could interfere with one another. Gravitational waves are . . . well, waves, and so are subject to constructive and destructive interference.
A simple metric for the expansion and contraction of spacetime by a point source of gravitational waves coming along the $z$-axis is $$ds^2=dt^2-\left[(1+2H(t,z))dx^2+(1-2H(t,z))dy^2+dz^2\right]$$ where $$H(t,z)=h\cos\left[2\pi f(t-z/v)\right]$$ for frequency $f$ and speed $v$ (which should be $c$). This should make the actual wave nature of gravitational waves clearer. If we have two sources of gravitational waves aligned along the same axis, then they can either increase the strength of the signal or significant decrease it, depending on their respective strains and the values of their $t-z/v$. More complex interactions occur when different waves are at other odd angles to one another.
I realize that I should apologize to the curious reader who may want to know more, because I looked at my notes and used non-standard notation. If you look at most papers on the subject, you'll see the quantity I referred to as $h$ denoted by $h_0$, and the quantity I referred to as $H(t,z)$ denoted by $h(t)$, where we set $z=0$. Therefore, you'd really see the equations $$h_0\sim\frac{1}{R}\frac{GM}{c^2}\left(\frac{v}{c}\right)^2,\quad h(t)=h_0\cos\left(2\pi ft\right)$$ for the case of a binary system moving together at a very slight rate. The first one might be written in terms of the orbital frequency of the system, but I prefer using this form for an easy approximation.
Is it plausible for a species to biologically detect gravitational waves?
Basically, no. The Laser Interferometer Gravitational-wave Observatory (LIGO) consists of the most high precision technology developed by the human species. Take a bow, human species! You're truly brilliant to have created such a wonderful instrument
LIGO's Extreme Engineering
LIGO exemplifies extreme engineering and technology. LIGO consists of:
Two “blind” L-shaped detectors with 4 km long vacuum chambers... built 3000 kilometers apart and operating in unison... to measure a motion 10,000 times smaller than an atomic nucleus (the smallest measurement ever attempted by science)... caused by the most violent and cataclysmic events in the Universe... occurring millions or billions of light years away!A few of LIGO's most remarkable engineering facts are listed below.
Most sensitive: LIGO is designed to detect a change in distance between its mirrors 1/10,000th the width of a proton! This is equivalent to measuring the distance to the nearest star to an accuracy smaller than the width of a human hair!
World's second-largest vacuum chambers: Encapsulating 10,000 m3 (350,000 ft3), each vacuum chamber encloses as much volume as 11 Boeing 747-400 commercial airliners. The air removed from each of LIGO’s vacuum chambers could inflate two-and-a-half MILLION footballs, or 1.8 million soccer balls! LIGO's vacuum volume is surpassed only by the Large Hadron Collider in Switzerland.
Ultra-high vacuum: The pressure inside LIGO's vacuum tubes is one-trillionth of an 'atmosphere' (in scientific terms, that’s 10-9 torr). It took 40 days (1100 hours) to remove all 10,000 m3 (353,000 ft3) of air and other residual gases from each of LIGO’s vacuum tubes to reach an air pressure one-trillionth that at sea level.
Air pressure on the vacuum tubes: 155-million kg (341-million pounds) of air press down on each 4 km length of vacuum tube. Remarkably, the steel tubes that hold all that air at bay are only 3 mm (0.12 inches) thick.
Curvature of the Earth: LIGO’s arms are so long that the curvature of the Earth is a measurable 1 meter (vertical) over the 4 km length of each arm. The most precise concrete pouring and leveling imaginable was required to counteract this curvature and ensure that LIGO’s vacuum chambers were "flat" and level. Without this work, LIGO's lasers would hit the end of each arm 1 m above the mirrors it is supposed to bounce off of!
Source: https://www.ligo.caltech.edu/page/facts
There are two main factors that mitigate against any organism developed gravitational-wave perception. Firstly, they would need to be surrounded by a continuous illumination of colliding black holes. Secondly, if they had LIGO-like receptors the things are too incredibly complicated, difficult to construct, and they're plain too big. This does overlook trying to perceive changes in size of the order of the thickness of a human hair over a length equivalent to a light year. A tad bit tricky? More like lots tricky.
Perhaps an organism that is the size of a small planet living in a cosmic environment extremely rich in colliding black holes might, remotely conceivable, need to "see" gravitational waves, but it might be able to develop other sensory systems that could do the same job with less trouble and difficulty.
Pity really. Gravitational-wave perceiving critters would be so cool!!
It's already been explained that it would be very difficult (likely impossible) for humans to develop biological receptors for gravitational waves due to sensitivity requirements. However even if such receptors existed, there does not exist a simply means to focus the gravitational waves. Remember, your eye includes a lens in addition to rods and cones.
How to Focus Gravitational Waves
As of now the only known way to focus gravitational waves would be using gravitational lensing; using the gravitational force of a massive object to bend light rays. The same is true for gravitational radiation. LIGO and other detectors do not focus the gravitational waves, but act simply as a single pixel in a camera. Directionality is then determined by triangulation using multiple detectors. So the best we can do currently is mimick ears, in the sense that we can point vaguely in the direction we detected the gravitational wave from. More detectors means better localization of the source; we know where the "light" is coming from but not what the "light source" looks like. So you would need to hand-wave a means of focusing gravitational waves (exotic matter, etc.), since the mass necessary for a gravitational lens is likely a deal breaker.
Any other way?
If you are dead-set on seeing using gravitational waves, and wish to handwave the biological gravitational wave receptors, the only way out of handwaving gravitational lenses would be gravitational wave interferometry, the gravitational equivalent to radio interferometry. ALMA's website has a good, though admittedly quite technical, description of how high-resolution images are made from arrays of telescopes (detectors).
