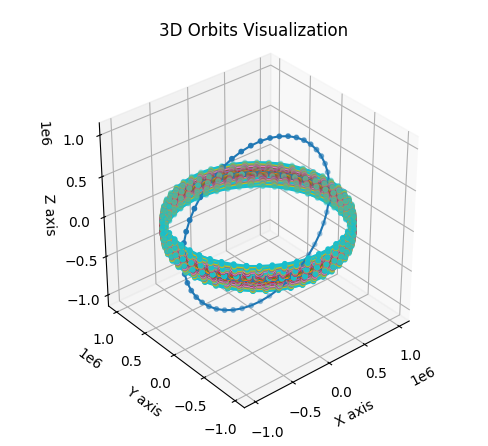
It's quite simple to set up a system with satellites in all possible positions around a star without them colliding. Inspired by the real-world 'Semicircular/hemispheric rule' system we use to manage air traffic, eastbound traffic flies at 'odd' flight levels (21 or 23 thousand feet) westbound travel at 'even' flight levels (20 or 22 thousand feet)etc.
We can use this more generally for any inclination by making each satellite's orbital altitude 10,000,000 km + its orbital inclination.
Those with an equatorial orbit will orbit at 10,000,000km, those with a polar orbit will orbit at 10,000,180 km. (You could multiply the orbital inclination by 10, or whatever you need to achieve good separation, maybe do something clever with prime numbers to even things out a little!)
This gives you something like this:
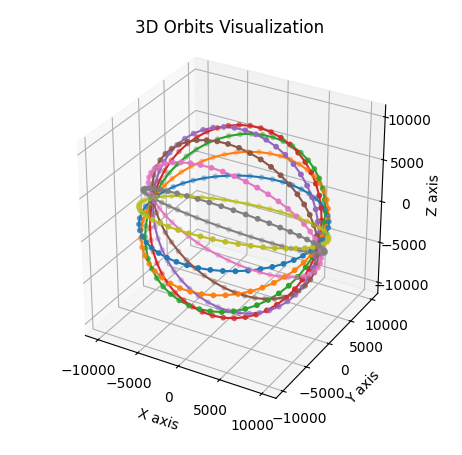
You can do the same with more orbits with an adjusted ascending node (Basically moving the 'pole' of the shell), adjust the altitude according to some factor of the ascending node too (e.g. an orbit with an ascending node of 270 degrees, and an inclination of 90 degrees, would have an altitude of 10,090,270km. you get a nice shell made up of non-intersecting orbits.
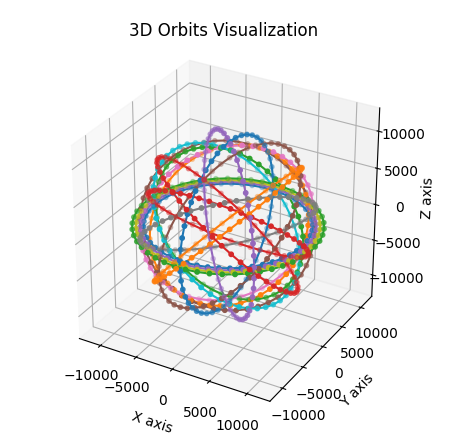
You could also do something similar with the orbital timing to reduce the amount of 'shadowing' of the outer satellites, but if we end up in a situation where the outer-most satellites are in shadow all the time, that just means that the other satellites are capturing all of the light from the sun (i.e. mission accomplished)
Also, as you asked about 'belts'. If you simply only include orbits with an inclination between some range, you'll naturally end up with something that looks like a belt.