What is the most energy efficient mode of travel by a land animal on a terrestrial planet with 0.1g at surface?

Question

Imagine there is a planet similar to Earth but has a much lower density, the gravitational acceleration at mean sea level is approximately a tenth of Earth's. I am wondering how would a land animal weighting as much as a hare move quickly on every type of terrain, ranging from snow to open grassland. Air time should not be longer than 10% of the time when making the journey.

Answer 1

Although low-gravity makes it much easier to travel long distances while airborne (as other users have noticed in their answers and comments), it might actually make it more difficult to move quickly, i.e. achieve a high velocity by accelerating quickly. This is because most land animals require contact with the ground in order to accelerate, and low gravity means that animals will remain airborne longer in between “footsteps.”

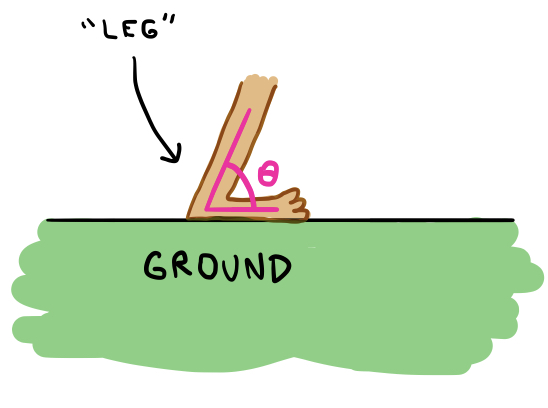
Suppose we have an animal that accelerates by running similarly to humans, rabbits, etc., by pushing against the ground with a leg-like appendage at an angle $\theta$.

Suppose this animal has mass $m$ and is capable of applying a force $F$ with its leg and maintaining contact with the ground for $t_c$ seconds. This force $F$ can be separated into horizontal and vertical components.

A force with magnitude $F\sin\theta$ is directed vertically and perpendicular to the ground, meaning that the normal force from the ground will propel the animal into the air with a vertical velocity of $Ft_c\sin\theta/m$. Assuming the ground is approximately flat in the direction of travel, the time elapsed before the animal lands on the ground again is equal to

$$t_{\text{land}} = \sqrt{\frac{2Ft_c\sin\theta}{mg}}$$

Additionally, a force with magnitude $F\cos\theta$ is directed parallel to the ground. Assuming that friction is great enough for no slippage to occur (which admittedly may not be the case when, say, there is snow on the ground), the horizontal acceleration is $F\cos\theta/m$ and the increase in horizontal velocity is $Ft_c\cos\theta/m$.

Assuming $t_c$ remains constant regardless of the animal’s velocity and $t_c << t_{\text{land}}$, we have that the animal is capable of increasing its horizontal velocity by $Ft_c\cos\theta/m$ every $t_{\text{land}}$ seconds. This makes for an effective acceleration of

$$a_{\text{eff}}=\frac{Ft_c\cos\theta}{mt_{\text{land}}}=\cos\theta\sqrt{\frac{2Fgt_c}{m\sin\theta}}$$

What does this tell us about animals on your planet?

• Since $a_{\text{eff}}$ is proportional to $g^{1/2}$, and your planet has a gravity $1/10$ that of Earth’s, you should expect analogous animals on this planet to accelerate $1/\sqrt{10}\approx 0.316$ times as fast.
• Sorry, but airtime will almost certainly make up (much) more than $10\%$ of the time. If you want to fix this, I recommend designing an animal with a very small value of $\theta$. This causes the majority of propulsion force to be horizontal, but the animal will probably need some sort of “friction pads” on its feet to prevent slippage in this case.
  • $\theta$ really does make a big difference. For small values of $\theta$, halving the value of $\theta$ increase $a_{\text{eff}}$ approximately by a factor of $\sqrt{2}\approx 1.414$.
• I didn’t do the calculations here, but the coefficient of friction and the possibility of “slippage” could make a big difference. I’d expect animals in low-friction environments (e.g. wet and snowy ones) to use much different transportation methods than those in high-friction environments (e.g. grassy and rocky ones).

That being said, all animals on this planet will have the same difficulty with accelerating. Evolutionarily speaking, since predators will also move more slowly, there’s no reason for animals to evolve the ability to move anywhere near Earth-level acceleration speeds.

Answer 2

hmm, actually, a rocky planet as done by a study, indicates that a rocky planet to be habitatable must be at a minimum, 0.02 Earth masses, which is an object bigger than the moon. so there's no way a rocky planet with the density you speak of could exist that would be habitable, and the materials that would be less dense that the minerals here on Earth would be water.

it'd be a Micro-Neptune

Answer 3

Probably something like a kangaroo where the energy of a hop can be absorbed on landing ready for the next hop. It might not even need much of a tail for stability under such low gravity conditions. Just lean forward then hop on a ballistic trajectory, swivel the body whilst in flight so the legs are facing in the direction of motion and are ready to absorb the energy on landing. To change direction put extra force into one leg or the other.

Answer 4

Forget about how animals could run on that planet, how could they survive on the planet?

And how could any hypothetical Earth Humans in that story survive on the surface of the planet without pressure domes and space suits?

There are many discussions of the requirements for planetary habitability, but those are usually about the suitability of a world to be habitable for carbon based lifeforms using liquid water, Earth life in the most general sense. Humans, and other large land animals, can survive in a more restricted range of environments than Earth life in general.

There is one source that I am familiar with, describing and discussing the requirements for a planet to be habitable for humans and other large multi celled land animals from Earth with similar requirements.

*Habitable Planets for Man (1964,2007), by Stephen H. Dole. You might be able to access or download it at:

https://www.rand.org/pubs/commercial_books/CB179-1.html[1]

In chapter Four The Astronomical Parameters the section on planetary properties on pages 53 to 67 discusses the property of the planet necessary for human habitability.

Dole says that planet needs to have a surface gravity of less than 1.5 g to be habitable, which according to figure 9 on page 31 corresponds to a planet with a mass of 2.35 Earth, a radius of 1.25 Earth, and an escape velocity of 15.3 kilometers per second. (page 53).

I note that you specify the surface gravity of your planet, but not its escape velocity. The ability of a planet to retain whatever atmosphere it acquires depends of the chemical composition of that atmosphere, the escape velocity at the outer edges of the atmosphere where gases escape, and on the average velocity of the air particles in the escape lawyers of the atmosphere.

Dole says that in order for a planet to retain atmospheric oxygen, its escape velocity should be:

"of the order of five times the root-mean-square velocity of the oxygen atoms in the exosphere".

(page 54)

Dole calculates that the escape velocity of the smallest planet capable of retaining atmospheric oxygen can be as low as 6.25 kilometers per second. According to figure 9 that corresponds to a planet:

"having a mass of 0.125 Earth mass, a radius of 0.63 Earth radius, and a surface gravity of 0.49 g. Under the above assumptions, such a planet could theoretically hold an oxygen-rich atmosphere, but would probably be much too small to produce one, as will be seen below."

(page 54)

I note that a surface gravity of 0.49 g is 4.9 times as much as the 0.1 g you specified.

Dole then makes two separate rough calculations of the minimum sized planet necessary to produce an oxygen-rich atmosphere.

Dole calculates 0.25 Earth mass in one calculation, which he considers too low, and in the other calculation 0.0.57 Earth mass, which he considers too high.

"With 0.25 being too low and 0.57 being too high, the appropriate value of mass for the smallest habitable planet must lie between these figures, somewhere in the vicinity of 0.4 Earth mass."

(page 56).

"Since it is not possible to obtain a more precise determination of the minimum mass of a habitable planet, for our purposes the value of 0.4 Earth mass will be adopted as the minimum mass. This corresponds to a planet having a radius of 0.78 Earth Radius and a surface gravity of 0.68 g."

(page 57).

I note that a surface gravity of 0.68 g is 6.8 times the 0.1 g you specify.

I note that since Dole wrote, there have been numerous discoveries in planetary science, some of which might change some of his conclusions.

For example "Exomoon Habitability Constrained by Illumination and Tidal Heating", Rene Heller and Rory Barnes, part 2, Habitability of Exomoons, suggests that the upper mass limit for habitable planets and moons might be different from that of Dole.

They suggest a minimum mass of 0.25 Earth for a moon to be habitable (for life in general, not necessarily for humans) and:

An upper mass limit is given by the fact that increasing mass leads to high pressures in the moon’s interior, which will increase the mantle viscosity and depress heat transfer throughout the mantle as well as in the core. Above a critical mass, the dynamo is strongly suppressed and becomes too weak to generate a magnetic field or sustain plate tectonics. This maximum mass can be placed around 2M⊕ (Gaidos et al. 2010; Noack & Breuer 2011; Stamenković et al. 2011). Summing up these conditions, we expect approximately Earth-mass moons to be habitable, and these objects could be detectable with the newly started Hunt for Exomoons with Kepler (HEK) project (Kipping et al. 2012).

https://arxiv.org/ftp/arxiv/papers/1209/1209.5323.pdf[2]

Another study suggests that Earth might be almost the smallest possible habitable planet:

0.3 Earth masses has been offered as a rough dividing line for habitable planets.[48] However, a 2008 study by the Harvard-Smithsonian Center for Astrophysics suggests that the dividing line may be higher. Earth may in fact lie on the lower boundary of habitability: if it were any smaller, plate tectonics would be impossible. Venus, which has 85% of Earth's mass, shows no signs of tectonic activity. Conversely, "super-Earths", terrestrial planets with higher masses than Earth, would have higher levels of plate tectonics and thus be firmly placed in the habitable range.[49]

https://en.wikipedia.org/wiki/Planetary_habitability#Mass[3]

If the belief that plate tectonics are necessary for habitability is correct, and if the lack of plate tectonics on Venus is due to its mass, and not to some other factor, then the lower mass limit for a habitable planet would be somewhere between the mass of Venus, 0.815 Earth, and the mass of Earth, 1.0 Earth.

Venus, with 0.815 Earth's mass, has a surface gravity of 0.904 g, which is 9.04 times your 0.1 g. Earth, with 1.000 Earth's mass, has a surface gravity of 1 g, which is 10.00 times your 0.1 g.

However, there is also some evidence that the minimum mass of a habitable planet could be much less than Dole calculated.

Ganymede and Callisto, the largest moons of Jupiter, have masses of 0.025 Earth and 0.018 Earth, and escape velocities of 2.741 and 2.440 kilometers per second, 0.245 and 0.218 Earth's escape velocity of 11.186 kilometers per second, and surface gravity of 0.146 g and 0.126 g.

Titan, the largest moon of Saturn, has similar properties, having a mass of 0.225 Earth, an escape velocity of 2.639 kilometers per second, 0.2359 that of Earth, and a surface gravity of 0.138 g.

Note that the escape velocities of all three moons, necessary to retain any hypothetical atmospheres they might have, are higher proportional to Earth's escape velocity than their surface gravity is proportional to Earth's surface gravity. This indicates that it might theoretically be possible for some planet or moon to have an escape velocity high enough to retain a dense atmosphere and also a surface gravity of only 0.1 g as you specified.

Incidentally, what sort of atmospheres do Ganymede, Callisto, and Titan have? Ganymede and Callisto have extremely thin atmospheric densities, vacuums for all practical purposes, so it would be expected that Titan would be the same.

But the atmospheric surface pressure on Titan is given as 146.7 kPa, or 1.45 atmospheres. That is 1.45 times the surface pressure on Earth.

Since Titan's ability to retain an atmosphere is similar to that of Ganymede and Callisto, the fact that Titan has so many millions of times more atmosphere than they do must be due to having produced or acquired much more atmosphere than they did, instead of having some superior ability to retain an atmosphere.

So the world in your story should be some small planet, dwarf planet, or moon of a planet, and have a dense rocky core surrounded by hundreds or thousands of kilometers or miles of ice or water to reduce the world's density and to give a a very low surface gravity while still having a high enough escape velocity to retain a dense enough and oxygen rich atmosphere.

But how can land animals run on the surface of land if the only land on the world is far below the surface of the ocean that covers the whole world?

If the world is an exomoon of a giant exoplanet, tidal heating might produce intense volcanism on the exommon. And the magma produced by the volcanoes in the rocky core should be cooled into rock by the ocean waters. And on Earth many forms of volcanic rock and ash are lighter than water. So much of the volcanic rock would float to the surface of the world ocean. And if enough volcanism produces enough rock to float to the surface of the ocean, it may produce floating islands and continents of volcanic rock that might last long enough for land based multi celled plants and animals to evolve.

So possibly you might be able to design such a world, one which somehow produces and retain a dense and oxygen rich atmosphere, and which has a surface gravity of only 0.2 g, 0.15 g, or even only 0.1 g.

And possibly there might have to be some sort of mini black hole in the center of the planet to make the surface gravity and escape velocity calculations come out correctly.

And possibly that world might have been modified and terraformed in the past by highly advanced aliens.

Or possibly that world might have been constructed in the past by highly advanced aliens. It might be a hollow cylinder which rotates to produce a simulated gravity of 0.1 g in the inner surface of the cylinder, and it might use its walls, instead of its escape velocity, to retain its atmosphere.

And the answers to this question might be useful:

https://worldbuilding.stackexchange.com/questions/178892/can-the-little-princes-planet-actually-exist-in-our-universe[4]

Answer 5

Efficient running requires two things:

1. Maximizing the amount of energy that goes into propelling you forwards vs. upwards.
2. Maximizing the conservation of energy between strides.

Wheels are ideal for both purposes. The friction of a wheel against the ground provides 100% forward force, and zero normal force, and rolling maximizes the bang for your energy buck. Unfortunately, wheels are not suitable for all types of terrain, which is why very few Earthling creatures make use of rolling locomotion.

So, how do we maximize the use of legs? Well, to start with, you'll want a bunch of them. At least six, so that you can keep a stable base at all times while moving half the legs at a time. That gives you more surface area for traction, and ensures that the body can maintain a constant stable altitude throughout the stride, so no energy is wasted on the body bobbing up and down. More legs gives you better acceleration, because better traction, so particularly fast ground creatures are likely to be centipede-like (and centipedes on Earth are, in fact, quite fast!), with a wave gait. Faster speeds will involve more simultaneous motion waves.

At some point, however, the need to continuously move the limbs up and down (absolutely) and back and forth (relative to the body) ends up sucking up a bunch of energy, and traction becomes less important when you are already up to speed and don't require high accelerations. The design of the limbs to include energy-recovery structures (like elastic tendons) can help, but eventually the ideal strategy begins to be ignoring some of the legs, so as to conserve more energy across subsequent strides. Thus, the fastest centipedomorphs will probably borrow a strategy from running lizards, lift their front body off the ground, and continue running with ever-smaller numbers of hind limbs at higher and higher speeds.

Multiple limbs is not, however, the only way to get high acceleration--a large foot area per limb can manage it as well. Thus, you could also see quadrupedal or bipedal animals with a mixed plantigrade / digitigrade gait--plantigrade to maximize traction at low speeds, with limbs carefully designed to ensure that they can move backwards at a constant extension when planted and containing energy-recovery structures to gain losses incurred when planting a foot upon lifting it at the back end of a stride, transitioning to digitigrade running at higher speeds.

Answer 6

Try to run? It'll take forever to accelerate, and you'd slip all over the ground! My idea is that the organism takes advantage of the friction (or lack thereof), by having a smooth underbelly and legs on either side that can be used to accelerate, brake or turn! And it lifts its legs up and doesn't use them unless it needs to increase its speed, slow down, stop or turn.