Could a flat planet form by rotation?

Question

Suppose a planet had an extremely fast rotation, so fast that the planet is flattened considerably and somewhat resembles fictional flat earth, Only at the north pile does life to exist, where the gravity is strong enough. This planet is tilted dramatically, like Uranus, so that this pole faces the sun almost directly during the summer. The planet orbits the sun very quickly, so that a year goes by in 24 hours, thus creating a “day/night” cycle despite the rotation being extremely fast.

How plausible is this?

Answer 1

A flat(ish) planet may be possible, but not quite as you've set it up

Haumea is a dwarf planet in our own solar system that is significantly flattened out by its own rotation. So, we know that stretched out, quickly rotating worlds can exist.

However, the gravity part of your idea does not work. No matter where you stand, gravity will always feel about the same. Planets are by definition hydrostatic; so, no matter how you change the properties of what a planet is made out of, its shape will always settle to rest in a form where the surface experiences nearly uniform gravity. The more massive the planet, the more true this is.

At your poles you may experience a bit more gravity because the solids in your world are not truly fluid so they would resist flattening to a degree, but if you were to stand on the North Pole, there would be significant amounts of gravity pulling you left and right in equilibrium, but much less pulling you down. As you walk towards the edge of the planet, the angle of gravity changes and becomes stronger... however, the strength of the planets centrifugal force also becomes stronger in proportion to the increase in gravity, because it is this equilibrium that defines the planet's flattened shape.

So while you may think that as you approach the "edge of the world", that you would feel like you are going up hill, this feeling would be negligible. In fact, this planet could possibly have a very large habitable zone and stable atmosphere everywhere you go. The Coriolis Effect may cause severe winds that might make it unsuitable for Earth Based life though.

Also, a 1 day Orbit around its parent star may lead to tidal locking making the fast rate of rotation difficult to explain. While its fast rate of rotation will resist tidal locking at first, it will also bleed off energy quickly (in geological time scales) in order to offer this resistance slowing the spin down.

Answer 2

You can't get there from here with real physics.

The amount of oblateness is related to the amount of spin. If the centrifugal force is anywhere near the gravitational pull, then the atmosphere will all drain off of the edges.

This answer provides good calculations on how oblate your planet can get before it rips itself in half. Spoiler: it's roughly a 3:1 ratio.

(retracted, please see below)

On the rotation/orbit thing, I don't believe that you can have a 24 hour orbit at a distance that also corresponds to the goldilocks zone for habitable life. I'd have to do a bit of research to figure out if a planet could precess that fast without ripping itself apart, but I think that would significantly reduce the speed of spin.

So, sorry, in an environment where Niven couldn't get away with God's Easteregg, you couldn't get away with this. Maybe you can crank your suspension of disbelief up a couple notches.

Addendum: After a little research, I found the possibility of an actual moon-sized object orbiting a white dwarf roughly every 24 hours, within the habitable zone of that star. As such, your crazy-fast orbit is actually MORE likely than the pancake shaped planet.

Answer 3

From what I recall, Hal Clement's calculations for Mesklin were found to be wrong -- even with the mass of several Jupiters to work with, you couldn't actually have a planet with such a high rate of spin.

First, the accepted explanation (as far as I'm aware) for a planet's rotation rate is that it's due to the impacts of accreting bodies during planetary formation -- hence Venus wound up with a sidereal day longer than its year, Earth and Mars came out almost the same, all the gas giants rotate faster (fast enough, in the case of Jupiter, to be visibly oblate through a very modest telescope).

If you have a planet with high enough spin rate to be flattened to the level you want, it would have to have been struck many times on the same (receding) edge and this would have to have occurred after the one that upset the spin axis to be orthogonal to the spin of the rest of its system. And as the spin builds up beyond the (roughly) half kilometer per second Earth has, each subsequent impact adds less to the rotation rate than the last.

Then we run into the gravitational and mechanical issues that proved Mesklin was impossible. In short, Mesklin (or any other planet with a similar ratio of effective surface gravity from poles to equator) will lose its atmosphere, likely before life could evolve to make the atmosphere breathable. In your case, you'd have surface gravity of, say, 3G at the poles (same as Mesklin at the rim), but nearly nothing at the very fast-moving equator. Orbital velocity isn't enough above the equatorial rotation speed, and the atmosphere (far taller in the lower gravity) will reach up into orbit -- at which point it simply floats away.

You would not feel like you're walking uphill going to the edge -- the surface (of both land and sea) would feel level (because it rests at gravitational equipotential). It's just that you'd get to a point, if the spin rate is high enough, where you could jump high enough to suffocate...

Answer 4

It Needs to Rotate once Every Few Seconds.

The formula you want is:

$$\frac{a-b}{b} = \frac{5}{4}\frac{(2\pi/T)^2 R^3}{GM}$$

Where:

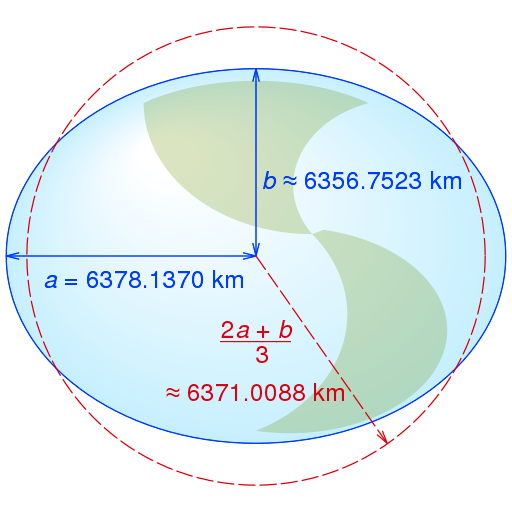
$a$ is the (larger) equatorial radius.

$b$ is the (smaller) polar radius.

$T$ is the time for one revolution.

$R = (b+2a)/3$ is the mean radius.

$M$ is the mass of the planet.

$G$ is Newton's constant.

The formula is derived in this thread where it seems to agree with the experimental numbers for Earth. This is good for you because it suggests we can indeed ignore how the planet is denser in the middle. See also Wikipedia and their source [8].

---

Since you want $a$ much bigger than $b$ we'll just use $R \simeq 2a/3$.

Since $a/b$ is very large we'll also write $\frac{a-b}{b} = \frac{a }{b}-1 \simeq \frac{a }{b} $. The formula becomes

$$\frac{a }{b} = \frac{5}{4}\frac{(2\pi/T)^2 }{GM}\frac{8 a^3}{27}$$

I'm going to ignore the constant factors because the goal is to show the thing has to spin ludicrously fast.

$$\frac{a }{b} = \frac{a^3 }{GM T^2} $$

Let's take $a$ as the 6000km radius of the Earth and $M$ the Earth's mass. In Si units:

$G \simeq 6 \times 10^{-11}\simeq 10^{-11}$

$a \simeq 6000km = 6000 \times 1000 = 6 \times 10^3 \times 10^3 = 6 \times 10^6 \simeq 10^6$

$M \simeq 6 \times 10^{24} \simeq 10^{24}$.

So we get:

$$\frac{a }{b} = \frac{10^{18} }{10^{-11} 10^{24} T^2}= \frac{10^{18} }{ 10^{13} T^2} = \frac{10^{5} }{ T^2}$$

If for example you want the ratio to be 1/100 we need

$$100 = \frac{10^{5} }{ T^2} \implies T^2 = 10^3 \implies T = 10^{1.5} \simeq 32 $$

Meaning one revolution every 32 seconds.

How fast is that?

The equator of the pancake is 40,000 km long. So an ant standing on the rim moves more than 1000km each second. Much zippier than the ISS orbit speed of 10ish km/s but only a measly 0.3% the speed of light.