Planetary cave: Gravity inside a non-concentric shell

Question

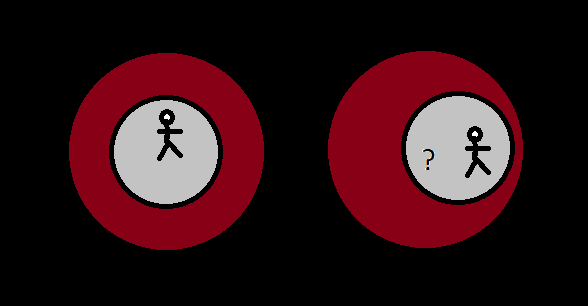
There are several previous questions concerning concentric shells. I won't reference them here because this is different.

I understand that there is no gravitational effect inside a concentric shell*. But what about a non-concentric one?

Research

I've looked online and found nothing. Maybe I'm just using the wrong search terms?

Question

I'd like to have a cavity inside a small planet. Assuming perfect spheres and uniform density, is there a general equation for the gravitational field inside the cavity, taking into account:

• The radius of the solid sphere
• The radius of the hollow spherical cavity
• The offset between centres.

Supplementary

If no exact solution exists, is there an approximate formula that will let me play with the variables to get a rough idea of the effects?

---

*Shell Theorem

In classical mechanics, the shell theorem gives gravitational simplifications that can be applied to objects inside or outside a spherically symmetrical body. This theorem has particular application to astronomy ... A spherically symmetric body affects external objects gravitationally as though all of its mass were concentrated at a point at its centre. If the body is a spherically symmetric shell (i.e., a hollow ball), no net gravitational force is exerted by the shell on any object inside, regardless of the object's location within the shell.

Answer 1

The solution isn't actually too bad; I had this as a problem in AP physics in high school. It doesn't have as much symmetry to exploit as a concentric shell, but it still has a lot of symmetry--as long as the cave and the enclosing body are both spheres, and the enclosing body has uniform density, you can treat everything as rotationally symmetric around the line connecting their centers.

From there, you can treat the cave as a body of negative mass whose gravity is added to that of the enclosing body. The somewhat surprising end result is that gravity is constant inside a spherical cave, and antiparallel to the offset of the cave center from the enclosing body's center.

Because of the rotational symmetry, we can reduce the problem to two dimensions to show that the field is in fact constant everywhere in the cave.

Let the enclosing body have radius $R$ and density $\rho$, the offset between centers be $d < R$, and the cave have radius $r < R - d$. The gravitational field inside a body of uniform density is $g \propto \rho x$. Expanded to 2 dimensions, the total gravitational force is $g \propto \rho \sqrt{x^2+y^2}$, but broken down into vector components we get $g_x \propto \rho \sqrt{x^2+y^2}\cos\theta = \rho \sqrt{x^2+y^2} \frac{x}{\sqrt{x^2+y^2}} = \rho x$, and similarly $g_y \propto \rho y$. The gravity due to the negative body that creates the cave when added to the enclosing body is $g_x \propto -\rho (x-d)$ and $g_y \propto -\rho y$. Adding these together, we get the summed components for the net gravity vector to be $g_x \propto \rho x - \rho (x - d) = \rho (x - (x - d)) = \rho d$ (i.e., a non-zero constant), and $g_y \propto \rho y - \rho y = 0$.

So, the total gravity is axis-aligned, constant, and dependent only on the density of the enclosing sphere and the eccentric distance. This is in fact the only way I know of to get an exact constant, uniform gravitational field with finite amounts of material (the infinite option being the space above an infinite plane).

To get the actual force (not just a factor it is proportional to), add a factor of $\frac{4 \pi G}{3}$ to get $g = \frac{4 \pi G}{3}\rho d$.

Answer 2

This is a classic problem in electrostatics - that is, in an analogous situation where we care about calculating the electric force on an object inside some cavity. The same solution technique applies for Newtonian gravity, and it relies on something called superposition. Effectively, the cavity is like a region of space inside a sphere of mass density $\rho$ centered at a point $\mathbf{p}$, inside which you've placed a smaller sphere of mass density $-\rho$ centered at a point $\mathbf{p}'$. In the region of intersection, the two densities cancel out, leaving you with a net density of $0$.

A simpler case

Say we have a body with uniform density $\rho$. We can use something called Gauss's law for gravity. This shouldn't be confused with its cousin, Gauss's law for electrostatics - usually just called "Gauss's law" - or the underlying mathematical theorem behind them both, referred to as the divergence theorem or Gauss's theorem. Regardless of how you want to refer to it, the law goes like this: $$\int_{\mathcal{S}}\mathbf{g}\cdot\mathrm{d}\mathbf{A}=-4\pi G\int_{\mathcal{V}}\rho\mathrm{d}V$$ where $\mathcal{V}$ is a surface with boundary $\mathcal{S}$, $\mathbf{g}$ is the gravitational field and $d\mathbf{A}$ is an area element. Then, in our case of a uniform sphere, $$g(r)\cdot4\pi r^2=-4\pi G\rho\frac{4\pi}{3}r^3$$ and $$g(r)=-\frac{4\pi G\rho}{3}r$$ We know that $\mathrm{d}\mathbf{A}$ points radially outwards, so does $\mathbf{g}$ by spherical symmetry, and so $$\mathbf{g}(\mathbf{r})=-\frac{4\pi G\rho}{3}\mathbf{r}$$ as claimed.

Modeling the cave

The principle of superposition says that to calculate the gravitational field due to two objects, we can simply add the gravitational fields created by each object. Let's call these fields $\mathbf{g}_+$ and $\mathbf{g}_-$, coming from the sphere of density $\rho$ and the sphere of density $-\rho$, respectively. Now we just apply the result from the last section: $$\mathbf{g}_+(\mathbf{r})=-\frac{4\pi G\rho}{3}(\mathbf{r}-\mathbf{p}),\quad\mathbf{g}_-(\mathbf{r})=\frac{4\pi G\rho}{3}(\mathbf{r}-\mathbf{p}')$$ The total gravitational fields is then $$\mathbf{g}(\mathbf{r})=\mathbf{g}_+(\mathbf{r})+\mathbf{g}_-(\mathbf{r})=-\frac{4\pi G\rho}{3}(\mathbf{p}-\mathbf{p}')$$ which is constant, though non-zero. Notice that if the spheres are concentric, $\mathbf{p}-\mathbf{p}'=\mathbf{0}$ and the field vanishes - the same result as the good old shell theorem.