It will be too hot there for liquid water.
Let us science this up. How can we determine how close this planet is to its star? We know the size of the star and we know how big it appears from the perspective of the planet. We can use those values to calculate the distance. This will be a big help in determining likely climate.
https://lco.global/spacebook/sky/using-angles-describe-positions-and-apparent-sizes-objects/
d = 206,265 D / θ
D = linear size of an object θ = angular size of the object, in arcsec d = distance to the object
Star is 74% the width of our sun. Our sun is 1392000km, **0.74 = 1030080 km width of star. From OP angular size is 1.88 degrees Convert degrees into arc seconds = 1.88 * 3600 = 6768
1030080 * 206265 = 212469451200 212469451200/ 6768 = the planet is 31393240 km away from its star; round to 31 million km = 0.2 AU
Our sun by comparison is 150000000 km away; 150 million km Our sun is 70 million km from Mercury.
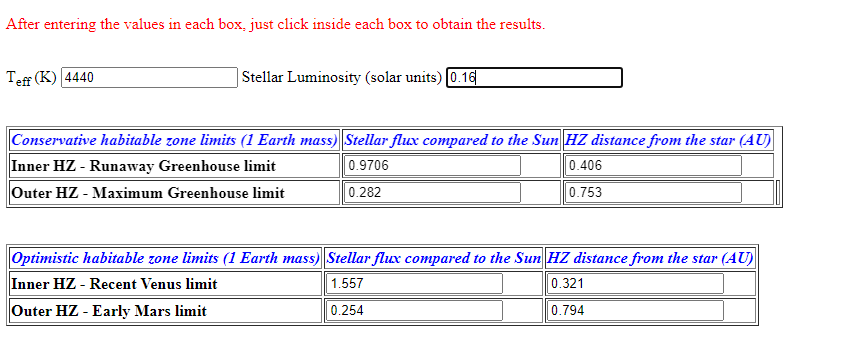
To use the habitable zone calculator one needs luminosity and temperature. OP provides luminosity of 16% of our sun. I took temperature from here
https://en.wikipedia.org/wiki/K-type_main-sequence_star ; an average K5 star has 0.17 luminosity so that matches, and wikipedia gives an average of 4440K temperature
Here is a snip from the calculator at http://depts.washington.edu/naivpl/sites/default/files/hz_0.shtml#overlay-context=content/hz-calculator
At 0.2 AU the planet is not inside the habitable zone of its star. It is too close to the star. It is hot there. The answer to the OP as regards the climate: toasty, as in stuff there is toast. Melba toast.
A side question should someone be interested in playing with these calculators is that of the accretion disc. I did not factor that in but it too will illuminate this planet. One quintillion times as bright as the sun is pretty bright. 1800 arc seconds in the sky is not trivial. How far away is the radiation source represented by the disc and what is the calculated habitable zone, treating this object as a star for purposes of calculation. Dailey I am trying to salvage your world and I suspect that maybe it could be a rogue planet, warmed just by the disc.
Anyone interested in crunching those numbers is welcome to addend an edit to this question. I think the temperature of the disc might be hard to estimate.