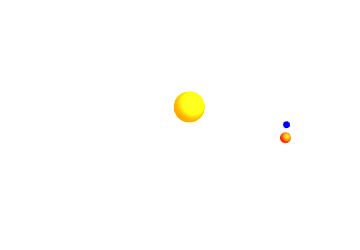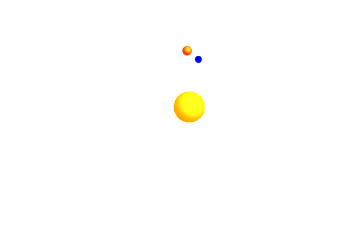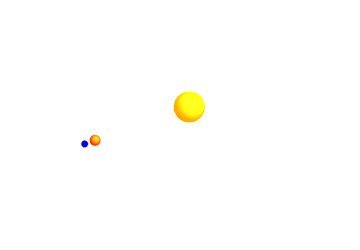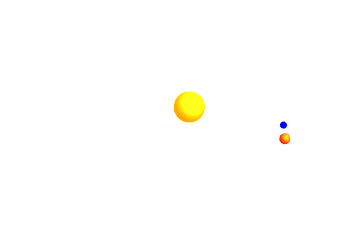A possibility yet not explored(also at the end of LSami's answer) might be a binary system with a suitable 3D configuration of the orbits.
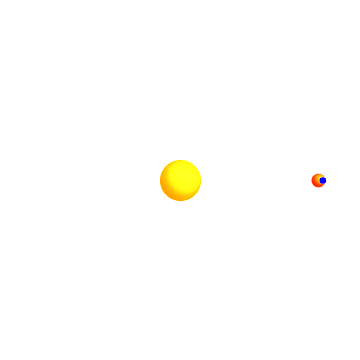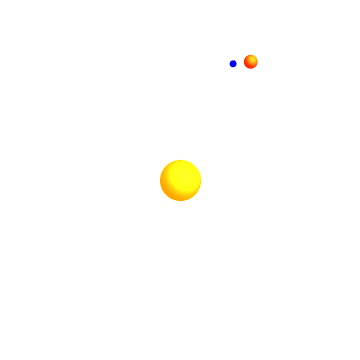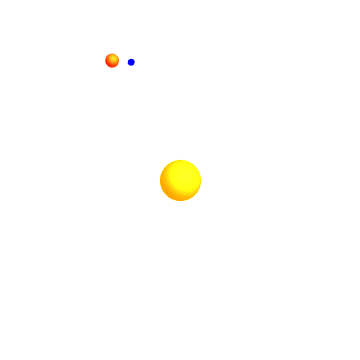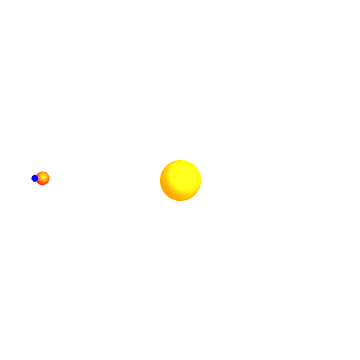
Imagine a 3D coordinate system with the $x$-axis pointing right, the $y$-axis pointing up, and the $z$-axis pointing at the viewer. We have a central yellow star (sitting at the origin in the animation, but it really should orbit the center of mass also), a red dwarf slowly orbiting the bigger component of the binary system in the $xy$-plane. And, finally, a planet (the blue dot), orbiting about the red dwarf in a plane parallel to $xz$-plane (i.e. one that has the $y$-axis as its normal). The animation tries to give a top-down view of the motion
The points:
- Unless the smaller star is close to the $x$-axis, it will not eclipse the bigger star, because the bigger star is not on the plane of the orbit of the planet.
- When the smaller star is very close to the $x$-axis, it may eclipse the bigger star, but if we synchronize the periods we can arrange the planet to always be either above or below the $xy$-plane at those instants, when the small star crosses the $x$-axis.
- When the dwarf is near the $y$-axis, 3/4 of the planet bathes in starlight. The ratio goes close to 1/2 in those years, where the bigger star is closer to the plane of the planet's orbit, and even then only for a single season (one season the planet will be nearly between the stars and be fully lit).
The caveats:
- I suck at celestial mechanics, but I suspect the long term stability of this set up may be in doubt. At least the ratio of orbital periods likely needs to be quite high, may be something like one hundred (if not thousands) of "planet years" per a single orbit of the red dwarf about the bigger star.
- Also, gravity of the bigger star may make the plane of the planet's orbit rotate over time.
- Also, if the ratio of periods is 1000:1, then the above synchronization idea doesn't help very much. The planet will reach the $xy$-plane, at points when the red dwarf has moved only very little off the $x$-axis. At those points the dwarf may almost eclipse the bigges star, resulting in something like only $50.001$ per cent of the panet having a semblance of a day. (in the animation the ratio of those periods is 10:1)
- But, those close to 50-50 days are few and far between. It might make for an occasion for the culture living on the planet!