You're talking about a double planet (and may want to look at this question).
The two planets have masses of 7M (twice the size of the Earth is about eight times the volume, but I'm allowing for more light elements to have been captured) and 0.15M respectively, so the ratio imbalance is about twice that of the Earth-Moon system; using Asimov's satellite criterion, ours is a double planet system.
If it orbited at the same distance of the Moon, Alpha being 7X as massive as Earth, Beta would complete one revolution in about $\sqrt{7}$ = 2.64 less time, giving 10.6 Earth days. To have it orbit every day, it must be $10.6^\frac{2}{3}$ = 4.827 times nearer, which means about 78720 km between the centers (62600 km between the surfaces), in geosynchronous orbit.
(The Roche critical distance for Alpha is $d = 1.442 R_m$ or about 6000 km above the surface; so, while it would experience some remarkable tides, Beta would not disintegrate into a ring).
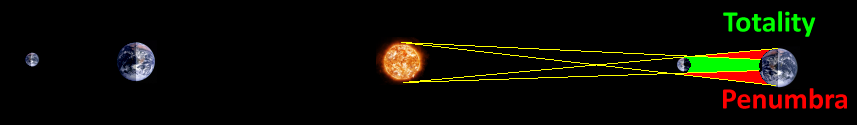
Supposing Alpha rotates once every 24 hours, has no axial tilt, and Beta orbits on the plane of the ecliptic, we are in a tidal lock situation - Alpha always shows the same face to Beta and passes directly between Alpha and its sun. Which means that during daytime, Beta eclipses Alpha, and half of Alpha is always dusk (either because it's a night illuminated by a full Beta, or because it's day and Beta causes a solar eclipse), while the other half of Alpha never sees Beta and has a normal 24-hour day cycle.
In this schema, Beta and Alpha rotate around their common barycenter in 1/364th of a 365-day year. So each year Beta "falls behind" by 24 hours, and more importantly, in six months it has fallen behind by 12 hours, and is now on the opposite side of Alpha. From the point of view of Alpha City, Beta moves in the sky by about one degree every day. When it is high in the sky at noon (right side), Alpha City is in totality and has 24 hours of night - so, more like one or two months of night. Then they have several months of penumbra. Finally Beta sets, and for four-five months you needn't worry and enjoy a normal circadian rhythm (while Counter-Alpha City on the other side of Alpha is in the dark); finally Beta is low on the horizon in the morning, prolonging the night with the penumbra of the oncoming eclipse.
Having Beta counter-rotate, or being in a 3:2 or 1:2 resonance instead of a 1:1 lock, or in a different orbit, gives more complex setups with an alternance of "long" and "short" days.