What would the problems with / consequences of a torus shaped planet be?

Question

Take a torus planet:

It has the structure of a normal planet (ours):

The diagram is not to scale. Assume similar proportions of Crust:Mantle:Cores as the Earth. Is has a similar volume (close to 1.1 x 10¹² km³).

It is simply there, as of now. It's orbiting a star, similar to Sol, our sun in the habitable zone.

What would the difficulties be with this for:

• The planet itself -

  • Would it stay intact?

  • Would the gravity it was exerting on itself cause it to form a sphere?

  • Would oceans be able to form - would they simply evaporate?

  • Would there be a magnetic field? What would be the problems with / without this field?

• A colonising / evolving race -

  • Would there be varying gravitational fields that would cause problems?

  • What about day / night cycles - presumably they would be strange?

  • And the above - oceans forming and the magnetic field.

Also, would the be strange effects in the center of the torus due to the gravity - I'm imagining something like this:

Image, Om/One speaker

Where you get objects floating because of gravity?

Other images: Tim, 2014

Answer 1

There are a lot of cool effects - and some problems.

Formation

Planets form after a long, drawn-out process that starts with grains of dust colliding and forming planetesimals. These then become protoplanets, which can be kilometers across. More collisions result in small, rocky planets, some of which become terrestrial planets; others form the cores of the gas giants. The whole process is such that it becomes extremely likely that a planet will form into a sphere (or, more properly, an oblate spheroid). It's true that you could have bits of dust and rock stick together into some sort or toroidal shape, but they would most likely collapse.

Incidentally, the IAU's definition of a planet requires that the body has rounded itself. This is primarily a terminology issue, but it stems from the fact that massive bodies tend to fall in on themselves into roughly spherical shapes. The IAU defines a planet as (emphasis mine) an object that

1. is in orbit around the Sun,
2. has sufficient mass to assume hydrostatic equilibrium (a nearly round shape), and
3. has "cleared the neighbourhood" around its orbit.

Again, this is mainly a terminology point, but it arises from the idea that massive objects become spherical under the influence of their gravitational pull.

Now I'll address the specific points in your question.

Would it stay intact?

I would think so, if it's massive enough - at least, in the sense that it wouldn't fly off into space. However, I doubt it could maintain the toroidal shape for long - it would most likely collapse into a sphere.

Would the gravity it was exerting on itself cause it to form a sphere?

Yes.

Would oceans be able to form - would they simply evaporate?

Well, if it's massive enough, it should be able to hold onto matter. Evaporation of oceans is going to be influenced by the distance to the star (which appears to be the same as that of Earth) and whether or not there is an atmosphere. I should think that it would be able to hold onto an atmosphere, so you should be fine.

Would there be a magnetic field? What would be the problems with / without this field?

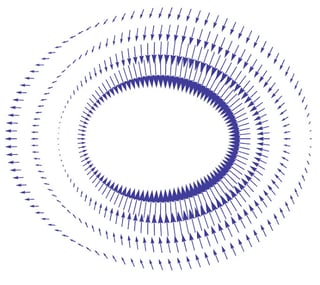
Here we run into a problem. The Earth's magnetic field comes from the motion of fluids in the core. This idea is known as the dynamo theory. However, as Wikipedia states,

A requirement for the induction of field is a rotating fluid. Rotation in the outer core is supplied by the Coriolis effect caused by the rotation of the Earth. The Coriolis force tends to organize fluid motions and electric currents into columns (also see Taylor columns) aligned with the rotation axis.

As celtschk pointed out, the Coriolis force still exists, because the object is rotating. That means that there will still be fluid motion through the core, and there should still be a magnetic field. I don't know quite what properties the field would have because it's hard to figure out where the poles would be. There might be a 'ring-shaped' (for lack of a better word) central section from which the field emanates - but like I said, I'm not sure. At any rate, it probably wouldn't come from near the axis of rotation of the planet, like Earth's field does.

Would there be varying gravitational fields that would cause problems?

Let me try to do a back-of-the-envelope calculation.

Putting some facts out there we'll need:

• The force between two objects due to gravity is $$F=G\frac{Mm}{r^2}$$
• The torus has a radius $R$ between the center point and the circle going through the circle going through the center of each cross section; each cross-section has a radius $r$.
• The mass of the torus is $m$, and the density is $\rho$.
• The volume of a torus is $$V=(\pi r^2)(2 \pi R)=2 \pi ^2 r^2 R$$

A given section of the torus defined by an angle $\theta$ (in radians) from the axis in the center will have a mass of $$m_{\theta}=\left( \frac{\theta}{2 \pi} \right)m$$ Now, the center of mass of a second slice separated from the first by angle $\theta$ will be a distance $$D_{\text{inner}}=\sqrt{ \left( \left( R - R \cos \left( \frac{\theta}{2} \right) + r \right) ^2 + \left( R \sin \frac{\theta}{2} \right) \right)^2}$$ away from an object on the inner part of the torus, and a distance of roughly the same to a point on the outer part of the torus. (I can't describe the exact technique yet, but it's very simple - I'll show it later if I can). This means that the force on the particle (with mass $\mu$) from the center of mass of the other piece is $$dF=G\frac{\left( \frac{1}{2 \pi} \right)m \mu}{\left( R - R \cos \left( \frac{\theta}{2} \right) + r \right) ^2 + \left( R \sin \left( \frac{\theta}{2} \right) \right)^2}d\theta$$ and the total force of gravity on the particle will be $$F=2 \int_0^{\pi} G\frac{\left( \frac{1}{2 \pi} \right)m \mu}{\left( R - R \cos \left( \frac{\theta}{2} \right) + r \right) ^2 + \left( R \sin \left( \frac{\theta}{2} \right) \right)^2} d \theta$$ Substituting in the mass of the Earth for $m$, the radius of the Earth for $r$, and $R/4$ for $r$, I get an acceleration of $51.3\text{ m/s}^2$. However, the torus needs to have a much lower mass in order to stay in a toroidal shape, so the surface acceleration due to gravity could actually be much more manageable.

What about day / night cycles - presumably they would be strange?

It appears that most places would have normal day/night cycles. Some places on the inner edge may never see day if $R$ is small, but if $R$ is big - and I think this is likely - things should be relatively normal. Although the sky would consist of the other side of the planet.

Answer 2

Some time ago, I calculated a toroidal planet numerically. In this answer, I give the parameters I obtained for reference to anybody who would be interested in this question.

Method

Rock and metals may seem solid, but on the scale of planets and in the pressures in their interiors, they are in a very good approximation liquid. To have a stable toroidal planet, its surface must have equal potential energy. In other words, when moving on the surface of the torus, we must not be doing any work. If this important condition is not met, the planet is unstable.

I represented the planet by putting many massive points, for which both potential and gravitation force can be calculated, in many slices that form a torus. Each slice had three circles of massive points. Several tests showed that putting more circles of massive points or more slices does not change the result too much.

Representation of the torus by massive points. The torus is in fact elliptical.

Then, I included a centrifugal force potential and I was looking for surface of constant potential, which would enclose the massive points and which could represent the surface of the planet. At the surface, I calculated the force field.

Gravitational force field on the torus surface. Inner side of the torus is right, outer side is left.

It may be counter-intuitive that the potential energy is equal on the whole surface, but the gravity strength is changing. Equal potential energy only means that the force always points into the surface and walking around does not require work. But the gravitational force will change. It is strongest inside the torus where the centrifugal and the gravitational forces add up. It is weakest at the outside, where they subtract.

Results

The most important result is that if you precisely balance the gravitational and the centrifugal force, the toroidal planet can indeed be stable. The planet must rotate very quickly, otherwise it will collapse to a sphere. In this case once in 2.65 hours. Other parameters valid for my planet are listed below, but there can probably be many others that work as well.

• Density: $5500\;\mathrm{kg/m^3}$
• Mass: 6.6 Earth masses
• Volume: 6.6 Earth volumes
• Outer radius: 19 134 km
• Inner radius: 6378 km
• Major axis: 6378 km
• Minor axis: 4464 km
• Outer g. acceleration: $3.64\;\mathrm{m/s^2}$
• Top/bottom g. acceleration: $7.36\;\mathrm{m/s^2}$
• Inner g. acceleration: $9.78\;\mathrm{m/s^2}$
• Length of day: 2.65 hours

Answer 3

This gives a good treatment of the subject at hand, with a surface gravity map provided as well.

Regarding the magnetic fields, if the (presumably metallic/liquid) core was in motion or somehow had a ring current induced within, it would produce a magnetic field which will direct particles to pass through the center of the torus. The image shows the ring current of the Earth's magnetosphere, which result in the aurorae. A similar magnetic field in a torus planet would not result in any aurorae, since they pass through the center.

But most certainly, the planet will NOT hold up under gravity without the benefit of exotic materials with tensile and compression strengths multiple orders of magnitude higher than anything known to engineers.

Answer 4

The consequence would be that (if naturally formed) its own gravity would collapse it into a sphere.

To persist, it would have to be engineered (artificially build) like Larry Niven’s Ringworld

In either case you will have to deal with the lack of magnetic field protecting it from cosmic rays and/or the sun (if either one has any).

A torus world (if you can make it rigid enough to persist) would have weird gravity, depending if you are on the "inner" side of the torus (weaker gravity) or the "outer" (stronger). And it would have to be improbably rigid to persist. Outside of the realm of physically feasibility. Only magic could do it for you.

Edit: After reading link in interesting @March Ho answer (go read it I'll wait) it seems that for some very narrow values of rotation speed, such torus might be stable (if not disturbed by tidal forces from own Sun or gravity of other planets).

Fascinating differences in gravity (with consequences to atmospheric flows), geostationary orbit just 2000 km up, North Pole Circle, differences in seasons between inner and outer equators, or even 4 cold and 4 warm seasons in a year, and bonus: moon trajectories, like one bobbing up and down through the hole, or forming a vase inside.

Unlikely, but intriguing if created.

Answer 5

That's a fun problem to think about. I am curious to know which of the planet's possible axes of rotation would be perpendicular to the tangent of the arc of its orbit around the sun.

as others have mentioned, soil and water would tend to accumulate in the center of the ring (where the smaller circumference will put any two points at the inner edge of a cross section closer to each other than particles on the outside edge of those two cross sections and the angle of attraction will be pulling the particles away from the surface of the planet and towards each other and by extension, the center of the torus, reducing the effect of "downward" gravitational force on friction between particles and enhancing slippage and accumulation in the center of the ring at a geometrically increasing pace). This would be despite rotational speed, as long as the planet had sufficient mass to exert a strong enough gravitational effect to prevent particles on the outer edge from leaving orbit. That would give you an interesting starting point for some very unusual tidal action - especially if you take a moon into account.

I think you would also see jets of charged coronal mass being sucked down into then ejected out of the center of the "top" and "bottom" of the torus if the torus was pointed "edge on" to the sun/ solar winds OR some possible accumulation of said particles on the far/trailing side of the torus if the torus is not oriented perfectly "edge on" to the sun/ solar winds. this could lead to a spectacular culumnar aurora centrus visible as a pale cylindrer in the first case. In the second case, visible only from the dark side of the ring, the aurora would be more like what we see on our own planet, but more turbulent with red near the center, green surrounding that and blue and purple mixing in at the edges. the view from the inside of the ring in the first case would depend on the size of your planet and the thickness of the atmosphere, but assuming the planet were large enough, the aurora could be viewed from the umbra of the sun-side half of the ring when looking straight "up" toward the center of the sun-side half of the torus from the innermost part of the half of the torus farthest from the sun.